filmov
tv
Laplace transform: Why do we only care about Re(s)
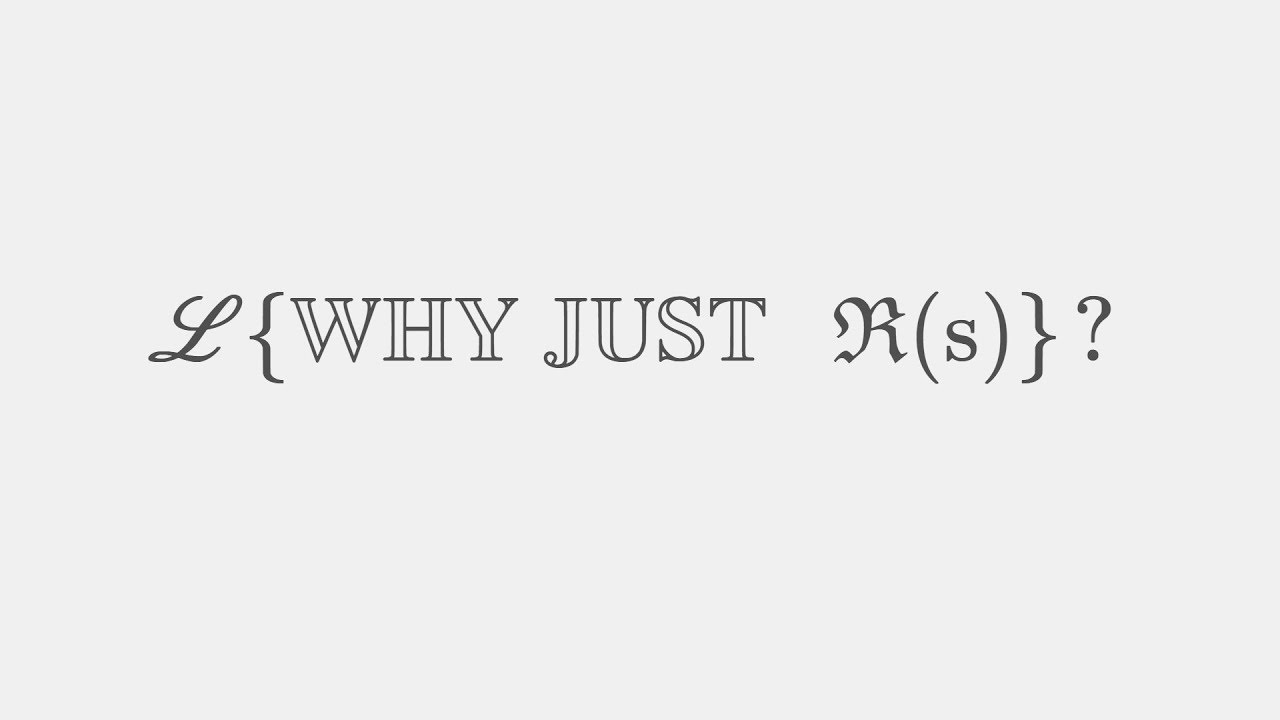
Показать описание
When encountering laplace transformations you are bound to work with certain conditions to make those functions converge. So why do we only care about the real part of this complex valued s?
Help me create more and better content! =)
Visit my website! =)
What does the Laplace Transform really tell us? A visual explanation (plus applications)
Why the Laplace Transform?
Laplace Transform Explained and Visualized Intuitively
The intuition behind Fourier and Laplace transforms I was never taught in school
Laplace transform: Why do we only care about Re(s)
What is Laplace Transform? Why Laplace Transform is used in Circuit Analysis?
Everything you need to know about Laplace transforms
When do you need the Laplace Transform?
What are Laplace Transforms?
Intro to the Laplace Transform & Three Examples
Laplace Transform Equation Explained
Why do we use the Fourier Transform?
Review of Laplace Transform (Part 1)
The Laplace Transform and the Important Role it Plays
Introduction to Laplace Transform- Why do we use Laplace Transform? What s time and frequency domain
The Laplace Transform: A Generalized Fourier Transform
This is why you're learning differential equations
Laplace Transform of t^4 #shorts
How to Find the Inverse Laplace Transform of 1/s^4 | #shorts
Laplace Transform: First Order Equation
The Laplace Transform - The Basic Idea of How We Use It
Using Laplace Transforms to solve Differential Equations ***full example***
What is Laplace Transform | Where the Laplace Transform comes from | Derivation of Laplace Transform
The Laplace Transform | Definition
Комментарии
 0:20:25
0:20:25
 0:02:59
0:02:59
 0:19:55
0:19:55
 0:18:00
0:18:00
 0:04:33
0:04:33
 0:13:36
0:13:36
 0:07:42
0:07:42
 0:07:11
0:07:11
 0:02:24
0:02:24
 0:12:05
0:12:05
 0:04:42
0:04:42
 0:00:59
0:00:59
 0:08:15
0:08:15
 0:10:13
0:10:13
 0:09:44
0:09:44
 0:16:28
0:16:28
 0:18:36
0:18:36
 0:00:15
0:00:15
 0:00:19
0:00:19
 0:22:38
0:22:38
 0:01:33
0:01:33
 0:09:30
0:09:30
 0:19:28
0:19:28
 0:15:01
0:15:01