Proof: Triangle Midsegment Theorem | Geometry, Proofs

Показать описание

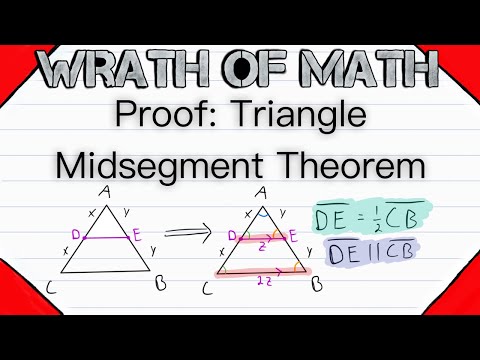
The triangle midsegment theorem states that in a triangle, the segment joining the midpoints of any two sides of a triangle is half the length of the third side and parallel to the third side. This is a very neat result and not too tricky to prove. In today’s geometry lesson, we go over a proof of the triangle midsegment theorem!

To prove the triangle midsegment theorem, we use the SAS (side angle side) triangle similarity postulate, which tells us if two pairs of sides of two triangles are proportional (in the same ratio), and the included angles of these sides are congruent as well, then the triangles are similar. Remember that corresponding angles of similar triangles are proportional, and corresponding angles of similar triangles are congruent!

We also use the converse of the corresponding angles theorem in this proof. The converse of the corresponding angles theorem states that if two lines are cut by a transversal, and a pair of the resulting corresponding angles are congruent, then the lines are parallel.

I hope you find this video helpful, and be sure to ask any questions down in the comments!

+WRATH OF MATH+

Follow Wrath of Math on...

Рекомендации по теме

Комментарии

Thank you so much! This made virtual geometry without hardly any instruction during the pandemic so much easier! The slides my son received, had no explanations. This was simple!

blueheronacupuncturellc

If I could prove it on my own I wouldn't need to watch this video, but thank you for your optimism

BrandonBDN

If the all angles is given and the big tringle base is given than how to calculate the small triangle base and altitude of both.

Plz give the answer.😇

muhammadyaseen

thanks! this helped me alot in my homework!✨

zahraasibaii

Our book has triangle similarity after this chapter. I would approach this by using dilation. Dilate DE with center of dilatiomn at A scale factor by 2.

mr.osunasmathematicschanne

It is about time, though, that someone proved all of Euclid's theorems and propositions using only cartesian/vector geometry methods. Euclidean geometry has been around for 2000 years, cartesian geometry proofs are ~300 or ~400 years old.

maxpercer

yeah im still so lossed. can someone explain this to me like im 4 yrs old?

jacksonward

Proof: Triangle Midsegment Theorem | Geometry, Proofs

Proof: Triangle Midsegment Theorem | Geometry, Proofs

Midsegment Theorem Triangles (Geometry)

The Triangle Midsegment Theorem

Triangle Midsegment Theorem Proof. Geometry video.

How to PROVE the Triangle Midsegment Theorem

Use triangle similarity to prove the Triangle Midsegment Theorem

Triangle Midsegment Theorem proof support

Vector Calculus 6: The Triangle Midsegment Theorem

Triangle Midsegment Theorem

Proving the Triangle Midsegments Theorem

Triangle Midsegment Theorem - All properties in less than 3 minutes.

Triangle Midsegment Theorem

Midsegment Of Triangle And Proving Its Theorem

6.4 The Triangle Midsegment Theorem

Triangle Midsegment Theorem Proof

Proving the Triangle Midsegment Theorem - Geometry

Proving the Triangle Midsegment Theorem in Coordinate Plane

Triangle Midsegment Theorem

#8 Converse Triangle midsegment (midpoint) Theorem proof

Midsegment Theorem and Coordinate Proof, 01

6.4 The Triangle Midsegment Theorem

Proving Midsegment Theorem

What is the Triangle Midsegment Theorem? | Geometry, Triangles

Triangle Midsegment Theorem