Arithmetic Mean, Geometric Mean, Weighted Mean, Harmonic Mean, Root Mean Square Formula - Statistics

This college statistics video provides a basic introduction into the arithmetic mean, geometric mean, weighted mean, harmonic mean, and root mean square formulas.

Introduction to Statistics:

Descriptive Vs Inferential Statistics:

Qualitative and Quantitative Data:

Statistic Vs Parameter:

Scales of Measurement:

__________________________________
Mean, Median, Mode, & Range:

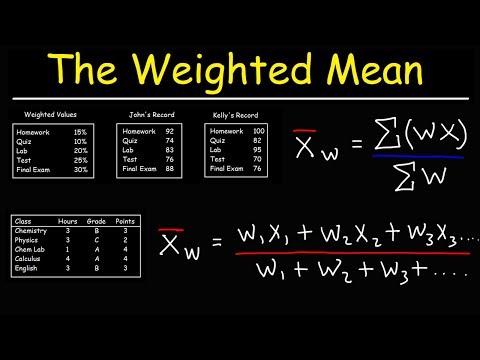
Weighted Mean & Averages:

Find Missing Value Given The Mean:

Excel - Mean, Median, Mode, & Range:

Arithmetic, Geometric, & Harmonic Mean:

___________________________________
Simple Frequency Tables:

Relative Frequency Distribution Table:

Cumulative Relative Frequency Table:

Dot Plots and Frequency Tables:

Stem and Leaf Plots:

____________________________________
Final Exams and Video Playlists:

Sequences - Free Formula Sheet:

[nguyendang]

Arithmetic Mean 0:00
Geometric Mean 5:05
Weighted Mean 11:14
Harmonic Mean 18:01
Root Mean Square 42:44
Thank you so much

[btechbinharuntech]

Imagine this guy is still making educational videos till date

[farheenkauser]

best video i came across related to these topics !!!! grateful to have a teacher like you in this platform .

[trelligan]

While I 'learned' all of these many years ago, if asked an hour ago I would have gotten confused and probably wrong. Tying the different averages to an application—middle number in a sequence—makes it much clearer and easier to remember. Thanks! #FeedTheAlgorithm

[assaultfighterhd]

can you do a face reveal, you have helped me since grade 8 till now in grade 12. Thank you for all the math and science lessons that you explained better then any teacher I have had.

[successthruknowledge]

So, really, in the same way that you can find the average of an arithmetic mean that is a series of four numbers using a1 and a4; then you should be able to do the same thing for a geometric mean; yes? Oh nice; I was never sure about 9:23 . I like especially the weighted mean chemistry example. I never heard of a harmonic mean. I liked how he emphasized the difference between the average speed and the arithmetic mean speed and how that relates to how time traveled is more influential with respect to speed. Cool pattern with the mean rankings!

[alex_ramjiawan]

The inconsistency with the units in the harmonic mean example of the car is so infuriating. Other than that, this was a good way to spend an hour when getting bored.

[valterfagerheim]

Correction: The average speed of a car traveling 400 miles at the speed of 80 mph one way and 50 mph the other way should be 61.54 mph (miles per hour) and not 61.54 m/s (meters per sec.)

[SUN_Star]

Sir, can you please make a video on Leibniz rule

[meissying]

tysmmm istg ur videos help me a learned a lot of new concepts 🥰

[shienlymaecerilo-c.franci]

can you teach us how to find the arithmetic sequence if the arithmetic means are given

[safa]

Proud of you 👏🏻 the best explanation ❤

[soundrogue]

10:31 warning, it's rounded up so if you don't end up with the same answer on the calculator that is why.

[Prof.Garcia]

I get he same results, using percentage or decimals the way you thaught in a different video, i just realized you could calculate use the percetages as whole numbers intead of decimals and percentages in wich case it would be

[ashiskumer]

Bro start making vedio for ielts student❤

[nyarangalucy]

Thank for all the support God bless you

[abdimalikmohamud]

It could hve been better if you could hve shown us how you were putting those digits in the calculator

[imdelululu]

Can u do a video on parallelogram vector?

[void]

This explanation lacks motivation and extensibility, and can cause some misunderstanding. It would be hard for a student to extend these ideas to any size data set, as they don't see the motivation for the mean (given a certain operation applied to a list of numbers, find a single number which, when the same operation is applied, yields the same result), and your example sequence are special cases that might give the impression of added general properties that are not true. You also pull out the weighted arithmetic average as a separate operation, which might give students the impression that you can't weight other average types.

I had to go over some of these points with several students after they watched this.

[PreciousOhiomah]

So lemme show you what I mean 😲😯 18:43