filmov
tv
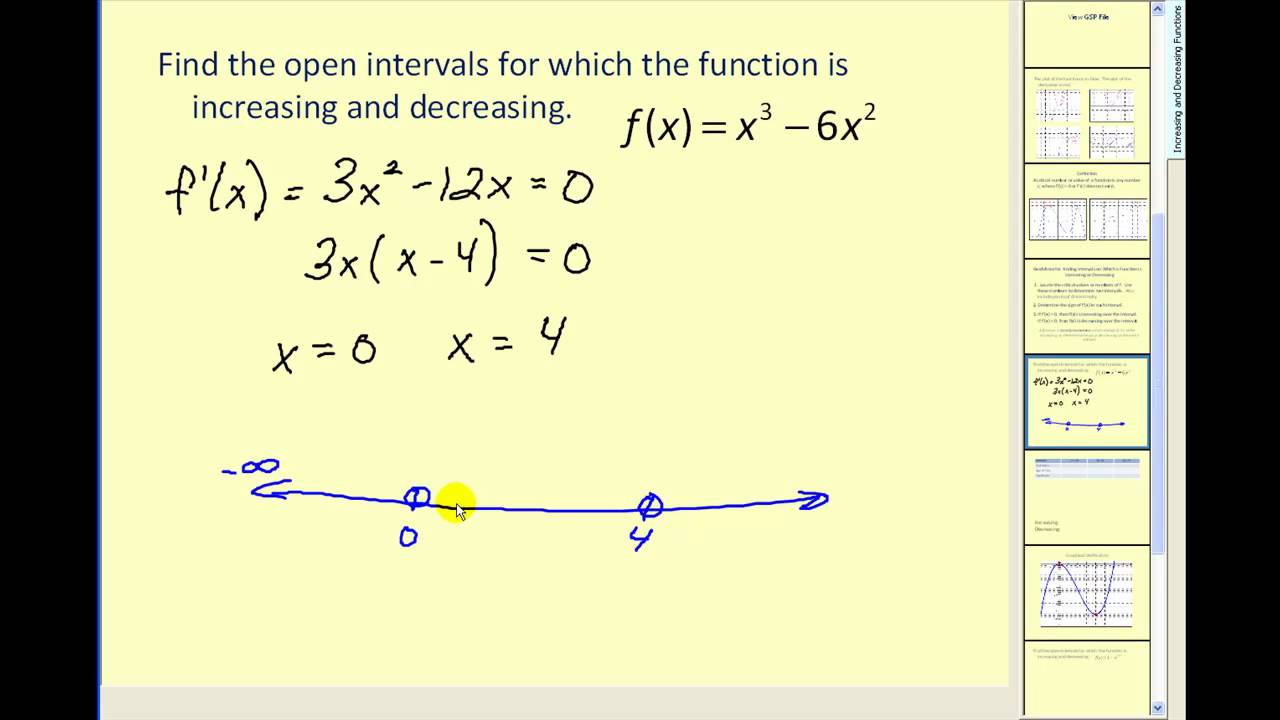
Determining where a function is increasing and decreasing using the first deriviative
Показать описание
Determine if the equation represents a function
Determine If a Function is Odd Even or Neither
How to determine the intervals that a function is increasing decreasing or constant
Determine whether a function is 1 to 1 (KristaKingMath)
How to Determine If a Function is Odd, Even or Neither
Determining Whether an Equation Represents a Function
Master Determine if the equation is a function or not
Determine the discontinuity of the function
Transformation of a Tangent Function: Determine the Period, Phase Shift, and Asymptotes
8.2 determine if it's a rational function
Defining a Function in Python | Python Tutorial for Beginners
Functions: Determine if the graph is a function or not.
How to determine if a function is continuous and differentiable
How to Determine if a Function is One-to-One Algebraically
Determining if a function is invertible | Mathematics III | High School Math | Khan Academy
Determining where a function is increasing and decreasing using the first deriviative
Determine if a Relation is a Function
Master Determining if a function is a polynomial or not
Determining if a Function is a Polynomial
Master how to determine if a function is linear or not
How to determine if a function is even odd or neither
How to determine if an ordered pair is a function or not
Determine if an Equation Represents a Function (Basic with Definition Only)
Ex: Determine if a Table of Values Represents a Function
Комментарии
 0:02:14
0:02:14
 0:02:21
0:02:21
 0:02:56
0:02:56
 0:04:23
0:04:23
 0:02:11
0:02:11
 0:01:57
0:01:57
 0:12:58
0:12:58
 0:01:05
0:01:05
 0:05:10
0:05:10
 0:01:47
0:01:47
 0:01:52
0:01:52
 0:03:06
0:03:06
 0:02:21
0:02:21
 0:13:56
0:13:56
 0:04:03
0:04:03
 0:10:05
0:10:05
 0:06:19
0:06:19
 0:04:38
0:04:38
 0:03:30
0:03:30
 0:04:55
0:04:55
 0:07:35
0:07:35
 0:02:59
0:02:59
 0:05:59
0:05:59
 0:05:16
0:05:16