filmov
tv
The Selberg Sieve and Large Sieve (Lecture 1) by Satadal Ganguly

Показать описание
Program
Workshop on Additive Combinatorics
ORGANIZERS: S. D. Adhikari and D. S. Ramana
DATE: 24 February 2020 to 06 March 2020
VENUE: Madhava Lecture Hall, ICTS Bangalore
Additive combinatorics is an active branch of mathematics that interfaces with combinatorics, number theory, ergodic theory, harmonic analysis and geometry over finite fields. This workshop has been designed to introduce the participants to different facets of additive combinatorics such as the Ramsey theory, inverse problems, polynomial methods, sum-free sets and more. It will also touch upon topics such as the work of B. Green and T. Tao, on long arithmetic progressions in the primes and Sieve methods from number theory.
This workshop will appeal to young researchers interested in number theory and combinatorics. It will also be beneficial to researchers working in different areas of mathematics to get an overview of the many different topics in the field of additive combinatorics.
0:00:00 The Selberg Sieve and Large Sieve
0:00:33 Plan
0:02:12 Some Linear Algebra
0:10:04 Proposition
0:11:40 Proof
0:14:36 Q: How small can Delta be?
0:22:11 Proposition (Duality Principle)
0:24:33 Remark
0:25:27 Proof
0:32:33 Corollary
0:36:59 Duality Principle
0:40:31 Theorem 0:
0:41:40 Proof
0:51:09 Remark
0:58:52 General Philosophy
0:59:36 Example - Bombier-Vinogrador theorem
Workshop on Additive Combinatorics
ORGANIZERS: S. D. Adhikari and D. S. Ramana
DATE: 24 February 2020 to 06 March 2020
VENUE: Madhava Lecture Hall, ICTS Bangalore
Additive combinatorics is an active branch of mathematics that interfaces with combinatorics, number theory, ergodic theory, harmonic analysis and geometry over finite fields. This workshop has been designed to introduce the participants to different facets of additive combinatorics such as the Ramsey theory, inverse problems, polynomial methods, sum-free sets and more. It will also touch upon topics such as the work of B. Green and T. Tao, on long arithmetic progressions in the primes and Sieve methods from number theory.
This workshop will appeal to young researchers interested in number theory and combinatorics. It will also be beneficial to researchers working in different areas of mathematics to get an overview of the many different topics in the field of additive combinatorics.
0:00:00 The Selberg Sieve and Large Sieve
0:00:33 Plan
0:02:12 Some Linear Algebra
0:10:04 Proposition
0:11:40 Proof
0:14:36 Q: How small can Delta be?
0:22:11 Proposition (Duality Principle)
0:24:33 Remark
0:25:27 Proof
0:32:33 Corollary
0:36:59 Duality Principle
0:40:31 Theorem 0:
0:41:40 Proof
0:51:09 Remark
0:58:52 General Philosophy
0:59:36 Example - Bombier-Vinogrador theorem
 1:01:31
1:01:31
 0:52:10
0:52:10
 0:56:34
0:56:34
 0:58:40
0:58:40
 1:12:21
1:12:21
 0:02:39
0:02:39
 1:00:05
1:00:05
 1:07:25
1:07:25
 0:18:00
0:18:00
 0:58:18
0:58:18
 0:38:24
0:38:24
 1:04:31
1:04:31
 0:57:27
0:57:27
 0:55:51
0:55:51
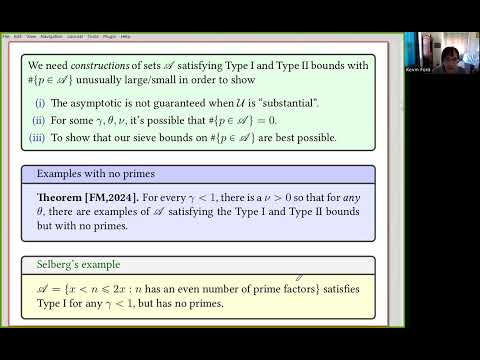 0:51:12
0:51:12
 0:59:24
0:59:24
 0:56:20
0:56:20
 0:42:08
0:42:08
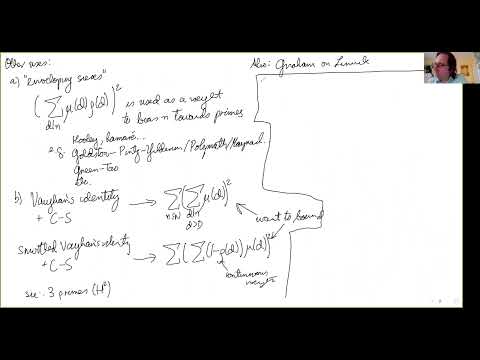 0:59:59
0:59:59
 0:38:29
0:38:29
 1:02:29
1:02:29
 0:08:00
0:08:00
 0:04:26
0:04:26
 1:00:09
1:00:09