How Physics Includes Air Resistance in Calculations | Real Physics

Ignore Air Resistance? I don't think so...

Hey everyone, I'm back with another video! This time, we're looking at how air resistance (drag) is modelled in physics equations. Quite often, in order to make the math easier, we are told to ignore air resistance, but in order to create a more realistic model, we need to account for it.

Using classical physics, we will be studying the motion of a pendulum. We will first look at all the parameters we use to describe a simple pendulum, and then learn how to generate an equation of motion for a pendulum in a vacuum. Then, we will see how to add in all the air resistance (drag) and how this affects the equation of motion. We will also be looking at how to solve these equations of motion, and what impact this has on the motion of the pendulum itself.

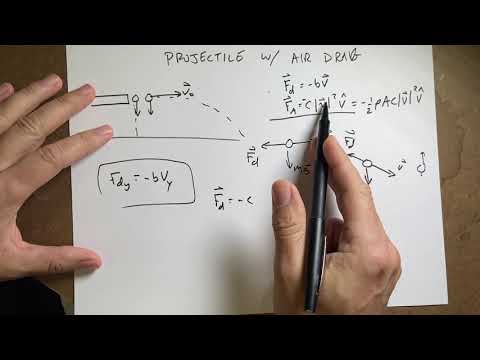
We use classical physics principles (such as Newton's Second Law of Motion) to generate the equation of motion. We decide to model air resistance very simply, by assuming that drag force generated is directly proportional to the (angular) speed of the pendulum at any point in time. We also see that a pendulum's motion is very easily described in terms of the angle (we called it theta) between its vertical position and any other position at a given point in time. We look at how this angle changes, as well as the angular velocity / angular speed, and the angular acceleration of the pendulum. It's worth noting that we use the Small Angle Approximation in order to easily solve the equations of motion, because without it we would need some computational methods. This means that we will only be able to consider the motion of the pendulum until about 60 degrees or 1 radian away from the vertical.

As it turns out, a pendulum in a vacuum (i.e. no air resistance) undergoes sinusoidal oscillation forever. In other words, it simply pings back and forth and back and forth. However, adding air resistance actually causes this sinusoidal oscillation to decay exponentially. Mathematically, we see this as multiplying our sine oscillation by a decaying exponential term, known as the "exponential envelope".

It's also worth noting that there are a few other solutions to the equation of motion that accounts for air resistance. This all depends on how strong the drag force is (depending on the medium the pendulum moves through, we called this factor "gamma"). Also, I've taken a lot of mathematical shortcuts and waved my hands many times in this video! My aim is to provide an intuitive understanding of how we do the mathematics and what comes from it, rather than a detailed theoretical dive into the topic.

Here are some useful resources for the bits that I mentioned in the video:

Here are some timestamps:
0:00 - Air Resistance!
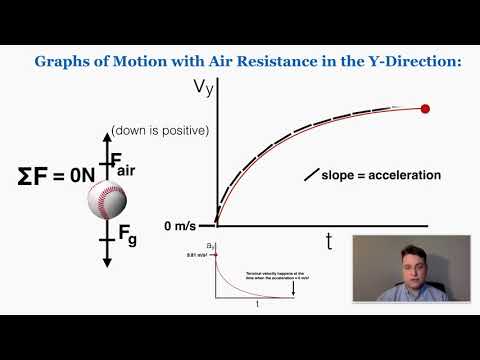
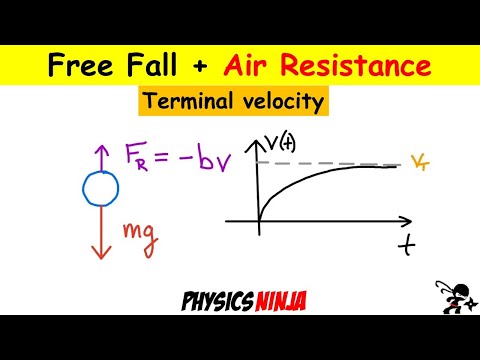
0:48 - Terminal Velocity (for which air resistance is essential!)
2:37 - How We Will Model Air Resistance Using Math
3:02 - Setting Up The Equations for a Pendulum Using the Angle Theta
3:38 - Angular Velocity and Angular Acceleration Explained Using Linear Velocity and Acceleration
5:36 - Newton's Second Law of Motion (and a Bad, Hand-Wavy Use of It!)
6:14 - Finding the Components of the Force on the Pendulum
6:54 - Setting Up an Equation of Motion (Ignoring Air Resistance For Now)
7:28 - The Small Angle Approximation
8:16 - The Solution (i.e. How a Pendulum Behaves Without Air Resistance) - Sinusoidal Oscillation
8:36 - Air Resistance Has Entered The Chat
8:48 - Modifying the Equation of Motion to Include Air Resistance
9:24 - Pendulum in Air vs Honey: The Constant of Proportionality
10:15 - The New Solution!
10:45 - The Pendulum No Longer Oscillates Forever - The Effect of Drag

Thanks so much for watching, please check out my links here:
Instagram - parthvlogs

[ParthGChannel]

Hi friends, thanks so much for watching this video! A kind viewer has pointed out that I have got my signs mixed up a bit! The drag force, which should indeed be acting in the opposite direction to the sine component of the gravitational force, already has an in-built negative sign due to the angular velocity (at least at the position the pendulum is in in our diagram). Therefore, the third term in the equation at 10:07 should actually be positive (unless we were to say that gamma is negative). Whoops :D

Thanks Murillo for pointing out my error!

[rodericksibelius]

I am a retired US Navy veteran Aircraft Structures, Airframes, Hydraulics, Pneumatics Flight Controls Systems Technician and I have always wanted to know the advanced physics and math those Mechanical and Aeronautical Engineers know.



I have been looking for an answer, for this simple pendulum problem and I have hanged string bob pendulums in my garage at STP 'Standard Temperature and Pressure' and have watched many excellent lectures here on YouTube, my mathematical physics or math can only grasp college algebra, basic physics and trigonometry with some knowledge of calculus one. Finally I have found your VIDEO gave me the insight in the way you have explained this complex problem. My goal was to know how to derive the real world mathematical physics of THE TIME 't', when the pendulum swinging has practically STOPPED, in air as a Mechanical Engineering problem. Thank You SIR.

[ogremighty]

Could you please explain "Natural Frequency" of objects to us? Thank you!

[przemekreszka]

I would love to see a video about solving basic differential equations(not numerically) or building uderstanding of a certain process by simply looking at them.

[ahsanrizvi]

For Those who don't want to go deep but also want to know the "other related" quantity, it's torque. Which is kinda angular force.

[_khlluxv]

You explain so well and I, as many others, don't feel anxious listening and learning, a BRILLIANT teacher, thank you very very much!

[shubhagkamath]

Thanks Parth for this amazing content, I always wanted this as a high schooler but didn't find any good video.

Thank you

[dhanashrikulkarni]

This is the perfect youtuber for me...
Mrbeast, Pewdiepie etc.
Are just show offs. He is the real person
Thanks bro..

[sunithasomalingam]

Great Brother...

May I know what was your thesis?

[sreedathpr]

Love your videos very much Parth. Can you tell what was your thesis about?

[avnishsaravanan]

Hello Parth! Your videos encourage me to learn something new everyday. Suggestion: Please make a video on string theory.

[sphakamisozondi]

01:25, my dog in essence, tastes drag force everytime i drive.

[dm]

Great to see vids like this up so thank you, but I'm unsure of some of your assumptions. You state that drag force is proportional to velocity, but I believe that is damping force that is proportional to the speed something moves, however, air resistance/drag is proportional to velocity^2. What you're including in your model is frictional damping (proportional to velocity, or in this case angular velocity), which would give a different differential equation and solution at the end if this difference was taken into account.

[ananyashah]

Hey man .I love your explaintion .smoth explaintion .I want see your vedio but now I can't because my jee exam is after months .but after exam I definitely going to whatch lots of your vedio .my emence love for physics ❤️.

[MaximQuantum]

Damnnn, you explained it so gently, I was surprised I could follow to the end with ease. 😁

[georgepp]

Simple is always good. Things should never be told complicated. Thank you.

[edwardjcoad]

Good job...a video with maths and physics. Might start watching again as some earlier videos were good but not for the physics buff.

[wayneyadams]

4:00 The accepted unit for angular velocity (speed) is radians per sec, rad/s, not degrees per second, where pi radians are equal to 180 degrees (1 rad = 57.3 degrees). The reason for using radians instead of degrees is that it allows us to relate angular quantities to linear quantities by multiplying by the radius. displacement = radius x angular displacement; velocity = radius x angular velocity; acceleration = radius x angular acceleration, and so on.

[MatheMagiX]

I've been waiting for a video like that for a long time. Thank you yt suggestions! But I need to know one more thing: IS AIR RESISTANCE ACTUALLY DIRECTLY PROPORTIONAL TO THE SPEED OF THE PENDULUM IN A LINEAR MANNER?

[budjy]

Second year physics undergrad here, definitely a good refresher!