filmov
tv
Even and Odd Trigonometric Functions & Identities - Evaluating Sine, Cosine, & Tangent

Показать описание
This trigonometry video explains how to use even and odd trigonometric identities to evaluate sine, cosine, and tangent trig functions. This video contains plenty of examples and practice problems.
The Unit Circle:
How To Remember The Unit Circle Fast:
Reference Angles:
The Six Trigonometric Functions:
How To Solve Right Triangles:
_____________________________
Reciprocal Identities:
Quotient Identities:
Pythagorean Identities:
The Pythagorean Theorem:
Trig Functions - Periodic Properties:
________________________________
The Exact Value of Trig Functions:
Special Right Triangles - 30 60 90:
Special Patterns - Pythagorean Theorem:
Cofunction Identities:
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
Trigonometry Formula Sheet:
The Unit Circle:
How To Remember The Unit Circle Fast:
Reference Angles:
The Six Trigonometric Functions:
How To Solve Right Triangles:
_____________________________
Reciprocal Identities:
Quotient Identities:
Pythagorean Identities:
The Pythagorean Theorem:
Trig Functions - Periodic Properties:
________________________________
The Exact Value of Trig Functions:
Special Right Triangles - 30 60 90:
Special Patterns - Pythagorean Theorem:
Cofunction Identities:
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
Trigonometry Formula Sheet:
Комментарии
 0:10:51
0:10:51
 0:02:31
0:02:31
 0:04:05
0:04:05
 0:03:18
0:03:18
 0:04:57
0:04:57
 0:05:40
0:05:40
 0:06:16
0:06:16
 0:07:53
0:07:53
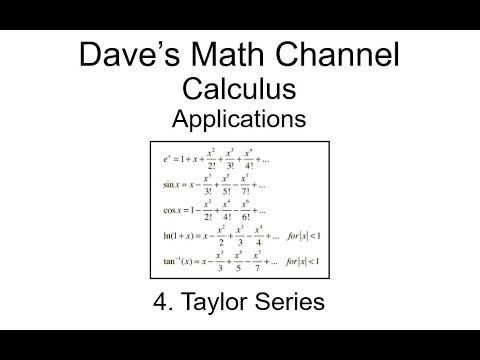 0:18:20
0:18:20
 0:01:50
0:01:50
 0:01:57
0:01:57
 0:02:22
0:02:22
 0:02:11
0:02:11
 0:17:47
0:17:47
 0:03:11
0:03:11
 0:09:18
0:09:18
 0:09:46
0:09:46
 0:06:53
0:06:53
 0:01:42
0:01:42
 0:05:57
0:05:57
 0:03:05
0:03:05
 0:06:19
0:06:19
 0:03:37
0:03:37
 0:01:06
0:01:06