Introduction to Square Roots

preview_player
Показать описание

  1. Eddie Woo
  2. math
  3. maths
  4. mathematics
Рекомендации по теме
Introduction to square 0:05:23

Introduction to square roots | Numbers and operations | 8th grade | Khan Academy

What are Square 0:04:00

What are Square Roots? | Math with Mr. J

Introduction to Square 0:07:04

Introduction to Square Roots

Introduction to Square 0:04:14

Introduction to Square Roots

Introducing Square Root 0:03:49

Introducing Square Root

Math Antics - 0:11:09

Math Antics - Exponents and Square Roots

THE SQUARE ROOT 0:05:13

THE SQUARE ROOT 🚀 What is the Square Root? 👨🏻‍🚀 Math for Kids

Why is it 0:03:03

Why is it called a SQUARE root?

Squares and square 0:11:37

Squares and square roots/ Class 8th / Introduction/ Lecture 1 / Part 1 /@arfatmathsclasses5723

What is a 0:06:38

What is a Square Root and a Perfect Square?

Understanding square roots 0:01:21

Understanding square roots | Pre-Algebra | Khan Academy

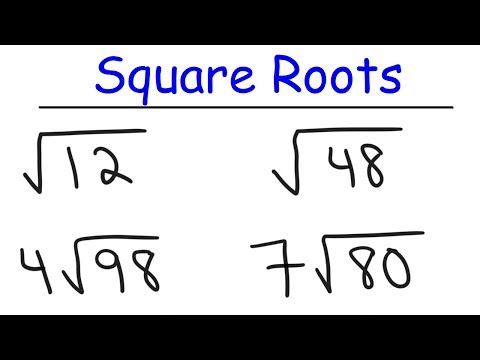
How To Simplify 0:10:46

How To Simplify Square Roots

Art of Problem 0:05:17

Art of Problem Solving: Square Root Introduction Part 1

Math Antic - 0:12:01

Math Antic - Simplifying Square Roots

Introduction to Square 0:04:37

Introduction to Square Roots With Area

Square Roots | 0:16:35

Square Roots | Intro, Perfect Squares, and Simplifying | Math with Mr. J

Square Roots and 0:05:03

Square Roots and Cube Roots | Math with Mr. J

What are Square 0:04:11

What are Square Roots? | Exponents | Best Square Root Tricks | Don't Memorise

Introduction - Squares 0:27:17

Introduction - Squares & Square Roots, Ch 5 - NCERT Class 8 Maths Solutions

Grade 8 | 0:07:03

Grade 8 | Maths | Square and square roots | Free Tutorial | CBSE | ICSE | State Board

Introduction to Square 0:07:25

Introduction to Square Roots | Number & Operations | 8th Grade

Simplifying square roots 0:03:09

Simplifying square roots | Exponents, radicals, and scientific notation | Pre-Algebra | Khan Academy

Square roots and 0:10:19

Square roots and real numbers | Pre-Algebra | Khan Academy

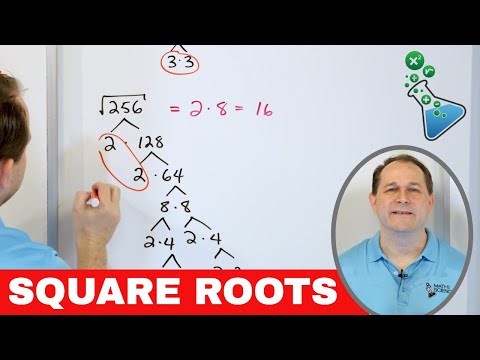
01 - Simplify 0:43:40

01 - Simplify Square Roots with Factor Trees in Algebra (Radical Expressions), Part 1

Комментарии
Автор

10 😂😂 I'm so happy you turned that silly answer into a positive learning experience. I could tell whoever said it was embarrassed right when the answer left their mouth.

GarciaLCc
Автор

Wow, you're such an amazing teacher. Basically any other teacher would have in some way punished that mistake of the student, where she said that 5² is 10, but you just handled it so well by explaining the whole class, what she did wrong so everybody could learn from it. We need more teachers like you.

zayne
Автор

You really are a greater teacher. Introducing a new concept and using the answer "10" the student gave to illustrate a common misstep is calculating powers that someone could have was flawlessly executed. I'm sure those issues and misunderstandings come up with multiplying powers by powers where the base is the same (e.g. 5^2 × 5^3) and then also raising powers by another exponent (e.g. (5^)^2). I appreciate how you don't brush off those mistakes/misunderstandings and use them as a great teaching/learning opportunity.

MrSF
Автор

Can you do a video about square and triangular numbers?

dannguyen
Автор

Hye sir why square is never negative and why we write square root+-0 e.g.square root of 25 is + or - 5?

rameshjain
Автор

We assume that (-5)^2=25 and 5^2=25 then (-5)^2=5^2.
then if we enter the square root in both sides we get : sqrt((-5)^2)=sqrt(5^2).
if sqrt(x)=x^(1/2)
then ((-5)^2)^(1/2)=(5^2)^(1/2)
(-5)^(2/2)=5^(2/2)
so -5=5.
How do you explain that?

el-mehdibenchaib