filmov
tv
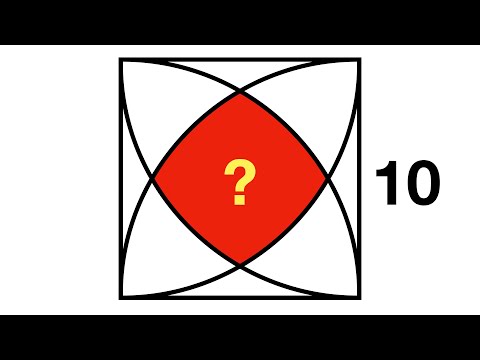
Brilliant Geometry Puzzle

Показать описание
Brilliant Geometry Puzzle
#4.2 | Geometry Puzzle | Brilliant |Chalk War | Math4Bronx | Prof. Soborno Isaac Bari
#4.1 | Geometry Puzzle | Brilliant |Chalk War | Math4Bronx | Prof. Soborno Isaac Bari
Brilliant Geometry Puzzle
Brilliant Geometry Puzzle
Brilliant puzzle for Clever Minds, draw 4 Triangle & 2 Squares using 8 Lines. 🤔🤔 #trending #puzz...
The hardest problem on the hardest test
Can You Find The Easy Answer? Learn The Brilliant Trick!
The Simplest Math Problem No One Can Solve - Collatz Conjecture
The Riddle That Seems Impossible Even If You Know The Answer
Brilliant Geometry Puzzle
The 3 Piece Dilemma - Brilliant Puzzle!
AREA OF green square Puzzle!! Best tricky puzzle only brilliant mind can solve this!! #Puzzles
Brilliant Geometry Problem
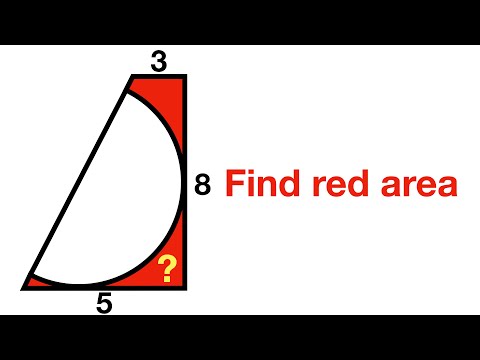
Brilliant Geometry Problem! Find the blue area.
Brilliant Geometry Puzzle
Can you solve the wizard standoff riddle? - Dan Finkel
The Korean king's magic square: a brilliant algorithm in a k-drama (plus geomagic squares)
How many Guards | Brilliant's daily Problem | 12-Oct-2021 | Geometry
brilliant geometry problem
Count The Triangle | Maths Riddle | brilliant math puzzles | Maths I Win
Can you solve this!Best Maths Puzzle!!Best Tricky Puzzle only brilliant mind can solve this! #puzzle
Brilliant PUZZLE: Make 7 equilateral triangles with 9 matchsticks || Elegant solution
Math's Beauty 😍 || Brilliant Geometry tricks #maths #mathsbeauty #circle #ytshorts
Комментарии
 0:04:54
0:04:54
 0:00:42
0:00:42
 0:02:07
0:02:07
 0:07:51
0:07:51
 0:04:54
0:04:54
 0:00:39
0:00:39
 0:11:15
0:11:15
 0:04:03
0:04:03
 0:22:09
0:22:09
 0:17:45
0:17:45
 0:03:03
0:03:03
 0:00:40
0:00:40
 0:05:52
0:05:52
 0:03:59
0:03:59
 0:04:38
0:04:38
 0:02:43
0:02:43
 0:05:26
0:05:26
 0:33:25
0:33:25
 0:06:09
0:06:09
 0:01:47
0:01:47
 0:00:49
0:00:49
 0:03:11
0:03:11
 0:02:20
0:02:20
 0:00:17
0:00:17