filmov
tv
Area of Circle | Circle inside Quarter Circle and Square problem | Advanced math problems | Geometry

Показать описание
Usually tangents are referred to tangent lines to a circle or a curve at some point. But in fact if two circles are touching each other they are called tangent circles, and they have many interesting properties. This problem is an application of tangent circles.
Any queries regarding the subject or videos are invited.
Instagram ID : problem_analysis
#maths #mathematics #circle #square #quartercircle #area #tangent #advancedmathproblems #calculation #problems #geometry
Any queries regarding the subject or videos are invited.
Instagram ID : problem_analysis
#maths #mathematics #circle #square #quartercircle #area #tangent #advancedmathproblems #calculation #problems #geometry
How to Find the Area of a Circle | Area of a Circle Step by Step
Area of a Circle | MathHelp.com
Area of a Circle | Beat the Calculator #shorts
Circle Area (classic visual proof)
Circle Area Derivation (visual proof)
Do you know Leonardo da Vinci's circle area proof?
Area of a Circle quick lesson
Circle area from peeling circumferences (pi day short)
Areas Related to Circles | Mathematics | 5th December 2024 | 7:00 PM Onwards
Area of circle
How to Calculate the Circumference of a Circle
The Area Of A Circle Formula - Simple Intuitive Explanation
Easy Way To Find The Area Of A Circle! #Shorts
How to find the Area of a Circle #shorts
Why is π = 3.14…??? #pivalue #circle #circles #geometry #maths #mathematics
GCSE Maths - How to find the Area and Circumference of a Circle (Circles Part 1) #106
How to Calculate the Area of a Circle
The Area of the Circle - Math for Kids
How to Find the Area of a Circle | Math with Mr. J
Quick Visual Proof: Area of a Circle
Circle area by exhaustion (two visual area techniques)
Area of a circle
Circle Area by Peeling Circumference
Area of a Segment in a Circle
Комментарии
 0:06:39
0:06:39
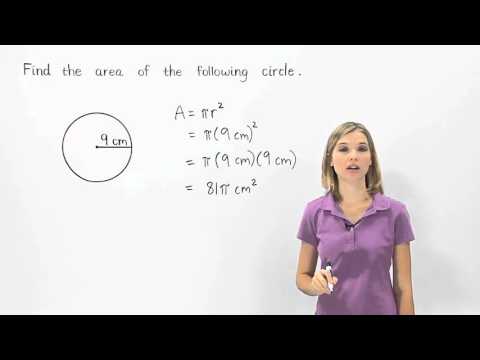 0:01:40
0:01:40
 0:00:52
0:00:52
 0:02:04
0:02:04
 0:00:25
0:00:25
 0:00:54
0:00:54
 0:00:58
0:00:58
 0:00:43
0:00:43
 0:47:46
0:47:46
 0:01:00
0:01:00
 0:00:59
0:00:59
 0:01:00
0:01:00
 0:00:30
0:00:30
 0:00:49
0:00:49
 0:00:31
0:00:31
 0:05:13
0:05:13
 0:02:27
0:02:27
 0:04:08
0:04:08
 0:09:08
0:09:08
 0:06:47
0:06:47
 0:01:00
0:01:00
 0:06:45
0:06:45
 0:01:10
0:01:10
 0:04:23
0:04:23