Using Mathematica for ODEs, Part 10. More modularity in Mathematica programming.

preview_player
Показать описание

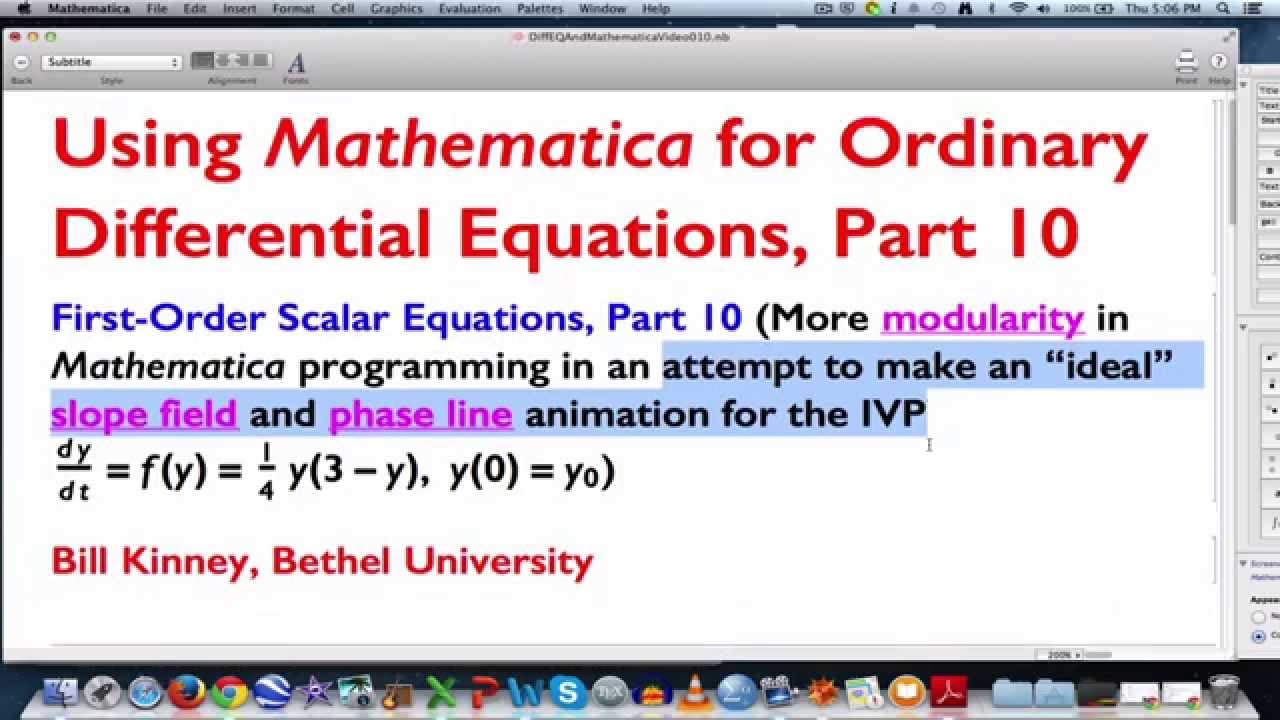
Using Mathematica for ODEs, Part 10. More modularity in Mathematica programming in an attempt to make an "ideal" slope field and phase line animation for the IVP dy/dt = f(y) = y*(3-y)/4, y(0) = y0.

AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
  1. Bill Kinney
  2. differential equation
  3. Mathematica (Software)
  4. Ordinary Differential Equation
  5. Mathematics (Field Of Study)
  6. ODE
Рекомендации по теме
Using Mathematica for 0:10:53

Using Mathematica for ODEs, Part 5 (Use Manipulate to see solutions change as parameters change)

Using Mathematica for 0:10:29

Using Mathematica for ODEs, Part 1 (Use DSolve and Plot for a pure antiderivative problem)

Using Mathematica for 0:13:17

Using Mathematica for ODEs, Part 10. More modularity in Mathematica programming.

Using Mathematica for 0:14:08

Using Mathematica for ODEs, Part 7...a smorgasbord of misc Mma and math subtleties

Using Mathematica for 0:12:32

Using Mathematica for ODEs, Part 4 (use DSolve, Plot, VectorPlot, Show, Simplify, Solve, & Apart...

Using Mathematica for 0:11:19

Using Mathematica for ODEs, Part 2 (Use VectorPlot and Show for a pure antiderivative problem)

Using Mathematica for 0:13:04

Using Mathematica for ODEs, Part 6 (using DSolveValue, Manipulate, VectorPlot, pure functions)

Solving Differential Equations(ODEs) 0:09:14

Solving Differential Equations(ODEs) in Mathematica | Tutorial -11

Using Mathematica for 0:14:11

Using Mathematica for ODEs, Part 8. Review slope fields for autonomous eqs. Phase lines.

Numerically Solve ODEs 0:05:08

Numerically Solve ODEs with Mathematica 2

Using Mathematica for 0:13:03

Using Mathematica for ODEs, Part 9. Equilibrium points (sinks & sources) on phase line. Modulari...

Using Mathematica for 0:10:32

Using Mathematica for ODEs, Part 3 (DSolve, VectorPlot...make a Slope Field for an Autonomous Eqn)

Numerically Solve ODEs 0:06:02

Numerically Solve ODEs with Mathematica 1

Solving ODEs Analytically 0:16:59

Solving ODEs Analytically Using Mathematica

Mathematica for ODEs 1:08:26

Mathematica for ODEs & Slope Fields: DSolve, DSolveValue, VectorPlot, Plot, ContourPlot, Manipul...

Two different ways 0:05:29

Two different ways to solve Partial differential equations ||(Mathematica tutorials-08)

Lecture 12 - 0:35:44

Lecture 12 - Solving Ordinary Differential Equations in Mathematica

Lecture 14 - 0:48:14

Lecture 14 - Implicit methods for ODEs (Hermite integration) in Mathematica

How to solve 0:05:42

How to solve first order coupled ODEs: Mathematica

Slope Fields & 0:07:56

Slope Fields & Solutions with DSolve on Mathematica for Nonautonomous Differential Equation Exam...

Solving Differential Equations 0:05:37

Solving Differential Equations in Mathematica with Boundary Conditions Given.

Mathematica: Formulate and 0:02:01

Mathematica: Formulate and solve a system of linear, first-order ODEs in Mathematica

Mathematica: NDSolve stiff 0:02:41

Mathematica: NDSolve stiff system of ODEs

Mathematica & Raspberry 0:06:36

Mathematica & Raspberry Pi - DSolve and 1st order ODEs

Комментарии
Автор

I was wondering whether you will do more videos of this series in the 2nd-order ODE later, especially for nonlinear non-homogeneous equation, or not?

I can see this series of videos mainly force on the introduction of Mathematica with ODE, instead of introduction of ODE with Mathematica. Function commands introduced here (up to 10 episodes) to solve 1st-order ODE should be able to extended to generate the solutions of most of the linear 2nd-order ODE for sure.

However it will be nice to see more videos to introduce it in the great detail.

eson