filmov
tv
Calculus 2, Lec 15E: Arc Length of a Polar Curve (Elliptic Function Needed to Evaluate for Limaçon)

Показать описание
In Calculus, we represent a polar curve r=f(θ) with parametric equations as x=f(θ)cos(θ), y=f(θ)sin(θ). The integrand of the arc length integral is then ((dx/dθ)^2+(dy/dθ)^2)^(1/2). This ends up simplifying to ((f(θ))^2 + (f'(θ))^2)^(1/2) because of trigonometric identities. When we apply this to the example f(θ)=2+4cos(θ), which is a limaçon, we get an integral that cannot be exactly evaluated without the use of a special function, called an elliptic function.
Links and resources
===============================
(0:00) Arc length integral formula in terms of parameterization x(θ) and y(θ) of polar curve r=f(θ)
(2:02) Simplify the formula to relate it to r=f(θ) and dr/dθ=f'(θ)
(6:06) Arc length integral for limaçon r=f(θ)=2+4cos(θ)
(7:42) Mathematica shows an Elliptic function is necessary
(9:46) Approximate arc length of limaçon r=f(θ)=2+4cos(θ)
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
Links and resources
===============================
(0:00) Arc length integral formula in terms of parameterization x(θ) and y(θ) of polar curve r=f(θ)
(2:02) Simplify the formula to relate it to r=f(θ) and dr/dθ=f'(θ)
(6:06) Arc length integral for limaçon r=f(θ)=2+4cos(θ)
(7:42) Mathematica shows an Elliptic function is necessary
(9:46) Approximate arc length of limaçon r=f(θ)=2+4cos(θ)
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
 0:10:51
0:10:51
 0:49:31
0:49:31
 0:53:55
0:53:55
 0:10:15
0:10:15
 0:10:29
0:10:29
 0:11:14
0:11:14
 0:47:58
0:47:58
 0:00:56
0:00:56
 0:04:25
0:04:25
![[Math 22] Lec](https://i.ytimg.com/vi/Cx1k-Kf8gpA/hqdefault.jpg) 0:22:40
0:22:40
 0:36:20
0:36:20
 2:12:45
2:12:45
![[Math 22] Lec](https://i.ytimg.com/vi/W8ycNiOPeVk/hqdefault.jpg) 0:43:44
0:43:44
 0:00:15
0:00:15
 0:00:48
0:00:48
 0:12:40
0:12:40
 0:00:20
0:00:20
 0:16:45
0:16:45
 0:59:40
0:59:40
 0:00:15
0:00:15
 0:00:47
0:00:47
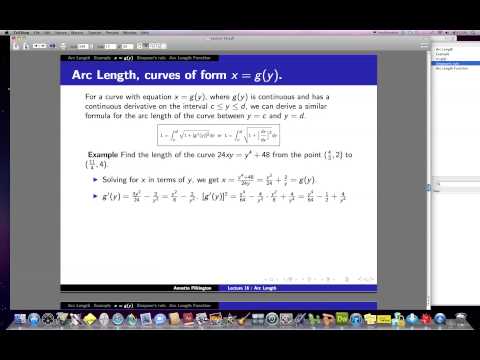 0:13:18
0:13:18
 1:15:51
1:15:51
 0:12:19
0:12:19