Solving Natural Logarithms | MathHelp.com

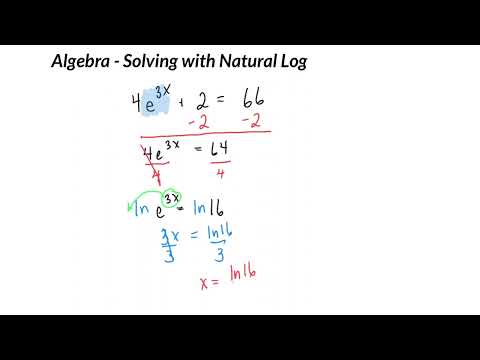
In this example, which involves natural logarithms, we’re asked to solve each of the following equations for x, and leave our answers in terms of e. To solve for x in the first equation, ln x = 3, we simply switch the equation from logarithmic to exponential form. Remember that ln x means the natural logarithm of x, and a natural log has a base of e. So, to convert the given equation to exponential form, remember that the base of the log represents the base of the power, the right side of the equation represents the exponent, and the number inside the log represents the result, so we have e…to the 3rd…= x, and we’ve solved for x. Notice that our answer, e cubed, is written in terms of e, which is what the problem asks us to do. Now, let’s take a look at the second equation, ln x squared = 8. Again, we solve for x by switching the equation from logarithmic to exponential form. Ln x squared means the natural logarithm of x squared, and a natural log has a base of e. So, converting the equation to exponential form, we have e…to the 8th…= x squared. Next, since x is squared, we take the square root of both sides. On the right, the square root of x squared is x. On the left, however, there are a couple of things to watch out for. First, remember that the square root of e to the 8th is the same thing as e to the 8th to the ½, which simplifies to e to the 8 times ½, or e to the 4th. Also, remember that when we take the square root of both sides of an equation, we use plus or minus, so our final answer is plus or minus e to the 4th = x.