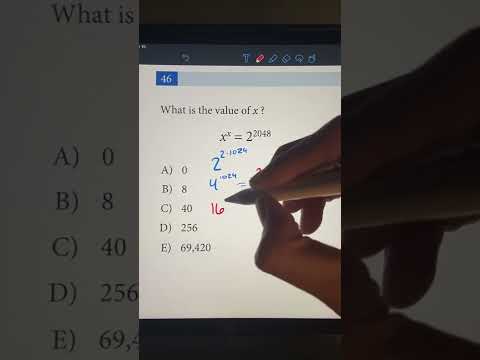
SAT MATH problem System of Quadratic Equations

#shorts #sat #math
Another system of quadratic equation. This one is not bad once you understand how the problems work.

[Littlebitofthateverything]

Yo, found this channel, and it suits my levels, not extremely hard yet not very easy, keep it up

[premnath]

Other way is that just subtract -2xy from both sides. In LHS you will get (x-y)² and in RHS just write the value of xy and solve and the answer comes 16

[Powerdeadmau]

All I did without doing it quadratically was use the "xy= -3". So I know for a fact either x and y must be "(3, -3), (-1, 1)", or well, 3 and -1, -3 and 1, etc.
Afterwards since x² + y² = 10, it's 9+1 = 10

And by this point we know it's 3 and 1, and one's negative. So (x-y)² can either be if:
(3 - [-1])² = (3+1)² = (4)² = 16
(-3-1)² = -4² = 16
Both go to 16

[thedomshoe]

you could do a system of equations with
x²+y²=10
xy=-3
and plug in, but that is a pretty clever way to do it though. like it alot

[connorf]

-3=x and 1=y, or vice Versa and plug in: (-3-1)^2=16; (-1-3)^2=16. Imo a lot faster and easier

[Jujudemonn]

You also could’ve substituted Sum(squared) - 2 product instead of xsquared - ysquared

[rayes]

CLEAN STEPS!!! Can I also solve it by substitution?

[drabyashchitrakar]

X² + y² = 10
(X - y)² + 2xy = 10
(X - y)² +2 × (-3) = 10
(X - y)² - 6 = 10
(X - y)² = 16 .. this one must be the easiest.

[spacebeatz]

Lol i did just plug the xy ang got it faster.