filmov
tv
Linear Systems Theory

Показать описание
In this lecture we will discuss linear systems theory which is based upon the superposition principles of additivity and homogeneity, we will explore both of these principals separately to get a clear understanding of what they mean and the basic assumptions behind each
Linear Systems Theory
Before we talk about nonlinear systems we need to first have a basic understand what a linear system is. Linear systems are defined by their adherence to what is called the superposition principles. There are just two superposition principles and they are called homogeneity and additively.
Firstly additively, which states that we can add the effect or output of two systems together and the resulting combined system will be nothing more that the simple addition of each system’s output in isolation. So for example, if I have two horses that can each pull a hundred kilograms of weight on a cart in isolation, well if I then combine these two horses to tow a single larger cart they will be able to pull twice as much weight.
Another way of stating the additivity principle is that for all linear systems, the net response caused by two or more stimuli is the sum of the response which would have been caused by each stimulus individually.
Our second superposition principle, homogeneity, states that the output to a linear system is always directly proportional to the input, so if we put twice as much into the system we will in turn get out twice as much.
For example, if I pay 50 dollars for a hotel room, for which I will get a certain quality of service, this principle states that if I pay twice as much I will then get an accommodation service that is twice a good. When we plot this on a graph we will see why linear systems are called linear because the result will always be a straight line.
These principles are of cause deeply intuitive to us and will appear very simple, but behind them are a basic set of assumptions about how the world works, so lets take a closer look at these assumptions that support the theory of linear systems. Essentially what these principles are saying is that it is the properties of the system in isolation that really matter and not the way these thing are put together or the nature of the relationships between them, this is of cause very abstract so lets illustrate it with some examples.
Imagine you have some ailment and you have two drugs that you know are meant to cure this problem, so you take them both at the same time. The result of this, or we might say the output to this system, will depend on whether the two drugs have any effect on each other when taken in combination. If the drugs have no effect on each other then it will be the properties of each drug in isolation that will define the overall output to the system, and because of this lack of interaction between the components our linear model will be able to fully capture and describe this phenomena.
Learn about the Systems Innovation Network on our social media:
Комментарии
 0:24:47
0:24:47
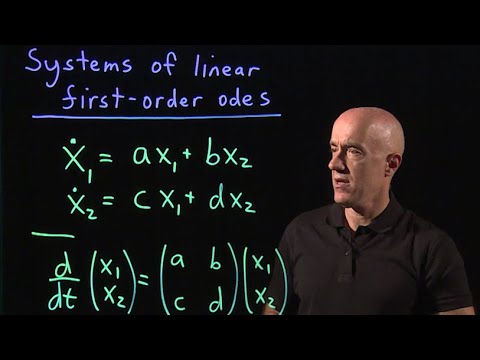 0:08:28
0:08:28
 0:04:03
0:04:03
 0:05:59
0:05:59
 0:19:53
0:19:53
 1:14:10
1:14:10
 0:35:36
0:35:36
 0:39:40
0:39:40
![[ICFP'24] Green -](https://i.ytimg.com/vi/F70QZaMoYJQ/hqdefault.jpg) 8:05:13
8:05:13
 0:14:22
0:14:22
 0:07:33
0:07:33
 0:13:25
0:13:25
 0:19:25
0:19:25
 1:18:32
1:18:32
 0:16:08
0:16:08
 0:09:57
0:09:57
 1:18:14
1:18:14
 0:33:38
0:33:38
 1:06:30
1:06:30
 1:17:47
1:17:47
 1:17:59
1:17:59
 0:15:48
0:15:48
 0:03:59
0:03:59
 0:13:11
0:13:11