filmov
tv
Two Amazing Machin-like Formulas for Pi/4 (Pi Approximation Day Visual Proofs)
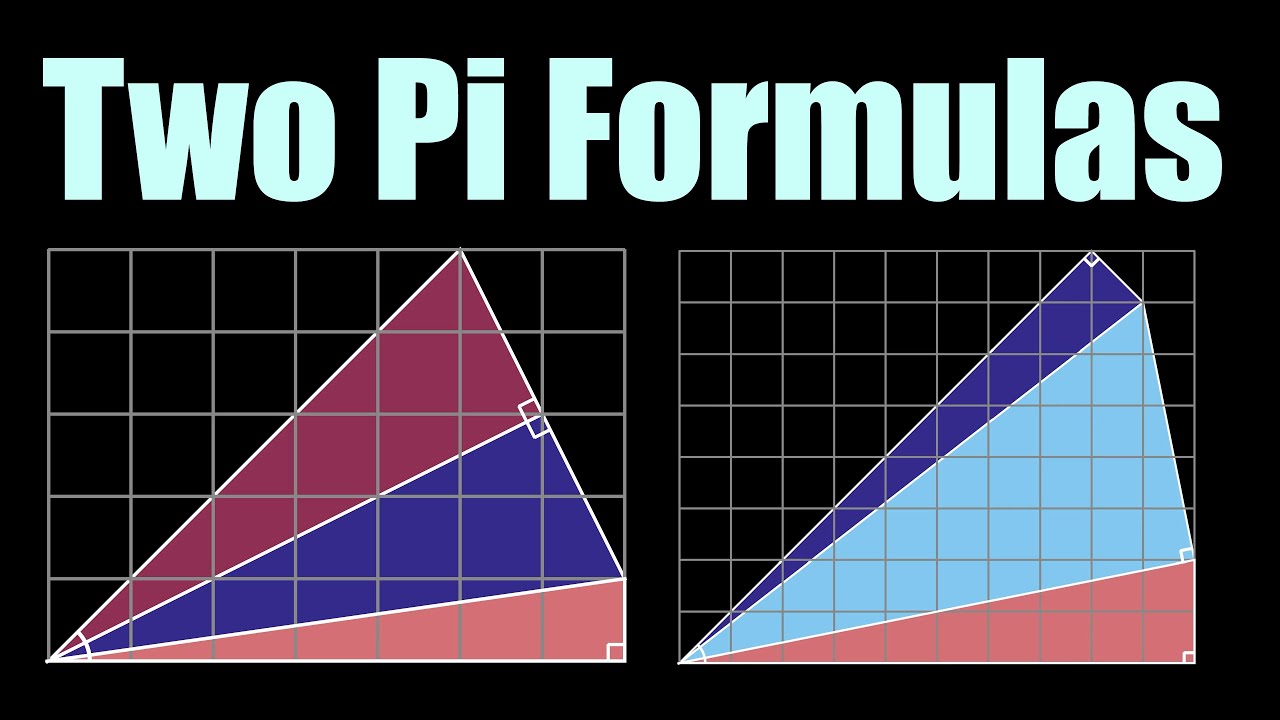
Показать описание
This video describes a relatively new visual proof of two different Machin-like formulas for Pi (or for Pi/4 more accurately), which is a formula that allows for relatively speedy computation of the digits of Pi. These two formulas are called Hutton's formula and Strassnitzky's formula although at least Hutton's was likely known to Machin.
#mathvideo math #pi #piday #geometry #inscribed #circumscribed #triangle #manim #animation #theorem #pww #proofwithoutwords #visualproof #proof #similartriangles #mathvideo #machinformula #machintheorem #digitsofpi #trapezoid
Here are three more Pi day gems:
To learn more about animating with manim, check out:
____________________________________________________
Background music:
Frozen in Love by Aakash Gandhi
#mathvideo math #pi #piday #geometry #inscribed #circumscribed #triangle #manim #animation #theorem #pww #proofwithoutwords #visualproof #proof #similartriangles #mathvideo #machinformula #machintheorem #digitsofpi #trapezoid
Here are three more Pi day gems:
To learn more about animating with manim, check out:
____________________________________________________
Background music:
Frozen in Love by Aakash Gandhi
Computing π: Machin-like formula
Machin Formula Visualization (Pi day special)
Can you guess the math formula?
An Exact Formula for the Primes: Willans' Formula
Reasons I regret formula-feeding my child
Change number in date format | Excel formulas | Text Function
The Better Quadratic Formula You Won't Be Taught
Sumifs formula in excel | Excel formula #shorts #sumifs
NYCU Data Science 2024 Week7: Machine Learning with scikit-learn (1)
What is the Formula for Power 🤔 This Trick Will Help you Remember...
Excel Formulas and Functions You NEED to KNOW!
This equation will change how you see the world (the logistic map)
how to solve rubik's cube 3x3 - cube solve magic trick formula #shorts
POWER EQUATION P = 2 π n t / 60 - Why ? Learn with Animation
GCSE Maths - How to Rearrange Formulas #48
Jim Simons: My Formula for Making Billions
The Trillion Dollar Equation
Master the Perfect ChatGPT Prompt Formula (in just 8 minutes)!
MS Excel LOOKUP Formula: Return Multiple Values
The Cost of a Formula 1 Car - Lewis Hamilton
please STEAL this hard trap melody formula🙏
Efficiency Formula | Physics Animation
Touching mercury
Liquid oxygen is magnetic! 🧲
Комментарии
 0:11:21
0:11:21
 0:03:15
0:03:15
 0:00:53
0:00:53
 0:14:47
0:14:47
 0:00:05
0:00:05
 0:00:24
0:00:24
 0:03:02
0:03:02
 0:00:18
0:00:18
 2:56:20
2:56:20
 0:00:42
0:00:42
 0:10:47
0:10:47
 0:18:39
0:18:39
 0:00:35
0:00:35
 0:06:00
0:06:00
 0:04:16
0:04:16
 0:00:51
0:00:51
 0:31:22
0:31:22
 0:08:30
0:08:30
 0:01:00
0:01:00
 0:00:28
0:00:28
 0:00:39
0:00:39
 0:01:31
0:01:31
 0:00:39
0:00:39
 0:00:33
0:00:33