Synthetic Division | MathHelp.com

Показать описание

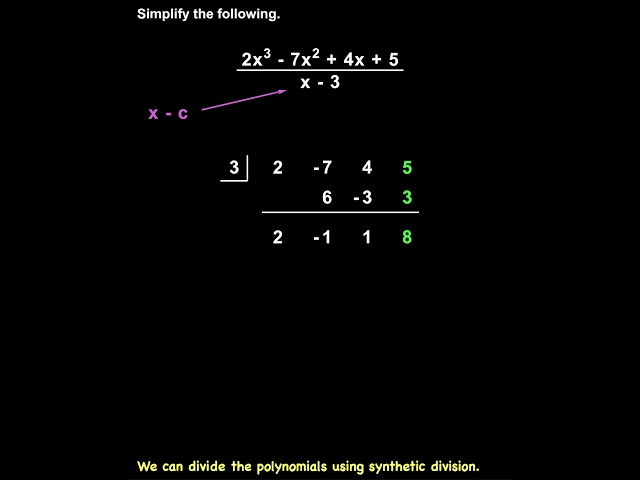
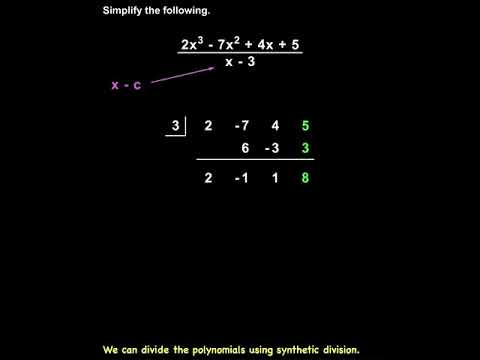
In this example, notice that we have a polynomial divided by a binomial, and our binomial is in the form of an x term minus a constant term, or x – c. In this situation, instead of having to use long division, like we did in the previous lesson, we can divide the polynomials using synthetic division, which is a much more efficient method. Here’s how it works. We start by finding the value of c. Since –c = -3, we know that c = 3. Next, we put the value of c inside a box, so we put the 3 inside a box. It’s very important to understand that the number that goes inside the box always uses the opposite sign as the constant term in the binomial. In other words, since the constant term in the binomial is -3, the number that goes inside the box, is positive 3. Next, we write the coefficients of the dividend, which are 2, -7, 4, and 5. Be very careful with your signs. Now, we’re ready to start our synthetic division. First, we bring down the 2. Next, we multiply the 3 in the box times 2 to get 6, and we put the 6 under the -7. Next, we add -7 + 6 to get -1. Next, we multiply the 3 in the box times -1 to get -3, and we put the -3 under the 4. Next, we add 4 + -3 to get 1. Next, we multiply the 3 in the box times 1 to get 3, and we put the 3 under the 5. Finally, we add 5 + 3 to get 8. Now, notice that we have a 2, -1, 1, and 8 in the bottom row of our synthetic division. These values will give us our answer: the first 3 numbers represent the coefficients of the quotient, and the last number is the remainder. And it’s important to understand that our answer will be one degree less than the dividend. In other words, since our dividend starts with x cubed, and we’re dividing by x, our answer will start with x squared. So our answer is 2x squared – 1x + 1 + 8 over x – 3. Notice that we always use descending order of powers in our quotient. In this case x squared, x, and the constant. Finally, remember that we add the remainder over the divisor, just like we did in the previous lesson on long division, and we have our answer. It’s important to understand that we’ll get the same answer whether we use synthetic division or long division. However, synthetic division is much faster.