Finding the Midpoint of Two Points - Explained with Examples! | Midpoint Formula, Analytic Geometry

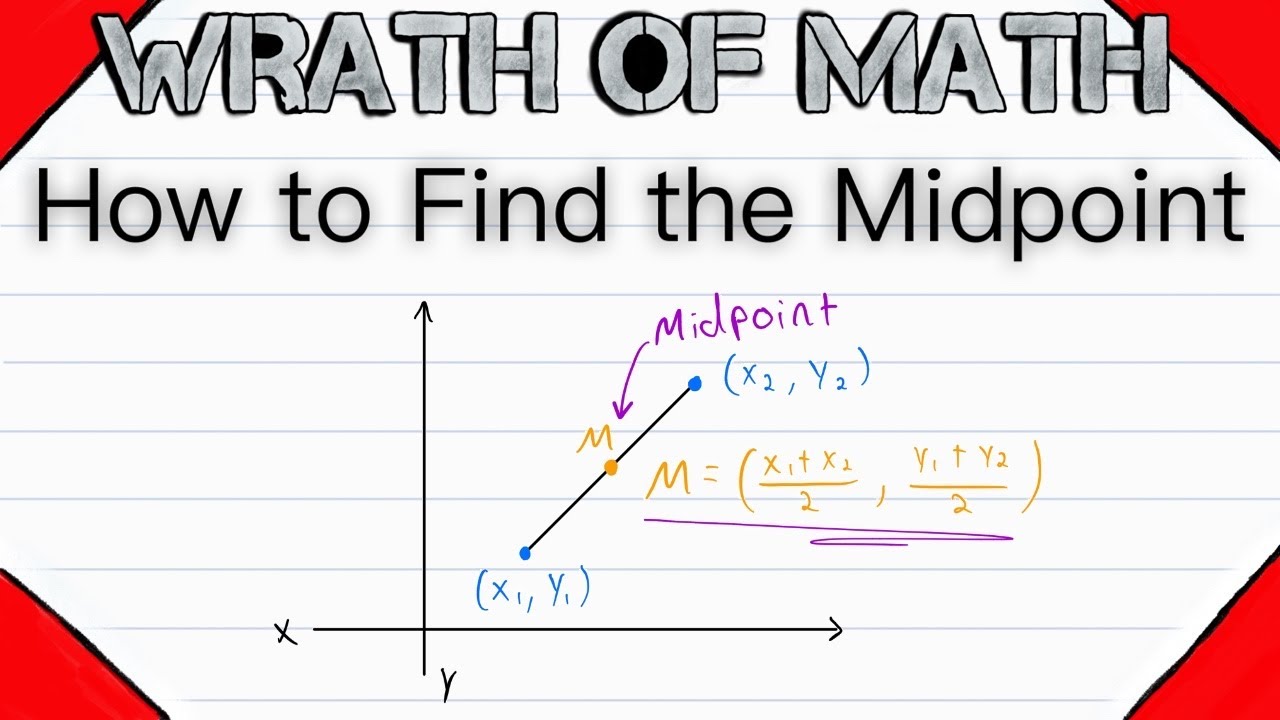
Given two points on the xy plane, how do we find the midpoint? If P and Q are two points in the plane, how do we find the midpoint of segment PQ? That's what we'll be going over in today's lesson - how to find the coordinates of the midpoint of two points!

Let point P = (x1, y1) and point Q = (x2, y2). If M is the midpoint of P and Q, then it must be halfway between the horizontal and vertical positions of P and Q. Thus, the x coordinate of M must be the average of the x coordinates of P and Q, as in (x1 + x2) / 2. Similarly, the y coordinate of M must be the average of the y coordinates of P and Q, as in (y1 + y2) / 2.

Thus, M = ( (x1 + x2)/2, (y1 + y2)/2 ).

SOLUTION TO PRACTICE PROBLEM:

We want to find M, the midpoint of (-1, 4) and (3, -2). First, average the x coordinates: (-1 + 3)/2 = 1. Then average the y coordinates: (4 + (-2))/2 = 1. So M = (1, 1).

I hope you find this video helpful, and be sure to ask any questions down in the comments!

********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.

********************************************************************

+WRATH OF MATH+

Follow Wrath of Math on...