filmov
tv
The Most Overlooked Concept in Calculus - Calculus of Inverse Functions
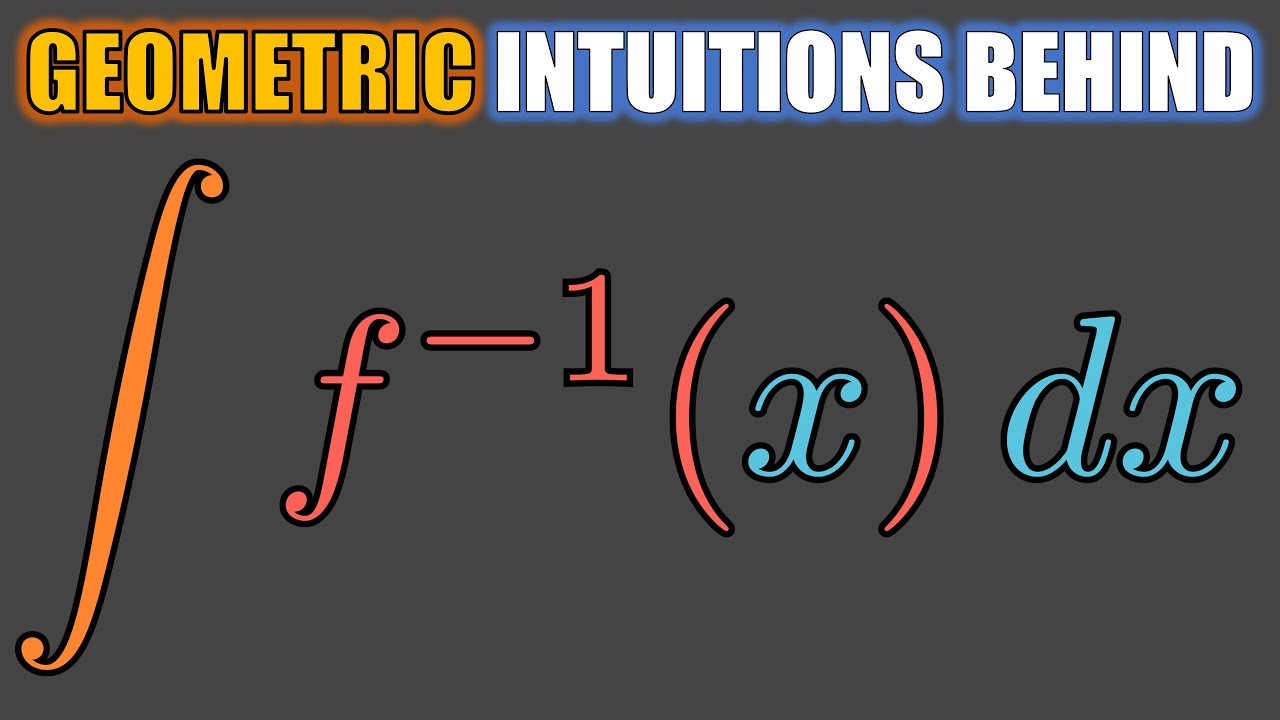
Показать описание
In this video, we look at one of the most overlooked concept in calculus, which is the derivatives and the integrals of inverse functions.
Chapters:
00:00 Inverse Functions (Intro)
01:54 Finding Inverse is Hard (Intro)
04:37 Derivative of Inverse Functions
06:28 Integral of Inverse Functions
08:38 Using Them to Solve Challenging Problems
09:58 Applications (Outro)
Music🎵:
Corrections:
2:16 omega should be [-1+sqrt(-3)]/2 (*principal cube root of unity)
4:13 denominator should be f'(f inverse of x)
Chapters:
00:00 Inverse Functions (Intro)
01:54 Finding Inverse is Hard (Intro)
04:37 Derivative of Inverse Functions
06:28 Integral of Inverse Functions
08:38 Using Them to Solve Challenging Problems
09:58 Applications (Outro)
Music🎵:
Corrections:
2:16 omega should be [-1+sqrt(-3)]/2 (*principal cube root of unity)
4:13 denominator should be f'(f inverse of x)
The Most Overlooked Concept in Calculus - Calculus of Inverse Functions
The Most Overlooked Concept In Bass Fishing!
The Most Overlooked Concept In All Of Sports Performance?? Adaptive Energy
The 2 Most OVERLOOKED concepts in Level Design
The Most Overlooked Concept in Math
Most overlooked but important concept in Python
Age-Adjusted Net Worth: The Most Overlooked Concept in Business 💡
The #1 MOST OVERLOOKED Concept ONLY PROS EXPLOIT - League of Legends
The Private Equity Strategy Most Overlook: How To Profit From Failing & Bankrupt Businesses
SHRM Certification: #1 Most Overlooked Concept
The Most Powerful Yet Overlooked Resource in Schools | Heejae Lim | TED
This is THE MOST overlooked defensive mechanic
This is one of the most overlooked skills in pickleball (VIDEO 8/21) #pickeball
Most Overlooked Decks VR to @the_hermits_cave
Kirk Parsley - The Most Overlooked Factor in Health and Longevity
The Most OVERLOOKED Mechanic in Street Fighter 6
Kirk Parsley: The Most Overlooked Factor in Health and Longevity
The Most Overlooked Guard in Jiu Jitsu
The Most OVERLOOKED Method Of Ranking Up In Rocket League
Details about the Envy you missed in Inside Out 2 😱
10 Years of Motion Design Knowledge In 7 Minutes
Most overlooked fact about how Warren Buffett amassed his fortune | Value investing | Steady returns
Most Overlooked Chord In C Major #guitar
I never wear these | Re-styling the most overlooked pieces in my wardrobe | Fall 2024
Комментарии
 0:11:41
0:11:41
 0:12:17
0:12:17
 0:06:35
0:06:35
 0:08:20
0:08:20
 0:00:54
0:00:54
 0:17:20
0:17:20
 0:00:42
0:00:42
 0:09:34
0:09:34
 0:34:38
0:34:38
 0:04:18
0:04:18
 0:05:12
0:05:12
 0:17:01
0:17:01
 0:00:49
0:00:49
 0:20:30
0:20:30
 1:02:52
1:02:52
 0:04:33
0:04:33
 1:04:54
1:04:54
 0:05:46
0:05:46
 0:12:47
0:12:47
 0:00:42
0:00:42
 0:07:13
0:07:13
 0:00:06
0:00:06
 0:00:31
0:00:31
 0:14:09
0:14:09