filmov
tv
Laplace's Equation on a Rectangle - Partial Differential Equation | Lecture 9

Показать описание
Equilibrium temperature distributions in higher spatial dimensions come from solving a PDE called Laplace's equation. Laplace's equation is difficult to solve in general and its solutions form the basis for harmonic theory. In this lecture we solve Laplace's equation on a rectangle, showing how we can decompose the solution using the principle of superposition and solve each component using separation of variables.
Follow @jbramburger7 on Twitter for updates.
Follow @jbramburger7 on Twitter for updates.
Laplace's Equation on a Rectangle
Laplace's Equation on a Rectangle - Partial Differential Equation | Lecture 9
Partial Differential Equation with Dirichlet Boundary Conditions (With Example)
Solution to Laplace’s equation on rectangular domains PART 1
Laplace Equation on a Rectangle, Example #1 [MATH-241] [PDEs]
PDE 101: Separation of Variables! ...or how I learned to stop worrying and solve Laplace's equa...
Laplace's Equation on a Rectangle with one Non Zero Boundary Condition
Separation of variables to solve Laplace's equation for the V in a cube 3D rectangular coordina...
Homogeneous Laplace's Equation on a Rectangle (Part 2)
Laplace Transform of a Piecewise Function (Unit Step Function)
Laplace's Equation (part 1): Introduction and Solutions on a Rectangular Domain
V9-9: Laplace Equation in rectangle, Dirichlet boundary conditions, derivation of formal solution.
Laplace transform of unit step function & rectangular window function
Laplace's Equation In Rectangular Coordinate (Part-1) (Hindi)
Separation of Variables - Laplace Eq Part 1
Partial Differential Equations - 5.9 - Laplace's Equation - Part 1 of 2
ME565 Lecture 10: Analytic Solution to Laplace's Equation in 2D (on rectangle)
Lecture 59 - Laplace equation over a rectangle
Human Calculator Solves World’s Longest Math Problem #shorts
How REAL Men Integrate Functions
Working Rule of solving two dimensional Laplace's Equation,( Rectangle)(Seperation of Variable...
NEWYES Calculator VS Casio calculator
#8. Rectangular Function | Laplace Transform | Signals and Systems
mod07lec67 - The Laplace Equation: Dirichlet and Neumann boundary conditions
Комментарии
 0:18:07
0:18:07
 0:20:19
0:20:19
 0:39:45
0:39:45
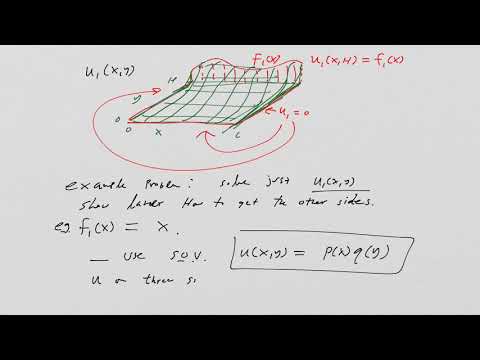 0:33:03
0:33:03
 0:18:05
0:18:05
 0:49:54
0:49:54
 0:18:49
0:18:49
 1:13:27
1:13:27
 0:19:30
0:19:30
 0:06:27
0:06:27
 0:45:55
0:45:55
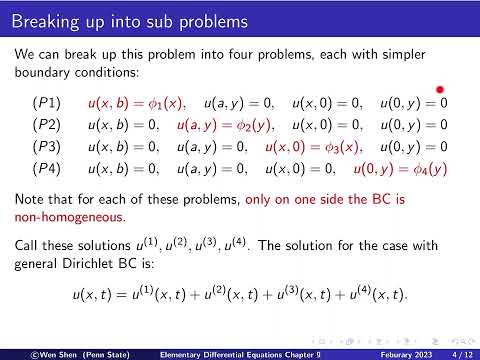 0:21:46
0:21:46
 0:07:07
0:07:07
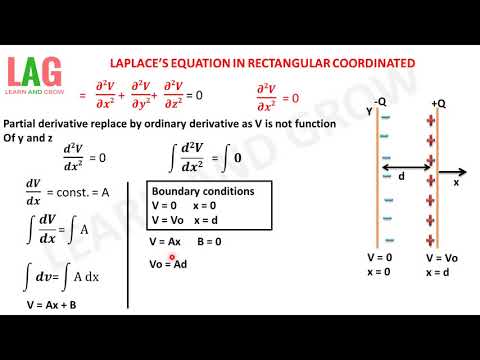 0:11:05
0:11:05
 0:07:10
0:07:10
 0:18:02
0:18:02
 0:48:05
0:48:05
 0:31:01
0:31:01
 0:00:34
0:00:34
 0:00:35
0:00:35
 0:03:30
0:03:30
 0:00:14
0:00:14
 0:10:42
0:10:42
 0:28:50
0:28:50