APRG Seminar: 2022-02-16 - Loredana Lanzani

APRG Seminar, Indian Institute of Science (Mathematics)

Speaker: Loredana Lanzani (Syracuse University, USA)

Title: The Cauchy-Szego projection and its commutator for domains in C^n with minimal smoothness: Optimal bounds

Abstract:
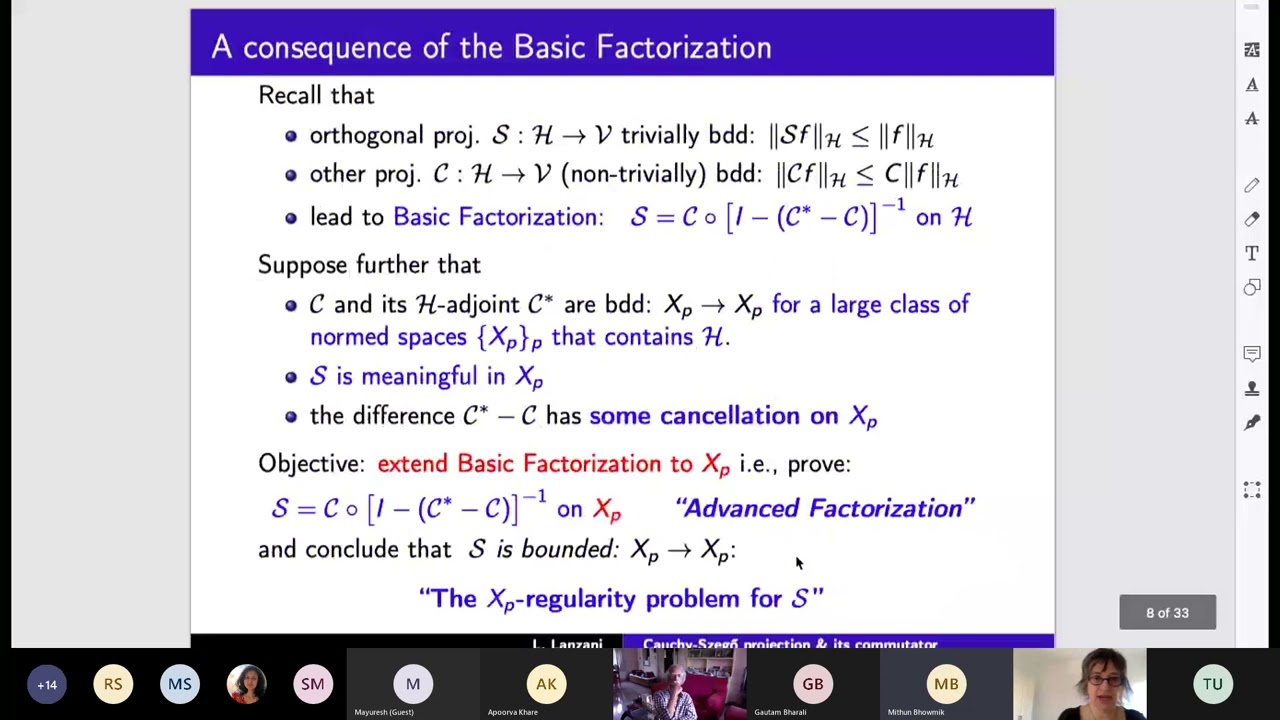
Let D ⊂ \C^n be a bounded, strongly pseudoconvex domain whose boundary bD satisfies the minimal regularity condition of class C^2. A 2017 result of Lanzani & E. M. Stein states that the Cauchy–Szegö projection S_ω maps L^p(bD,ω) to L^p(bD,ω) continuously for any p∈(1,∞) whenever the reference measure ω is a bounded, positive continuous multiple of induced Lebesgue measure. Here we show that S_ω (defined with respect to any measure ω as above) satisfies explicit, optimal bounds in L^p(bD,Ω_p), for any p∈(1,∞) and for any Ω_p in the maximal class of A_p-measures, that is Ω_p=ψ_p.σ where ψ_p is a Muckenhoupt A_p-weight and σ is the induced Lebesgue measure. As an application, we characterize boundedness in L^p(bD,Ω_p) with explicit bounds, and compactness, of the commutator [b,S_ω] for any A_p-measure Ω_p, p∈(1,∞). We next introduce the notion of holomorphic Hardy spaces for A_p-measures, and we characterize boundedness and compactness in L^2(bD,Ω_2) of the commutator [b,S_{Ω_2}] where S_{Ω_2} is the Cauchy–Szegö projection defined with respect to any given A_2-measure Ω_2. Earlier results rely upon an asymptotic expansion and subsequent pointwise estimates of the Cauchy–Szegö kernel, but these are unavailable in our setting of minimal regularity of bD; at the same time, recent techniques that allow to handle domains with minimal regularity, are not applicable to A_p-measures. It turns out that the method of quantitative extrapolation is an appropriate replacement for the missing tools.