Integration By Parts Full Explanation in 4 minutes

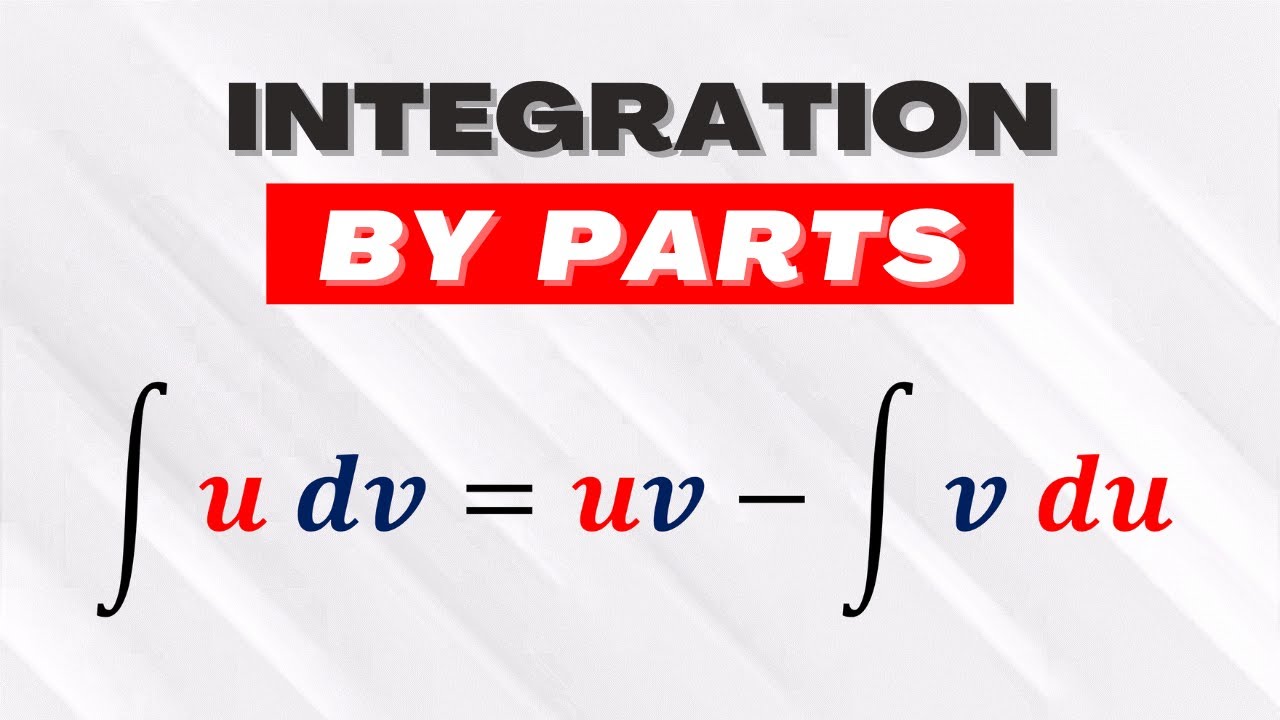
Integration by parts is used when integrating a product of function whose factors are different. Integration by parts is the reverse of Product Rule in differentiation. In this video, we will be looking on when to use integration by parts, the derivation of the formula, how to select part of the function to be 'u', and solving an example using Integration By Parts.

0:00 When to use by parts
0:47 Derivation of by parts formula
1:44 Rule for selection of u
2:17 Choosing u and dv
2:55 Solving example using by parts

[anglelee]

Clear and direct explanation! Best explanation for by parts found so far. Good job. Keep it up.

[ingGS]

You do a great job at explaining without skipping essential steps. Congratulations!

[mohanbabupm]

Wow wow I comprehended instantly this is how o
A teacher should I hit and subscribed I mean the bell button

[futurewarships]

Thanks for making this, these videos really do help people out!!

[benjaminmatanovic]

I'm preparing for my exams, and I wanted to give praise to your explanation. You presented this topic in a very clear and intuitive way for people to understand. Very well!

[denggabriel]

This is the first video on YouTube that am commenting on, but woow, your explanation is on another level. Thanks please, much appreciated.

[abelmulila]

Thanks for a clearly detailed explanation 🎉🎉

[prabak]

Good effort. Very brief and apt. Thank you.

[jitendrathakran]

Neat explanation, God bless, thank you

[jackkalver]

In the case of a pure inverse function, the integration by parts formula can be derived from the graph: int x dy=x*y–int y dx

[hasithadilshan]

Could you please send soft copy of above video

[nayikinisrinivas]

Sir I am a 14yr I'm not understanding this is it OK or anything problem

[rogeraak]

I never use this method. I use the D-I method. Much, much easier. And much easier to learn.