filmov
tv
Limit with floor
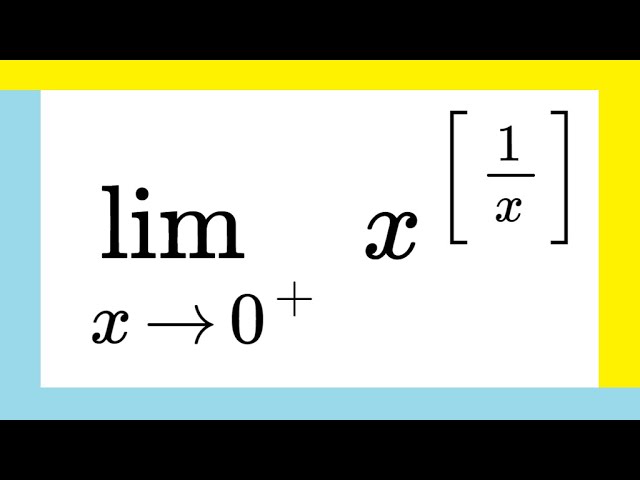
Показать описание
Here I evaluate a neat calculus limit with the floor function, namely the limit as x goes to 0 from the right of x to the floor of 1/x. It's a nice application of the definition of floor, and a quick and tasty math snack. This problem can be found in chapter 2 of Stewart's calculus textbook.
Limit with floor
Limit of Floor(x) or Greatest Integer Function [x]
Limit of Floor Function of 6x-x^2 | Many People Got this Wrong!
Limit of a floor function
floor(0.999...)=?
Evaluating limit of flooring and ceiling functions | Limit of flooring & ceiling functions
A floored limit.
Limit of floor function Thomas calculus.Ex.2.4
TCMU opening SEAsations exhibit this weekend
Limit Fungsi Tangga (Floor)
Limit Fungsi Floor
Example 8: Evaluating a limit (Greatest Integer Function)
A cool floor limit
High heels limit her Combat Effectiveness🤙🔥 | I Am Nobody | YOUKU Shorts
a crazy integral - floor function - limit problem
One Side Limit For Greatest Integer Step Function
2 Unlimited - No Limit (Rap Version) (Official Music Video)
Limit Of Floor
Sea Floor is the Limit | Aesthetic Freediving Footage
Limit of x[x]: Product of x and Greatest Integer Function
Installing Gibidi Floor motor into foundation box with limit switch
2 Unlimited - No limit - Live 2023
What does one mean by the limit x goes to 0? | Intro. to Mechanics | S-7
Hot Limit (T8-Floor P-Mix)
Комментарии
 0:02:11
0:02:11
 0:03:34
0:03:34
 0:08:44
0:08:44
 0:01:52
0:01:52
 0:03:05
0:03:05
 0:04:23
0:04:23
 0:16:32
0:16:32
 0:03:53
0:03:53
 0:04:38
0:04:38
 0:04:05
0:04:05
 0:10:12
0:10:12
 0:02:55
0:02:55
 0:07:50
0:07:50
 0:00:32
0:00:32
 0:10:17
0:10:17
 0:05:33
0:05:33
 0:03:46
0:03:46
 0:03:40
0:03:40
 0:04:35
0:04:35
![Limit of x[x]:](https://i.ytimg.com/vi/YOUZhs4DRUE/hqdefault.jpg) 0:08:03
0:08:03
 0:01:24
0:01:24
 0:04:27
0:04:27
 0:10:55
0:10:55
 0:04:10
0:04:10