filmov
tv
A Fibonacci bounded partial sum of the Harmonic series.

Показать описание
We determine the limit of a certain sequence defined in terms of Fibonacci and Harmonic numbers.
A Fibonacci bounded partial sum of the Harmonic series.
The Millin Series (A nice Fibonacci sum)
A nice Fibonacci reciprocal sum!
Using partial fractions to evaluate two Fibonacci reciprocal sums.
A nice Fibonacci sum done two ways!!
Partial Sums of Harmonic Series pg 10
Multiplicative Fibonacci-like
An inverse tangent Fibonacci sum!
An infinite product involving Fibonacci numbers!
Students in first year.. 😂 | #shorts #jennyslectures #jayantikhatrilamba
Florian Luca: Fibonacci numbers and repdigits
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
What is the first Fibonacci number greater than 10,000?
OODLES of FUN with Sequences and Series (Direct & Recursive Formulas, Series & Partial Sums)
Johann Brauchart - Spherical Fibonacci Points: Weyl sums, ...
MAT 134 -- Lecture 30 -- Sequences
a very Fibonacci product!
Learn how to evaluate the partial sum of a series
NEWYES Calculator VS Casio calculator
Tribonacci numbers | Fibonacci numbers | nth Fibonacci number | Binet’s formula | mathocube |
MAT157 - March 8, 2022
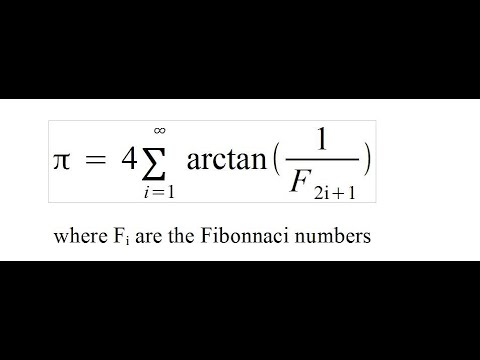
Infinite arctan sum involving Fibonacci numbers. Why Pi appers?
I met @AmanDhattarwal bhaiya | Lovely Professional University
Mathematics: Is there a partial sum formula for the Harmonic Series?
Комментарии
 0:15:21
0:15:21
 0:22:55
0:22:55
 0:23:09
0:23:09
 0:16:53
0:16:53
 0:16:13
0:16:13
 0:07:53
0:07:53
 0:14:00
0:14:00
 0:13:26
0:13:26
 0:20:47
0:20:47
 0:00:11
0:00:11
 0:53:36
0:53:36
 0:00:15
0:00:15
 0:04:53
0:04:53
 1:09:34
1:09:34
 0:39:34
0:39:34
 1:18:10
1:18:10
 0:15:02
0:15:02
 0:01:49
0:01:49
 0:00:14
0:00:14
 0:04:35
0:04:35
 1:40:30
1:40:30
 0:13:01
0:13:01
 0:00:25
0:00:25
 0:01:52
0:01:52