filmov
tv
Emil Prodan | Topological Bulk-Boundary Correspondence via K-Theory
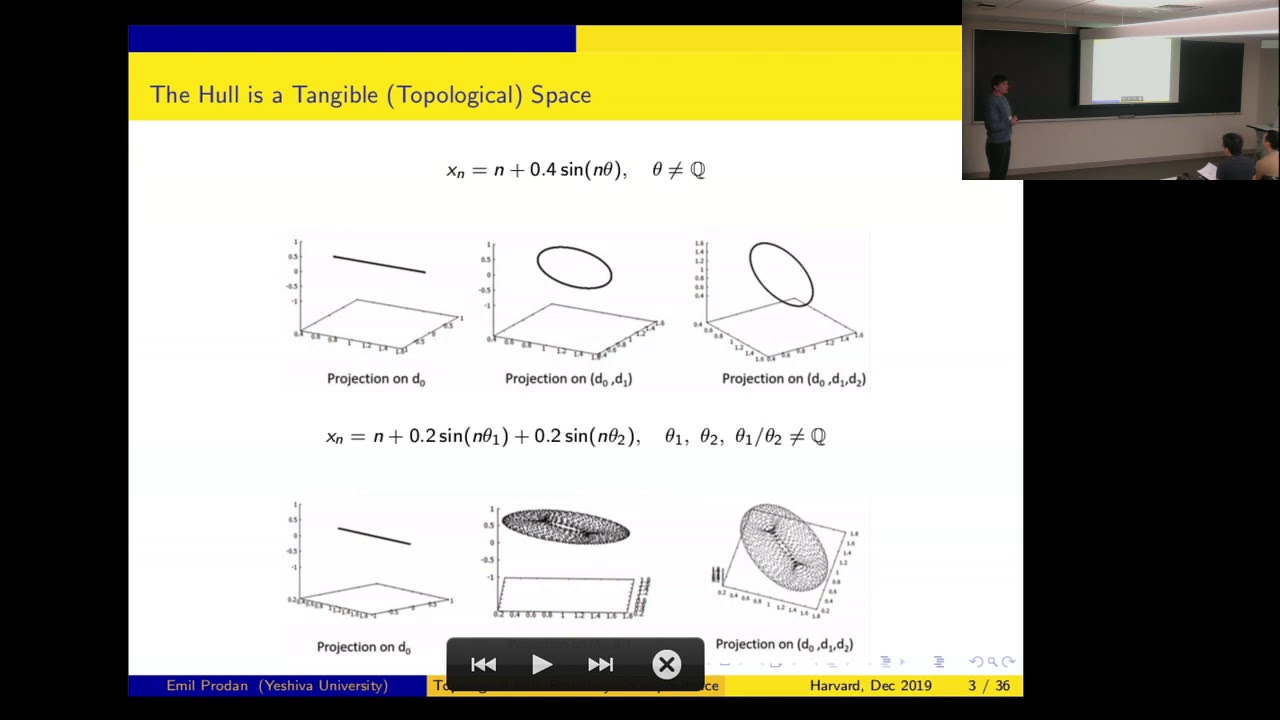
Показать описание
Speaker: Emil Prodan, Yeshiva
Title: Topological Bulk-Boundary Correspondence via K-Theory
Abstract: I will demonstrate how K-Theory becomes one of the finest tools available when it comes to establishing the bulk-boundary correspondence in aperiodic systems. For example, the closely related quasi-periodic and quasi-crystalline systems display the same bulk K-theory but they have a completely opposite edge physics: topological edge spectrum exists for the first class of systems but it is entirely absent for the second class. The K-Theory of the edge and its relation with the bulk explain the differences. I will then demonstrate how the K-Theoretic guidance lead to concrete engineering of many aperiodic meta-materials where topological pumping is possible.
Title: Topological Bulk-Boundary Correspondence via K-Theory
Abstract: I will demonstrate how K-Theory becomes one of the finest tools available when it comes to establishing the bulk-boundary correspondence in aperiodic systems. For example, the closely related quasi-periodic and quasi-crystalline systems display the same bulk K-theory but they have a completely opposite edge physics: topological edge spectrum exists for the first class of systems but it is entirely absent for the second class. The K-Theory of the edge and its relation with the bulk explain the differences. I will then demonstrate how the K-Theoretic guidance lead to concrete engineering of many aperiodic meta-materials where topological pumping is possible.
 1:01:11
1:01:11
 1:02:09
1:02:09
 1:58:25
1:58:25
 0:52:08
0:52:08
 0:32:28
0:32:28
 0:55:39
0:55:39
 0:58:49
0:58:49
 1:12:12
1:12:12
 0:30:03
0:30:03
 1:00:02
1:00:02
 0:08:06
0:08:06
 1:03:15
1:03:15
 1:12:00
1:12:00
 0:38:04
0:38:04
 1:54:37
1:54:37
 0:17:26
0:17:26
 0:40:46
0:40:46
 0:29:18
0:29:18
 0:35:51
0:35:51
 1:18:17
1:18:17
 0:05:47
0:05:47
 0:05:15
0:05:15
 0:49:28
0:49:28
 1:19:40
1:19:40