Introduction to Vector-Valued Functions

preview_player
Показать описание
  1. Math with Dr. Claire
  2. vector calculus
  3. calculus
  4. calculus 3
  5. parameterization
  6. 3D
Рекомендации по теме
Introduction to Vector-Valued 0:05:26

Introduction to Vector-Valued Functions

Introduction to Vector-Valued 0:08:44

Introduction to Vector-Valued Functions

3D Curves and 0:10:07

3D Curves and their Tangents | Intro to Vector-Valued Functions

Introduction to Vector 0:09:45

Introduction to Vector Valued Functions

Introduction to vector-valued 0:16:01

Introduction to vector-valued functions and curves, Multivariable Calculus

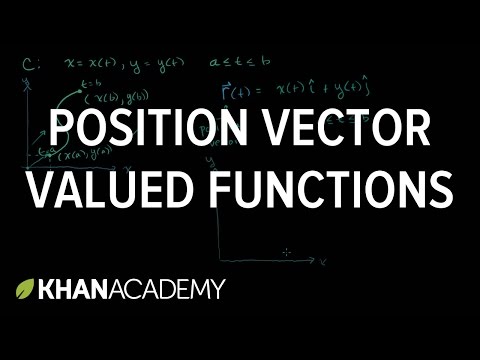
Position vector valued 0:07:45

Position vector valued functions | Multivariable Calculus | Khan Academy

Vector-Valued Functions (Introduction) 0:06:20

Vector-Valued Functions (Introduction)

13.1 Introduction to 0:09:38

13.1 Introduction to Vector Valued Functions

Session B | 1:29:51

Session B | Tuesday morning | TQC 2024

Introduction to Vector-Valued 0:05:39

Introduction to Vector-Valued Functions

Calculus 3 Lecture 2:04:56

Calculus 3 Lecture 12.1: An Introduction To Vector Functions

12.1 : introduction 0:08:06

12.1 : introduction to vector valued function

Introduction to Vector 0:03:11

Introduction to Vector Valued Functions

Introduction to Vector 0:13:22

Introduction to Vector Valued Functions and derivatives

Intro to Vector 0:04:07

Intro to Vector Functions

13.1 Vector functions 0:33:56

13.1 Vector functions | Anas Abu Zahra

Position vector valued 0:07:05

Position vector valued functions | Multivariable Calculus | Khan Academy

Vector-Valued Functions - 0:07:57

Vector-Valued Functions - Introduction: 01. Introduction

12.1 Intro to 0:06:53

12.1 Intro to Vector-Valued Functions

Calculus 3: Lecture 0:56:23

Calculus 3: Lecture 12.1 Vector-Valued Functions

Intro To Vector 0:12:42

Intro To Vector Valued Functions

Introduction to vector-valued 0:01:26

Introduction to vector-valued functions

Introduction to Calculus 0:09:12

Introduction to Calculus with Vector-Valued Functions

Calculus 3 - 0:57:54

Calculus 3 - Intro To Vectors

Комментарии
Автор

This is so cool!! Especially going from 3D to 2D by omitting an axis (the helix to cosine function blew my mind 😯)

AmirJacob_
Автор

You are Amazing. Words don't do justice to how good your explanation is.

ShahFahad-ezcm
Автор

Hey Claire, Im a mechanical engineer and have mathematical problem to solve. Imagine two given spheres anywhere in space with an equal radius. The intersection between them is a circle in space, but i want the curve in parametrized form. My solution is to make a new coordinate system with the origin in the center the circle. The z-axis is facing in the direction of the line between the two centers of the spheres. In this coordinate-system the equation of the circle is simple because the z-component is always zero.

To get to this new coordinate system i first rotatet the original one around the z-axis and then around the new x-axis so it alines with the connection line. Then i get the solution by doing a coordinate-transformation, but the symbolic solution is very very long.

My question is if you could think of a more elegent way of getting a closed symbolic parametrized solution of the intersection circle.
Sadly my mathematical toolbox as a engineer is rather limited and i gues you coulf think of a nicer way to solve this problem.

Greeting from Germany☺️

legoweaponary
Автор

Very well explained..
Which software did you use for the visualisation of the curves?

asmaazhar