filmov
tv
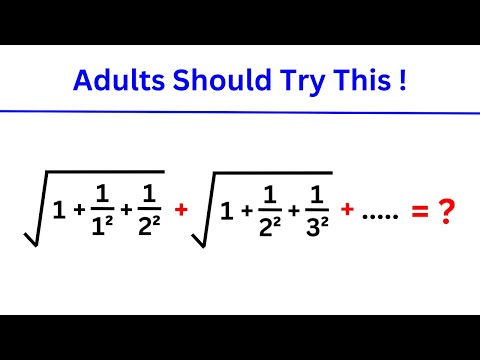
Nice Algebra Problem || Tricky Maths Olympiad Question

Показать описание
Hello my Wonderful family 😍😍😍
Trust you're doing fine 😊.
•If you like this video about Math Olympiad Problem Solving.
~Please like and Subscribe to my channel.
It helps me a lot. Thanks 🙏
•Oxford University Entrance Exam Question
•Harvard University Entrance Examination
•International Math Olympiad
•Math Olympiad Questions
•Mathematics Education
•Math Problem Solving
•Advanced Math Concepts
•Challenging Math Problems
•Algebraic Expressions
•Nice Square Root Math Simplification
•Nice Algebra Simplification
•Nice Radical Simplification
•USA Mathematical Olympiad
•Germany Math Olympiad
•Japanese Math Olympiad
•Pakistan Math Olympiad
•China Math Olympiad
•Russian Math Olympiad
•Indian Math Olympiad
•Australia Math Olympiad
•Thailand junior maths olympiad questions
•How to solve | Math Olympiad
#matholympiad #algebra#math#simplification #exponents#viralmathproblem #howtosolve#mathematics#viral #mathematicslesson#math#maths
#canyousolvethis?#olympiad #mathematics#mathtricks#matholympiadquestion#funofmathematics#algebra#exponential#olympiadalgebra#exponentialequation #howtosolvemaths#education #equations #mathbeast
•SPREAD POSITIVITY ALWAYS ❤️
Subscribe (•‿•)
Trust you're doing fine 😊.
•If you like this video about Math Olympiad Problem Solving.
~Please like and Subscribe to my channel.
It helps me a lot. Thanks 🙏
•Oxford University Entrance Exam Question
•Harvard University Entrance Examination
•International Math Olympiad
•Math Olympiad Questions
•Mathematics Education
•Math Problem Solving
•Advanced Math Concepts
•Challenging Math Problems
•Algebraic Expressions
•Nice Square Root Math Simplification
•Nice Algebra Simplification
•Nice Radical Simplification
•USA Mathematical Olympiad
•Germany Math Olympiad
•Japanese Math Olympiad
•Pakistan Math Olympiad
•China Math Olympiad
•Russian Math Olympiad
•Indian Math Olympiad
•Australia Math Olympiad
•Thailand junior maths olympiad questions
•How to solve | Math Olympiad
#matholympiad #algebra#math#simplification #exponents#viralmathproblem #howtosolve#mathematics#viral #mathematicslesson#math#maths
#canyousolvethis?#olympiad #mathematics#mathtricks#matholympiadquestion#funofmathematics#algebra#exponential#olympiadalgebra#exponentialequation #howtosolvemaths#education #equations #mathbeast
•SPREAD POSITIVITY ALWAYS ❤️
Subscribe (•‿•)
Комментарии
 0:08:26
0:08:26
 0:05:32
0:05:32
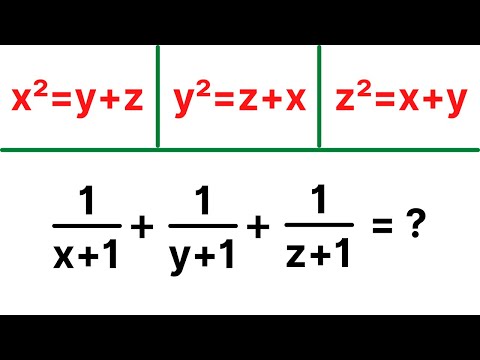 0:03:15
0:03:15
 0:09:18
0:09:18
 0:10:01
0:10:01
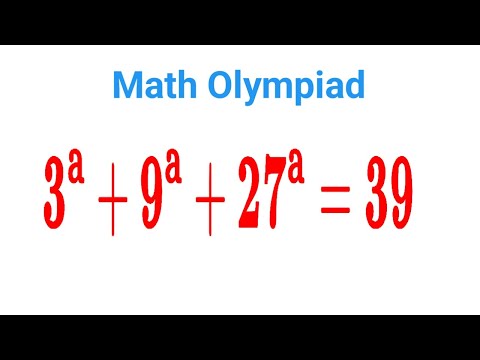 0:07:18
0:07:18
 0:08:16
0:08:16
 0:08:29
0:08:29
 0:08:42
0:08:42
 0:04:38
0:04:38
 0:09:08
0:09:08
 0:09:18
0:09:18
 0:10:58
0:10:58
 0:10:31
0:10:31
 0:02:28
0:02:28
 0:08:08
0:08:08
 0:10:13
0:10:13
 0:00:31
0:00:31
 0:10:24
0:10:24
 0:10:13
0:10:13
 0:00:46
0:00:46
 0:04:15
0:04:15
 0:01:00
0:01:00
 0:09:33
0:09:33