filmov
tv
LINEAR APPROXIMATION TO ESTIMATE SQRT(24) - how to use linearization with no f(x) or a (Part 3)
Показать описание
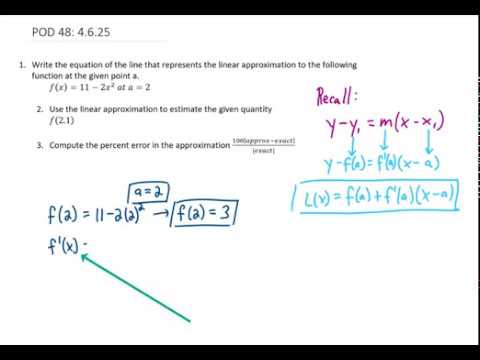
Linearization or the linear approximation of a function can be used to estimate the output of a function when finding its exact value is difficult. This has a handful of different useful applications. In this video I'll show you how to find the linearization of a function at a point and how to apply it to estimate more challenging values near that point like sqrt(24).
In order to find the linearization of a given function at a given point, it will be very similar to finding the equation of a tangent line. With linearization, we can use the formula for the linearization of a function, denoted L(x).
In this video I'll show you how to find the linearization to approximate sqrt(24) when no f(x) or a has been given. This means that we will be coming up with a linear approximation based on a function that we have to come up with knowing the value we want to approximate. Then we will use this linear approximation to approximate the value of sqrt(24).
READ MORE
WATCH NEXT
YOU MIGHT ALSO BE INTERESTED IN...
Some links in this description may be affiliate links meaning I would get a small commission for your purchase at no additional cost to you.
In order to find the linearization of a given function at a given point, it will be very similar to finding the equation of a tangent line. With linearization, we can use the formula for the linearization of a function, denoted L(x).
In this video I'll show you how to find the linearization to approximate sqrt(24) when no f(x) or a has been given. This means that we will be coming up with a linear approximation based on a function that we have to come up with knowing the value we want to approximate. Then we will use this linear approximation to approximate the value of sqrt(24).
READ MORE
WATCH NEXT
YOU MIGHT ALSO BE INTERESTED IN...
Some links in this description may be affiliate links meaning I would get a small commission for your purchase at no additional cost to you.
0:06:03
LINEAR APPROXIMATION TO ESTIMATE SQRT(24) - how to use linearization with no f(x) or a (Part 3)
0:07:03
Use a linear approximation to estimate the given number
0:06:48
Linear Approximation to Estimate A Number | Estimate Sqrt and Ln with Calculus
0:04:22
Use linear approximation to estimate the following
0:00:38
Linear Approximation in a Snap!
0:00:33
Use linear approximation to estimate sqrt root of 4.1
0:08:15
Use a linear approximation to estimate the given number
0:05:48
How to Use Linear Approximation to Estimate the Value of 1 Divided by the Square Root of 65
0:07:17
LINEAR APPROXIMATION AND DIFFERENTIALS TO APPROXIMATE SQRT(0.9) - how to use linearization (Part 2)
0:00:34
Human Calculator Solves World’s Longest Math Problem #shorts
0:05:03
Use linear approximation to estimate - Differential Calculus
0:03:54
Use Linear Approximation to Estimate ln(1.01) The Easy Way!
0:04:15
Use a linear approximation to estimate xxxx
0:06:06
How to Use Linear Approximations to Estimate the Value of the Fifth Root of 0.95
0:07:00
use local linear approximation, no calculator!
0:09:49
Linear Approximations | Using Tangent Lines to Approximate Functions
0:00:50
5 simple unsolvable equations
0:03:48
Linear Approximation 6: Approximating sqrt(25.1)
0:03:06
Math 1A 3.9.16 Use a linear approximation (or differentials) to estimate the given number.
0:22:13
Linear Approximation- (Calc1-Examples#26)
0:28:24
4.2 Linear Approximations and Differentials
0:10:35
Approximation Using Linearization (Calculus)
0:00:32
Cheating in exams😏!?
0:04:01
Calc 1 POD #48 Linearizations
Комментарии