3D Trigonometry | Trigonometry | Maths | FuseSchool

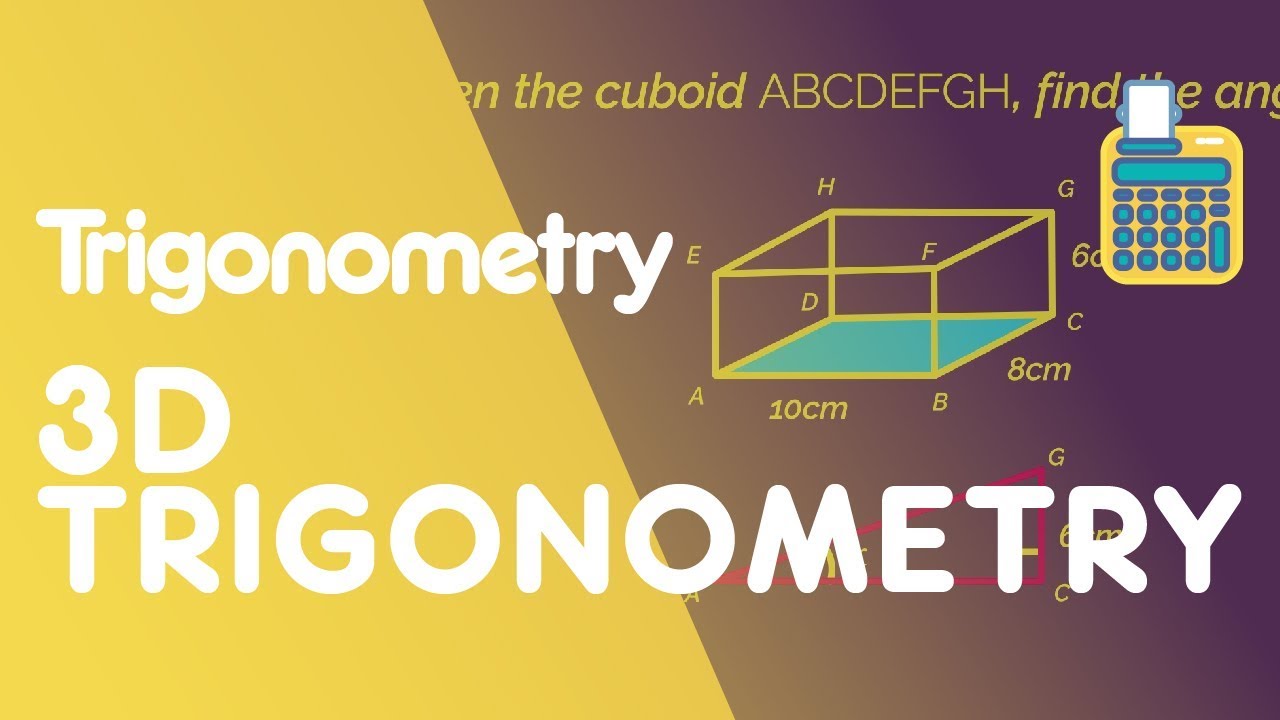
Trigonometry in 3D can look really scary… but if we split it down into 2D problems, then there’s nothing new to learn.

Always look for right angle triangles inside the 3D shapes, so find where a horizontal plane meets a vertical plane.

Then we can use Pythagoras and SOHCAHTOA.

Start by drawing your 2D right angle triangle… CAG

We know it’s a right angle, because CG is a vertical line and CA is a horizontal plane.

Before we can find angle x, we need to find the length AC.

To find the length of AC, we need to go back to our first diagram…

And draw on the line AC

We can easily find the length of AC using Pythagoras on this triangle

It is better to keep y as root 164 because this is the most accurate answer. You need to be careful to not round any values too early on in these questions.

So now that we know the length of AC, we can go back to the actual question.

And are now able to find the size of angle x using SOHCAHTOA 25.1 degrees

We just need to take these questions step by step, always searching for those right angle triangles.

Let’s have a look at a square based pyramid.

As always, start by looking for right angle triangles. Right angles are only found between vertical and horizontal lines…

So we need to drop a vertical from T down to the centre of the horizontal base.

Now we have a right angle triangle, with a base of 4… because it’s half the length of AB.
And a height of 10.

We have a right angle triangle, so we can use SOHCAHTOA to find angle x. Pause the video, find angle x and click play when you’re ready.

Did you get 68.2 degrees?

What if the question had asked for angle ACT? What right angle triangle would you have used? See if you can find ACT. Pause the video, give it a go and click play when
you’re ready.

Did you get 60.5 degrees? Did you use this right angle triangle?

So that’s all there is to 3D trigonometry. You just need to hunt down those right angle triangles - so always look for a vertical line and a horizontal line or plane.

Quite often these are two step problems, once using Pythagoras and once using SOHCAHTOA.

3D trigonometry does actually have lots of real life applications… if you’re ever trying to work out a distance in 3 dimensions… like an architect designing a house… or a pilot in a plane, chances are 3D trigonometry is involved.

If you have any questions or want further help, comment below.

CREDITS
Animation & Design: Waldi Apollis
Narration: Lucy Billings
Script: Lucy Billings

SUPPORT US ON PATREON

SUBSCRIBE to the FuseSchool YouTube channel for many more educational videos. Our teachers and animators come together to make fun & easy-to-understand videos in Chemistry, Biology, Physics, Maths & ICT.

These videos can be used in a flipped classroom model or as a revision aid.

[RiseTrendUK]

Hey Fuseschool, thank you for the idea that I need for my GCSE. It's a very high level and I am taking Higher Tier; it's hard but if I practice, I will make to efficient.

[sridash]

I don't want to be the first to comment