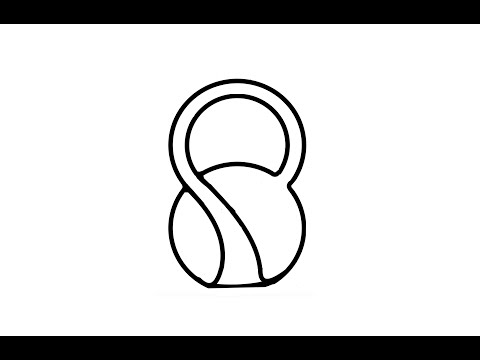
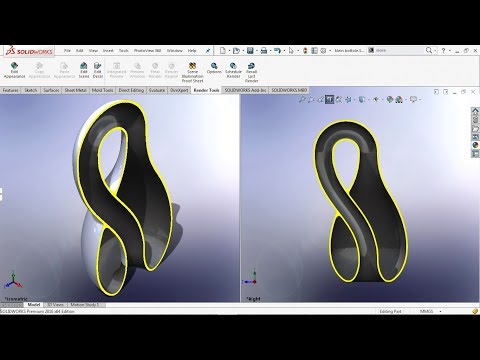
Solid Klein Bottles

Simple closed geodesics in a q-manifold are by construction solid q-manifolds with boundary obtained by stacking together facets using the geodesic map T. It is of interest to know what the topology of the geodesic is within the manifold but this is not our interest here. We look at the geodesic itself as an object. (This might surprise since in the continuum, the object "simple closed geodesic" is just a circle, a one dimensional connected manifold. Also using naive notions of geodesics in graphs one just has a cyclic graph without any interesting structure. But geodesics as defined by us earlier have more structure,l they are a path in the frame bundle. There can be a nontrivial permutation achieved when returning back. And this happens in general like in a 2-manifold that is non-orientable there can be geodesics that are Moebius strips. In two dimensions, there are only two possibilities. Cylinder or Moebius strip. In three dimension, since the boundary of a geodesic tube is a flat 2-manifold, we either have a Klein bottle or a torus. Both are possible. The geodesic itself is then a so called "solid Klein bottle". Also here, a solid Klein bottle is topologically different from a solid torus and quadratic cohomology can distinguish it similarly as we have seen in 2016 already that quadratic cohomology can distinguish the cylinder from the Moebius strip. Simplicial cohomology of course does not work because simplicial cohomology is even a homotopy invariant. We need finer notions to distinguish the Moebius strip from the cylinder. The interesting question now is whether there is anything more interesting happening in higher dimensions. We can not go far with quadratic cohomology as the computational task becomes large in higher dimensions. So far we see just the dichotomy of quadratic Betti numbers (0,0,,..0.,1,1,0,.. 0) and (0,0,0....,0) as in the two dimensional case. Related is the question to take the complement of the interior of the geodesic which is the complement of a knot in higher dimensions and study this cohomology. Our Fusion inquality discussed ad nauseam last year sheds light between the cohomology of an open set and the cohomology of its closed complement.

For the 2016 result, here is the case study from 2016
I had called quadratic cohomology also Wu cohomology or interaction cohomology.