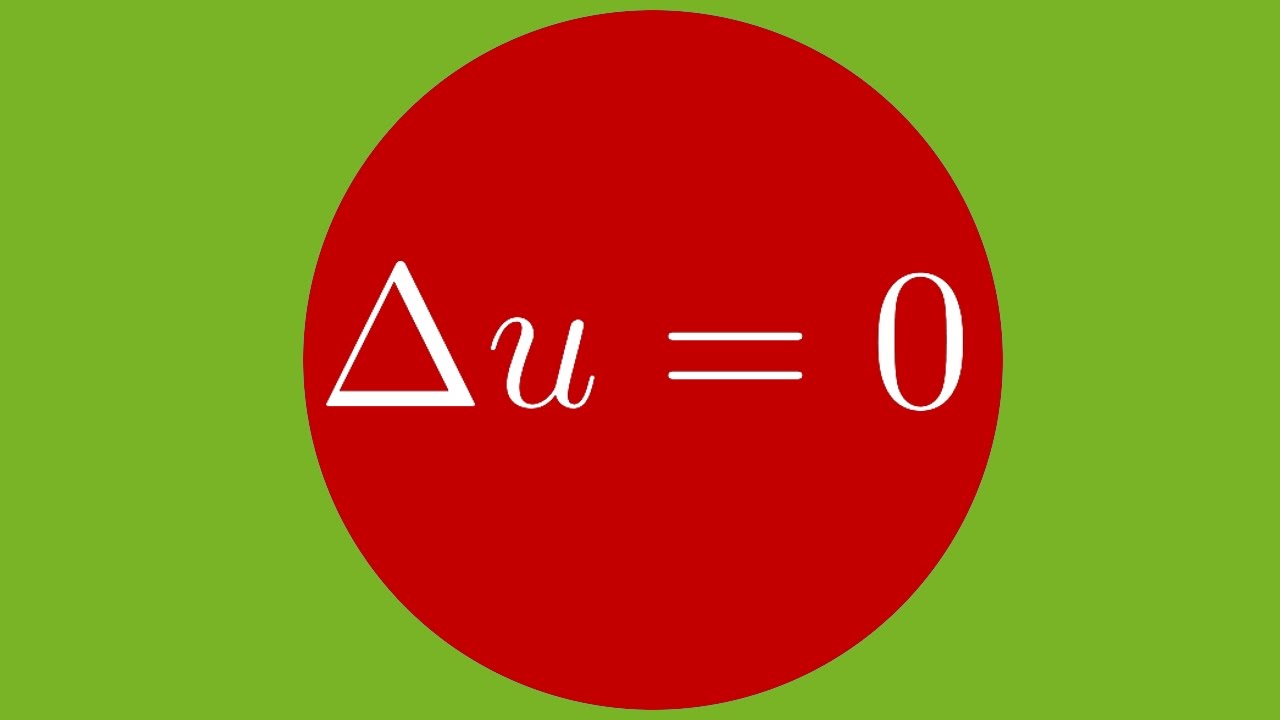
Laplace Equation on the Unit Disk

preview_player
Показать описание
  1. MathTheBeautiful
  2. Partial Differential Equations
  3. Laplacian
  4. Eigenvalues
  5. Diffential Operator
  6. Laplace Equation
Рекомендации по теме
Laplace Equation on 0:22:50

Laplace Equation on the Unit Disk

How to solve 0:00:46

How to solve differential equations

Differential Equations | 0:04:44

Differential Equations | Laplace Transform of a Piecewise Function

ch11 4. Poisson 0:02:50

ch11 4. Poisson equation on a unit square. Wen Shen

Laplace Transform of 0:06:27

Laplace Transform of a Piecewise Function (Unit Step Function)

Partial Differential Equation 0:39:45

Partial Differential Equation with Dirichlet Boundary Conditions (With Example)

Solve differential equation 0:07:05

Solve differential equation with Laplace Transform involving unit step function

Fourier Series Solution 0:14:04

Fourier Series Solution of Laplace's Equation

laplace of integral 0:04:45

laplace of integral function | Laplace Transforms of Integrals | LAPLACE TRANSFORM | MATHEMATICS

Separation of Variables 0:07:10

Separation of Variables - Laplace Eq Part 1

ch11 3. Laplace 0:03:54

ch11 3. Laplace equation with non-homogeneous Dirichlet BCs. Wen Shen

Derivation of Poisson's 0:14:30

Derivation of Poisson's Formula for of Laplace's Equation on the Unit Disk: Complex Fourie...

ch11 5. Laplace 0:06:47

ch11 5. Laplace equation with Neumann boundary condition. Wen Shen

LAPLACE EQUATION WITH 0:07:18

LAPLACE EQUATION WITH EXAMPLE PROBLEM ||M3||OU EDUCATION

Laplace PDE on 0:35:14

Laplace PDE on circular domain solved in polar coordinates

Intro to the 0:12:05

Intro to the Laplace Transform & Three Examples

Laplace Equation on 0:25:45

Laplace Equation on an Annulus, Example #4 [MATH-241] [PDEs]

Poisson's Equation and 0:09:59

Poisson's Equation and Laplace's Equation

Solving Laplace Equation 0:43:19

Solving Laplace Equation in spherical coordinates part 1

LAPLACE AND POISSON'S 0:03:14

LAPLACE AND POISSON'S EQUATIONS (derivation)

Laplace Equation 0:19:35

Laplace Equation

Derivation and Solution 0:33:40

Derivation and Solution of Laplace’s Equation

Laplace Transforms with 0:12:24

Laplace Transforms with Unit Step Functions

Laplace's equation: Why 0:15:33

Laplace's equation: Why are solutions unique?

Комментарии
Автор

Professor MathTheBeautiful, thank you for a powerful analysis of Laplace Equation on the Unit Disk in Partial Differential Equations. This is an error free video/lecture on YouTube TV.

georgesadler
Автор

Please keep sharing your lectures. Just beautiful. Thank you

darrenpeck
Автор

Can you please give a link to the lesson in which you derived the Laplacian in polar coordinates?

princeardalan
Автор

1. How can we tell if a PDE is separable before we actually try the separation technique? (which may fail if the equation is not separable, and we may waste a whole lotta time by trying to do the impossible :q ) Is there any rule for when the equation is separable?
2. After separating the variables, what is the justification behind the assumption that both sides of the equation must be equal to a constant? How do you know that this is true?
3. Can you also show how to solve the same problem in rectangular coordinates, for comparison? (just to show that polar coordinates are really better to go with in this case, and that it is still possible to solve it in rectangular coordinates, just cumbersome to do so)

Oh, and it would be nice to put the link in the description to the derivation of the Laplacian in polar coordinates. You said in the video that it's been derived in another lesson, but you didn't say which one.

scitwi
Автор

I am highly thankful to you sir....now i can solve my assignment

khadijaijaz
Автор

The radial could be a Cauchy-Euler equidimensional differential equation, que no?

CARLOSSAA-yf
Автор

How do you solve the differential equation in R(r) without assuming that C_n r^{2} + D_n r^{-2} is a solution?

koetje