Cauchy-Schwarz's Inequality | Schwarz's | Inner Product Space | MAKAUT PYQ | Linear Algebra

preview_player
Показать описание


This video lecture of Cauchy-Schwarz's Inequality from Inner Product Space will help Engineering and Basic Science students to understand following topic of Mathematics:
1. What is a Inner Product.
2. How to define Norm.
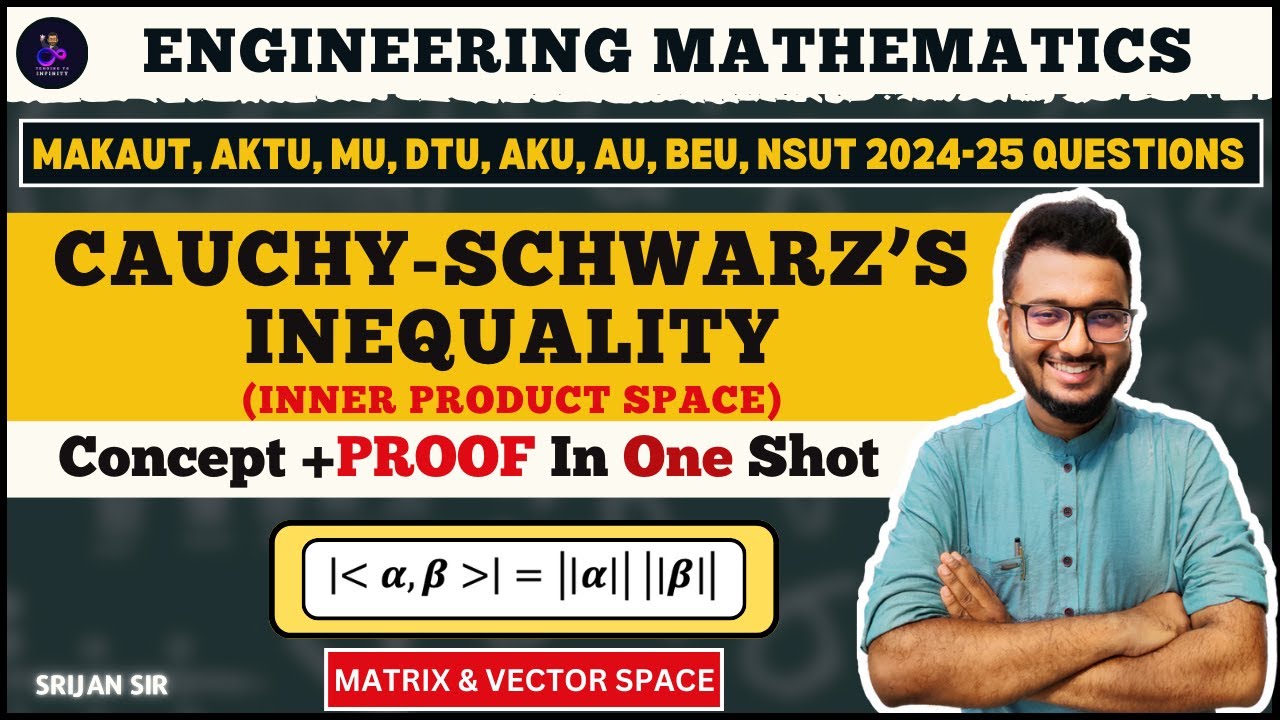
3. Statement of Cauchy-Schwarz's Inequality
4. Statement of Schwarz's inequality
5. Statement of Schwarz's theorem
6. Proof of Cauchy-Schwarz's Inequality
7. Solve Previous year question solution.

#cauchyschwarzs #schwarz_ineqality #innerproductspace #norm #norm_of_vectors #matrix #innerproduct #linearalgebra #engineeringmathematics #makaut #bscmaths #BSM101 #BSM102 #matrix #organiser #organiser_solution #makautsemester #makaut_organiser_solution #definition #definition_of_vector_space #internal_operation #euclidean #euclidean_space #makaut_3rd_sem #makaut_3rd_sem_maths #makaut_3rd_sem_aiml #makaut_3rd_sem_aiml_maths

Link of Playlist of Calculus (Integration):

Do Like & Share this Video with your Friends. If you are watching for the first time then Subscribe to our Channel and stay updated for more videos around Mathematics.

Thanks For Watching My Video
Like, Share & Subscribe

Query Solved :
  1. Tending to Infinity
  2. cauchy schwarz inequality
  3. inequality
  4. cauchy schwarz inequality proof
  5. cauchy schwarz inequality example
  6. cauchy schwarz inequality jee
Рекомендации по теме
Cauchy Bunyakovsky Schwarz 0:01:47

Cauchy Bunyakovsky Schwarz Inequality I (visual proof)

Cauchy-Bunyakovsky-Schwarz Inequality II 0:02:05

Cauchy-Bunyakovsky-Schwarz Inequality II (visual proof)

Proof of the 0:16:55

Proof of the Cauchy-Schwarz inequality | Vectors and spaces | Linear Algebra | Khan Academy

Basic Cauchy-Schwarz Inequality 0:02:19

Basic Cauchy-Schwarz Inequality - Linear Algebra Made Easy (2016)

A Visual Proof 0:05:35

A Visual Proof of the Cauchy Schwarz Inequality

02.Exercises Proof of 0:05:17

02.Exercises Proof of the Cauchy-Schwarz Inequality

SCHWARZ'S INEQUALITY PROOF 0:11:07

SCHWARZ'S INEQUALITY PROOF || SCHWARZ'S INEQUALITY IN LINEAR ALGEBRA

Cauchy-Schwarz Inequality | 0:28:10

Cauchy-Schwarz Inequality | Mathematical Olympiad Course, Part IV

Cauchy Schwarz Inequality 0:10:16

Cauchy Schwarz Inequality | Applications to Problems, and When Equality Occurs

The Cauchy-Schwarz Inequality 0:15:07

The Cauchy-Schwarz Inequality Part 1

Cauchy Schwarz and 0:12:47

Cauchy Schwarz and what to DO with it (corrected) // An Introduction to Real Analysis

The Cauchy Schwarz 0:05:45

The Cauchy Schwarz Inequality via Lagrange Multipliers

Cauchy-Schwarz Inequality: Math 0:00:36

Cauchy-Schwarz Inequality: Math Notes for the Curious

Cauchy Schwarz Proof 0:09:19

Cauchy Schwarz Proof

Math 521 #151: 0:07:06

Math 521 #151: Minimum value using Cauchy–Bunyakovsky–Schwarz Inequality

Cauchy-Schwarz: Proof using 0:07:59

Cauchy-Schwarz: Proof using Jensen's Inequality

Solution 82: Simple 0:10:13

Solution 82: Simple Bashing & Cauchy-Schwarz 'Equality'

The Cauchy-Schwarz Inequality 0:10:17

The Cauchy-Schwarz Inequality

Cauchy-Schwarz Inequality: Method 0:14:55

Cauchy-Schwarz Inequality: Method 1 || Theory of Metric Spaces

Cauchy-Schwarz Inequality (Must 0:04:42

Cauchy-Schwarz Inequality (Must For Olympiads) | Cauchy-Schwarz Inequality Explained By Aman Sir

The Cauchy-Schwarz inequality 0:04:02

The Cauchy-Schwarz inequality saves the situation

The Cauchy-Schwarz Inequality 2:15:20

The Cauchy-Schwarz Inequality

AM-GM Inequality via 0:01:00

AM-GM Inequality via negative space

Calculus III: The 0:08:00

Calculus III: The Dot Product (Level 12 of 12) | Cauchy-Schwarz, Triangle Inequality

Комментарии
Автор

15:33 sir, if the vectors are linearly independent then both the values of c1 and c2 should be equal to 0 and hence the value of the equation should be equal to theta, right? Sir, i have watched all the previous lectures but i can't understand this.

prapti
Автор

11:00 bracket mein therefore ||β|| = √<β, β> hoga na sir?

Study-