filmov
tv
Probability and Infinite Geometric Series Example - Coin Toss Question

Показать описание
#smartalexcoaching #probability #infiniteseries
This VDO looks at the approach to solving probability question that results in nn infinite geometric series.
At Smart Alex Coaching, we provide mathematics tutoring for students from primary to high school both online and face-to-face.
These VDOs are created to complement our students' lessons but also to help anyone wanting to learn maths no matter where they are in the world.
Please support our mission by clicking Like, Comment, Share and Subscribe.
VDOs are uploaded weekly with various topics, different tips and tricks to make maths easier to understand.
#hsc #sumtoinfinity #limitingsum #advancedmaths
This VDO looks at the approach to solving probability question that results in nn infinite geometric series.
At Smart Alex Coaching, we provide mathematics tutoring for students from primary to high school both online and face-to-face.
These VDOs are created to complement our students' lessons but also to help anyone wanting to learn maths no matter where they are in the world.
Please support our mission by clicking Like, Comment, Share and Subscribe.
VDOs are uploaded weekly with various topics, different tips and tricks to make maths easier to understand.
#hsc #sumtoinfinity #limitingsum #advancedmaths
Probability and Infinite Geometric Series Example - Coin Toss Question
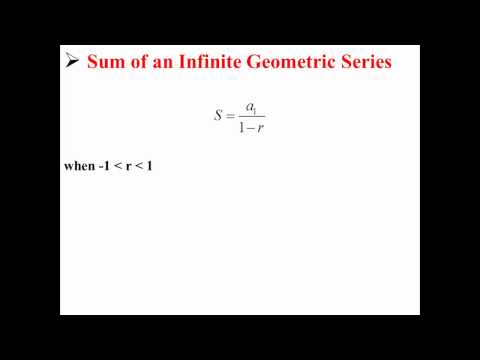
Finding The Sum of an Infinite Geometric Series
Geometric Series Video 3 - Connection to Probability
2017 AMC 10 A Problem 18 (Probability, Infinite Geometric Series)
Infinite Geometric Series
Applications of Probability Sum to infinity of a Geometric Series
Geometric Series | Convergence, Derivation, and Example
WJEC S1 Probability Sum to infinity
Infinite Series - Numberphile
Infinite Series and Probability
Making Probability Mathematical | Infinite Series
Computing an infinite series
Another Infinite Geometric Series
Video 1 11 A 9 5 Infinite Geometric Series
Conditional Probability Question of Infinite Geometric series Part B
Introduction to Infinite Geometric Series
SOA Exam P Question 14 | Infinite Geometric Series
S01.6 The Geometric Series
Algebra 2 Section 11 5 Infinite Geometric Series
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Applications of Probability Sum to infinity of a Geometric Series
Arithmetico-Geometric Sequences
Working with Infinite Geometric Series
F20 Probability Lecture 12: Probability and Infinite Series
Комментарии
 0:09:29
0:09:29
 0:19:50
0:19:50
 0:13:27
0:13:27
 0:02:16
0:02:16
 0:01:57
0:01:57
 0:05:10
0:05:10
 0:06:28
0:06:28
 0:06:03
0:06:03
 0:09:31
0:09:31
 0:04:07
0:04:07
 0:14:21
0:14:21
 0:04:49
0:04:49
 0:00:37
0:00:37
 0:21:36
0:21:36
 0:18:51
0:18:51
 0:08:09
0:08:09
 0:03:08
0:03:08
 0:04:07
0:04:07
 0:08:23
0:08:23
 0:00:38
0:00:38
 0:05:10
0:05:10
 0:12:31
0:12:31
 0:00:44
0:00:44
 1:35:48
1:35:48