filmov
tv
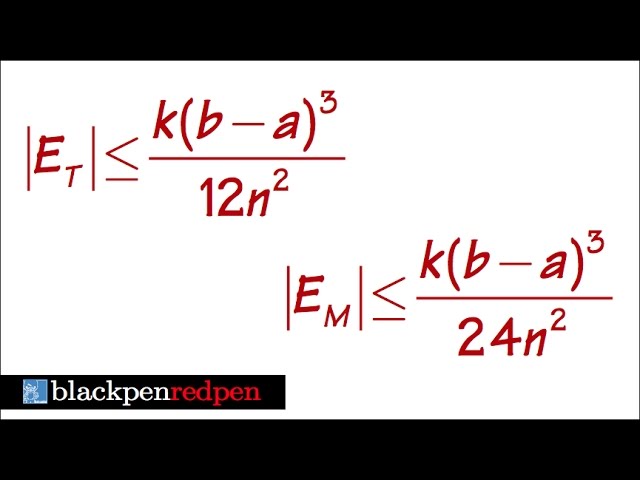
Error Bound Formulas for midpoint and trapezoid rules, sect7.7#19b
Показать описание
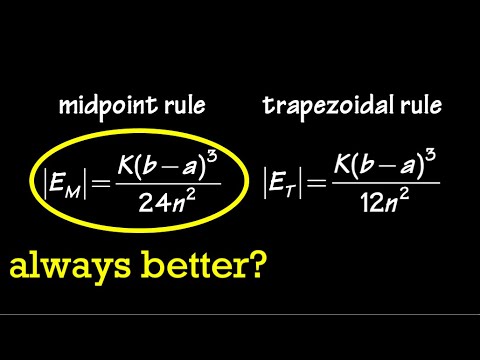
Error bound formula for midpoint rule,
Error bound formula for the trapezoid rule,
approximate integrals,
how to find the K,
blackpenredpen
Error bound formula for the trapezoid rule,
approximate integrals,
how to find the K,
blackpenredpen
Error Bound Formulas for midpoint and trapezoid rules, sect7.7#19b
Midpoint Rule - Error Bound Example 1
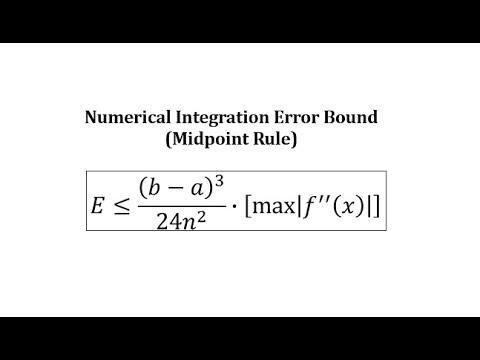
Numerical Integration Error Bound (Midpoint Rule)
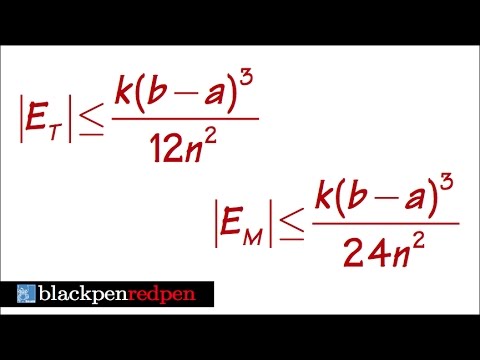
Error bound formulas for trapezoid and midpoint rules
Midpoint Rule - Error Bound Example 2
Trapezoid Rule Error - Numerical Integration Approximation
Midpoint Rule - Error Bound Example 3
Approximate Integration-Finding K in an error bound formula problem II-Lesson-15
Simpson's Rule - Error Bound Example 1
Error Bound in Midpoint Approximation
Approximate Integration: Midpoint Rule Error Bound: Proof
Trapezoidal Rule - Error Bound Example 1
Simpson's Rule Error - Numerical Integration Approximation
Error Bounds of Midpoint and Trapezoidal Rules
Simpson's Rule | Error Bound | Explained through an example
Midpoint rule error bounds
Error Estimates (Midpoint Rule, Trapezoid Rule, Simpson's Rule)
Approximate Integration-Why Error Bound Formula-Lesson-12
Calculus 2: Approximate Integration | Midpoint, Trapezoidal & Simpson’s Rule | Math with Profess...
Trapezoidal Rule - Error Bound Example 2
Approximate Integration-Finding K in an error bound formula problem I-Lesson-14
Approximate integration Error bound problem 1
Approximating an Integral using the Midpoint Rule and Estimating Its Error
Can the trapezoidal rule be better than the midpoint rule for approximating an integral?
Комментарии
 0:13:52
0:13:52
 0:07:28
0:07:28
 0:03:16
0:03:16
 0:09:31
0:09:31
 0:05:25
0:05:25
 0:05:18
0:05:18
 0:11:15
0:11:15
 0:15:54
0:15:54
 0:08:56
0:08:56
 0:15:12
0:15:12
 0:45:31
0:45:31
 0:08:13
0:08:13
 0:06:05
0:06:05
 0:10:37
0:10:37
 0:10:34
0:10:34
 0:02:38
0:02:38
 0:09:37
0:09:37
 0:08:04
0:08:04
 0:55:59
0:55:59
 0:12:22
0:12:22
 0:16:04
0:16:04
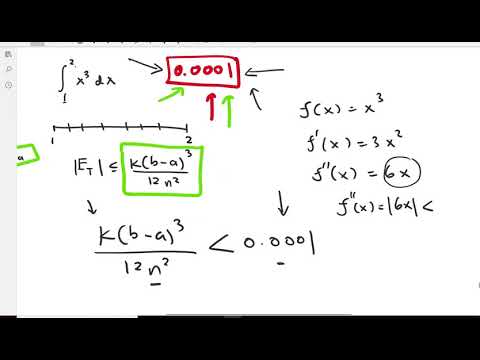 0:10:44
0:10:44
 0:07:53
0:07:53
 0:06:42
0:06:42