filmov
tv
Evaluation Homomorphism for Polynomial Rings Part 3
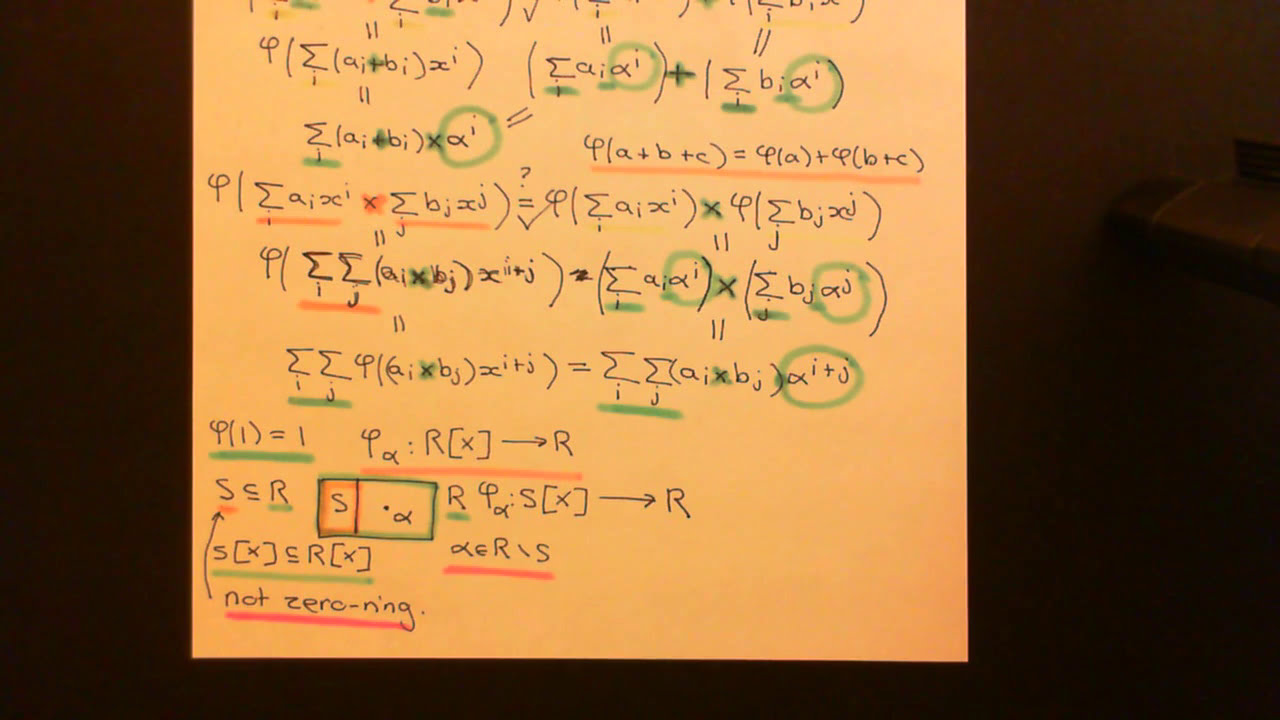
Показать описание
In this video we discuss the evaluation homomorphism applied to polynomial rings.
Evaluation Homomorphism for Polynomial Rings Part 1
Evaluation Homomorphism for Polynomial Rings Part 2
Evaluation Homomorphism for Polynomial Rings Part 3
79 The evaluation homomorphism
Commutative Algebra 13, Polynomial Ring Homomorphisms
Evaluating Polynomials at Polynomials Part 1
80 Kernel and image of the evaluation homomorphism
Evaluating Polynomials at Polynomials Part 2
Example on Evaluation Homomorphism
Use Evaluation Homomorphism ℤ[x] → ℤ by f(x) ↦ f(0) to Show <x> is Prime but Not Maximal in ℤ[x]...
FLOW The Evaluation Homomorphism
Homomorphisms on Polynomial Coefficients (Algebra 3: Lecture 9 Video 1)
Lec evaluation homomorphism theorem and proof ,kernel of evaluation homomorphism ,ring theory
Abstract Algebra | Ring homomorphisms
Theorem 22.4_ The evaluation homomorphism for field theory_ Abstract Algebra
Abstract Algeba: L25, ring homomorphism, 11-4-16
Theorem :- Evaluation Homomorphism
Algebra #3: Polynomial Rings and Fancy Things
Abstract Algebra: decomposing rings, polynomial foundations, 11-13-17
RingHomomorphismsII
Ring Homomorphisms and Isomorphisms -- Abstract Algebra 21
FLOW Quotients of Polynomial Rings
03 Polynomial Rings
Ring homomorphism
Комментарии
 0:22:41
0:22:41
 0:09:11
0:09:11
 0:12:47
0:12:47
 0:17:51
0:17:51
 0:04:26
0:04:26
 0:26:04
0:26:04
 0:06:44
0:06:44
 0:11:41
0:11:41
 0:06:14
0:06:14
 0:09:03
0:09:03
 0:11:12
0:11:12
 0:20:13
0:20:13
 0:18:02
0:18:02
 0:20:01
0:20:01
 0:06:28
0:06:28
 0:52:21
0:52:21
 0:08:09
0:08:09
 0:17:30
0:17:30
 0:50:47
0:50:47
 0:08:43
0:08:43
 1:02:52
1:02:52
 0:15:08
0:15:08
 0:03:02
0:03:02
 0:12:31
0:12:31