filmov
tv
The Linearity of Differentiation

Показать описание
In this video we prove that if f and g are both real valued functions which are differentiable at a point p, then the function f+g is also differentiable at p and has derivative f’+g’ and the function cf (where c is a constant multiple) is differentiable at p with derivative cf’.
These results taken together mean that any linear combination of two differentiable functions will be differentiable and the derivative of the linear combination will be the linear combination of the derivatives. This fact is known as the linearity of differentiation.
These results taken together mean that any linear combination of two differentiable functions will be differentiable and the derivative of the linear combination will be the linear combination of the derivatives. This fact is known as the linearity of differentiation.
 0:04:26
0:04:26
 0:05:43
0:05:43
 0:00:58
0:00:58
 0:06:30
0:06:30
 0:07:47
0:07:47
 0:32:46
0:32:46
 0:03:29
0:03:29
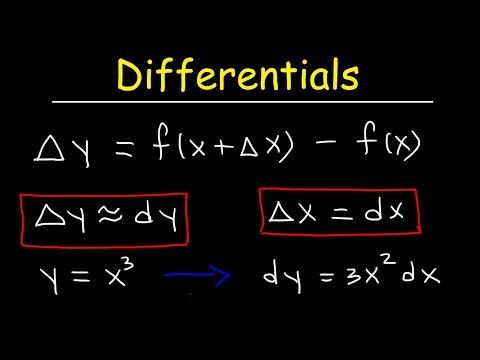 0:10:13
0:10:13
 0:59:51
0:59:51
 0:27:14
0:27:14
 0:01:45
0:01:45
 0:04:02
0:04:02
 0:01:57
0:01:57
 0:20:18
0:20:18
 0:08:40
0:08:40
 0:22:28
0:22:28
 0:16:37
0:16:37
 0:03:41
0:03:41
 0:08:49
0:08:49
 0:20:34
0:20:34
 0:04:37
0:04:37
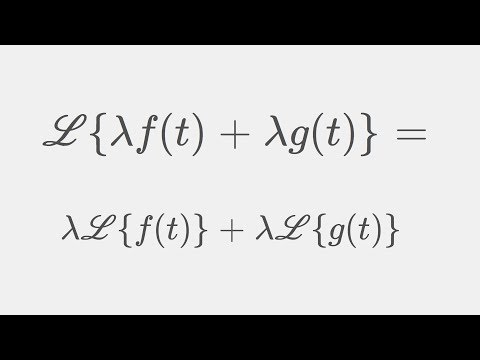 0:05:40
0:05:40
 0:06:57
0:06:57
 0:13:43
0:13:43