filmov
tv
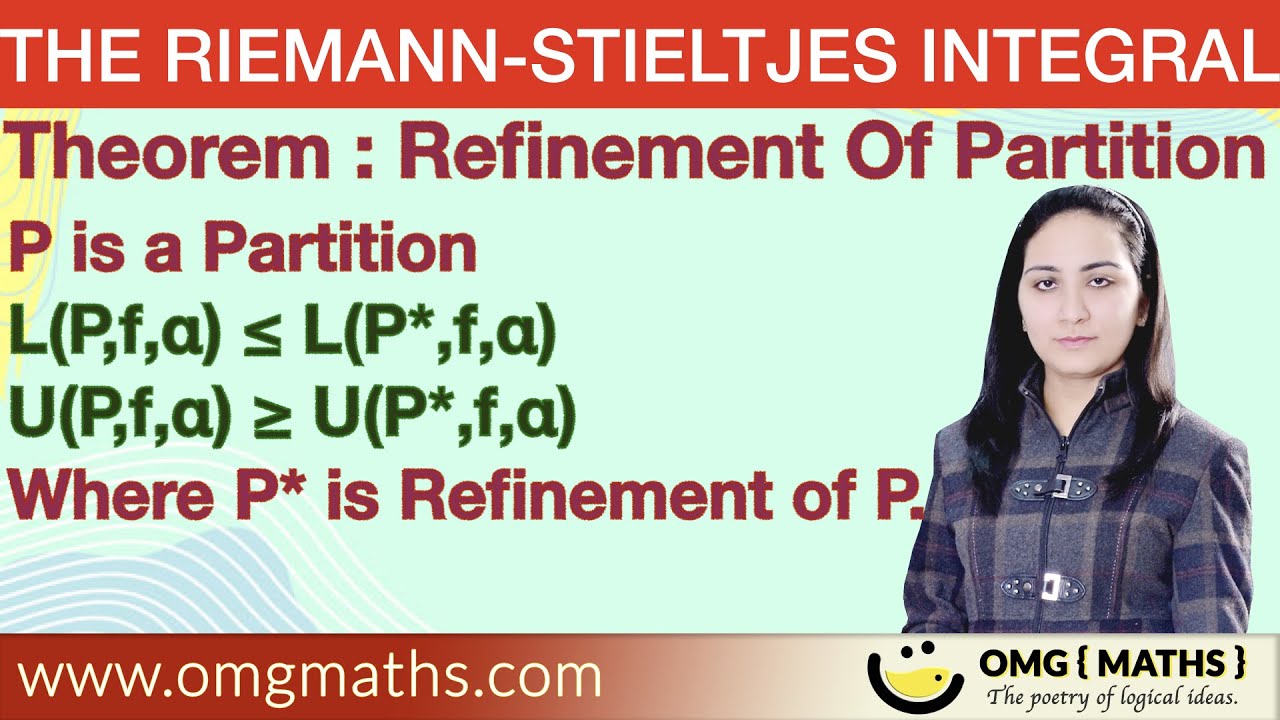
Refinement of Partition | Theorem | The Riemann Stieltjes sum | Effects of refinement on r-s sums
Показать описание
Theorem of Refinement of Partition | Refinement of partition and its effect on Riemann Stieltjes sum | Riemann stieltjes sums | Lower Reimann Stieltjes Sum | Upper Riemann Stieltjes Sum | The R-S Integral | Real analysis | OMG Maths | Classes By Cheena Banga. | #omgmaths
Pdf link:
****The Riemann-Stieltjes Integral****
****Real Analysis playlist****
useful for Msc | BSC | NET | NBHM | LPU | DU | IIT JAM | TIFR
Other topics covered in playlist:
The Riemann-Stieltjes Integral
The R-S Integral
Limit and Continuity
Limit Inferior and limit superior definition and theorems
Cauchy Sequence in Compact Metric Space is Convergent
nth tail of sequence
Tail of a sequence
P Series Test for convergence of series
Limit Comparison Test for Convergence
Cantor Intersection Theorem
E is a subset of metric space X then Diameter of closure of E is equal to Diameter of E
Cauchy First theorem on limits
Bolzano Weierstrass Theorem
Every bounded sequence has a convergent sub sequence
Every Cauchy sequence is a Bounded sequence
Every convergent Sequence is cauchy sequence
Cauchy Sequence
Cauchy Sequence Definition
Cauchy Sequence theorems
Sub sequence of a sequence
Algebraic Properties of Limits
Algebra of limit of sequence
Properties of limit
limit laws of sequence
sandwich theorem
squeeze theorem
Sequence and series
real sequence
range of sequence
constant sequence
uniqueness theorem
Sequences in metric space
limit of sequence
Convergent sequence
Every connected subset of R is an interval
The Real line R is connected
Every interval is connected
In R, intervals and only intervals are connected.
A subset E of R is connected iff E is an interval
compactness in Real Analysis
Connectedness in Real Analysis
Compactness in topology
Connectedness in topology
compactness
connectedness
theorems of compactness
theorems of connectedness
Heine-Borel theorem
Closed Set | definition | theorems
set is closed iff its complement is open
Bolzano weierstrass theorem : Every infinite bounded subset of R has a limit point.
Definition of Neighbourhood of a point
Definition of Open set
infinite intersection of open sets need not to be open
Union of two NBDS is NBD
Intersection of NBDS is NBD
Superset of a NBD is also a NBD
Every Open interval (a,b) is neighbourhood of each of its points.
Closed interval is neighbourhood of each point except end points.
real numbers is NBD of each real number
Rational numbers set is not the neighbourhood of any of its points.
Metric space | Distance Function | Example
Metric space : Definition and Axioms
Real Analysis : Introduction and Intervals
Union of countable sets is countable
Finite,infinite,equivalent,denumerable,countable sets
Infinite subset of countable set is countable
Field,Ordered Field,complete Ordered Field
Set of Integers is Countable
Supremum and infimum
Set is countably infinite iff it can be written in the form distinct elements
Continuum Hypothesis
Cartesian product of two countable sets is Countable
Set of Rational numbers is Countable
Keep Watching
OMG Maths
Classes by Cheena Banga
Definition of metric Space
Examples of metric space
Open and Closed sets
Topology and convergence
Types of metric spaces
Complete Spaces
Bounded and complete bounded spaces
Compact spaces
Locally compact and proper spaces
connectedness
Separable spaces
Pointed Metric spaces
Types of maps between metric spaces
continuous maps
uniformly continuous maps
Lipschitz-continuous maps and contractions
isometries
Quasi-isometries
notions of metric space equivalence
Topological properties
Distance between points and sets
Hausdorff distance and Gromov metric
Product metric spaces
Continuity of distance
Quotient metric spaces
Generalizations of metric spaces
Metric spaces as enriched categories
Pdf link:
****The Riemann-Stieltjes Integral****
****Real Analysis playlist****
useful for Msc | BSC | NET | NBHM | LPU | DU | IIT JAM | TIFR
Other topics covered in playlist:
The Riemann-Stieltjes Integral
The R-S Integral
Limit and Continuity
Limit Inferior and limit superior definition and theorems
Cauchy Sequence in Compact Metric Space is Convergent
nth tail of sequence
Tail of a sequence
P Series Test for convergence of series
Limit Comparison Test for Convergence
Cantor Intersection Theorem
E is a subset of metric space X then Diameter of closure of E is equal to Diameter of E
Cauchy First theorem on limits
Bolzano Weierstrass Theorem
Every bounded sequence has a convergent sub sequence
Every Cauchy sequence is a Bounded sequence
Every convergent Sequence is cauchy sequence
Cauchy Sequence
Cauchy Sequence Definition
Cauchy Sequence theorems
Sub sequence of a sequence
Algebraic Properties of Limits
Algebra of limit of sequence
Properties of limit
limit laws of sequence
sandwich theorem
squeeze theorem
Sequence and series
real sequence
range of sequence
constant sequence
uniqueness theorem
Sequences in metric space
limit of sequence
Convergent sequence
Every connected subset of R is an interval
The Real line R is connected
Every interval is connected
In R, intervals and only intervals are connected.
A subset E of R is connected iff E is an interval
compactness in Real Analysis
Connectedness in Real Analysis
Compactness in topology
Connectedness in topology
compactness
connectedness
theorems of compactness
theorems of connectedness
Heine-Borel theorem
Closed Set | definition | theorems
set is closed iff its complement is open
Bolzano weierstrass theorem : Every infinite bounded subset of R has a limit point.
Definition of Neighbourhood of a point
Definition of Open set
infinite intersection of open sets need not to be open
Union of two NBDS is NBD
Intersection of NBDS is NBD
Superset of a NBD is also a NBD
Every Open interval (a,b) is neighbourhood of each of its points.
Closed interval is neighbourhood of each point except end points.
real numbers is NBD of each real number
Rational numbers set is not the neighbourhood of any of its points.
Metric space | Distance Function | Example
Metric space : Definition and Axioms
Real Analysis : Introduction and Intervals
Union of countable sets is countable
Finite,infinite,equivalent,denumerable,countable sets
Infinite subset of countable set is countable
Field,Ordered Field,complete Ordered Field
Set of Integers is Countable
Supremum and infimum
Set is countably infinite iff it can be written in the form distinct elements
Continuum Hypothesis
Cartesian product of two countable sets is Countable
Set of Rational numbers is Countable
Keep Watching
OMG Maths
Classes by Cheena Banga
Definition of metric Space
Examples of metric space
Open and Closed sets
Topology and convergence
Types of metric spaces
Complete Spaces
Bounded and complete bounded spaces
Compact spaces
Locally compact and proper spaces
connectedness
Separable spaces
Pointed Metric spaces
Types of maps between metric spaces
continuous maps
uniformly continuous maps
Lipschitz-continuous maps and contractions
isometries
Quasi-isometries
notions of metric space equivalence
Topological properties
Distance between points and sets
Hausdorff distance and Gromov metric
Product metric spaces
Continuity of distance
Quotient metric spaces
Generalizations of metric spaces
Metric spaces as enriched categories
Комментарии
 0:31:53
0:31:53
 0:02:25
0:02:25
 0:13:35
0:13:35
 0:06:52
0:06:52
 0:16:24
0:16:24
 0:11:40
0:11:40
 0:09:11
0:09:11
 0:16:43
0:16:43
 0:10:57
0:10:57
 0:10:39
0:10:39
 0:07:35
0:07:35
 0:15:31
0:15:31
 0:26:11
0:26:11
 0:23:21
0:23:21
 0:18:33
0:18:33
 0:06:25
0:06:25
 0:14:39
0:14:39
 0:16:22
0:16:22
 1:00:04
1:00:04
 0:12:06
0:12:06
 0:04:52
0:04:52
 0:17:38
0:17:38
 0:51:23
0:51:23
 0:00:59
0:00:59