Kruskal-Wallis-Test - calculate required sample size with G*Power

// Kruskal-Wallis-Test - calculate required sample size with G*Power //

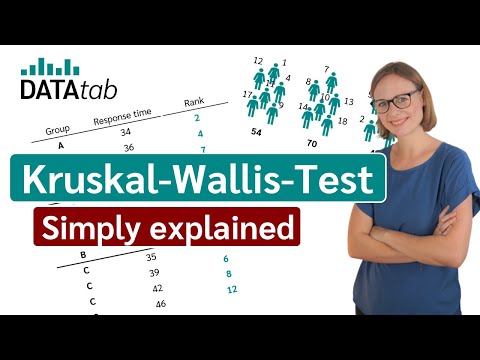
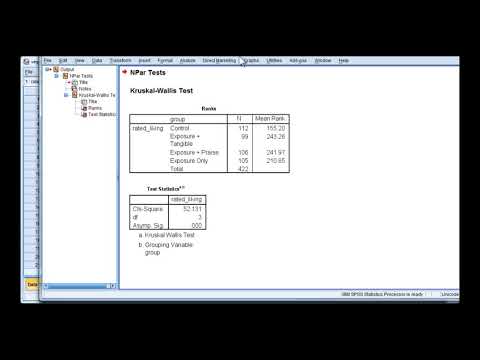
The Kruskal-Wallis-test is a non-parametric statistical method that is used in place of the one-way ANOVA when the data is not normally distributed. This test is used to assess whether the median of at least three groups is different.

In the run-up to an empirical study or data collection for the Kruskal-Wallis-test, the necessary sample size must be determined, for example using G*Power. The minimum sample size depends on the assumed effect size (f or Eta²), the alpha level, the statistical power and the number of groups.

At the end, I show examples of different minumum sample size for the Kruskal-Wallis-test with different characteristics of the input parameters mentioned using G*Power

Download link:
============

Introduction to G*Power:
====================

⏰ Timestamps:
==============
0:00 Introduction
0:10 Selecting the Kruskal-Wallis-test
0:23 Select type of power analysis
0:31 Input parameter I: Effect size f
1:05 Input parameter II: Alpha error probability
1:21 Input parameter III: power (1-beta error)
1:48 Input parameter IV: number of groups
1:59 Calculation and overview

If you have any questions or suggestions regarding Kruskal-Wallis-test - calculate required sample size with G*Power, please use the comment function. Thumbs up or down to decide if you found the video helpful.

#useR #statorials

Support channel? 🙌🏼
===================

[jessiemae]

Great! Thanks for being so easy to follow and getting straight to the point. Also appreciate the references (eg. Cohen 1992).

[GM__user]

Hello, thanks for the video! Just a question, how does this differ from G*Power's one-way ANOVA? Or in other words, what makes this calculation a Kruskal-Wallis test per se?