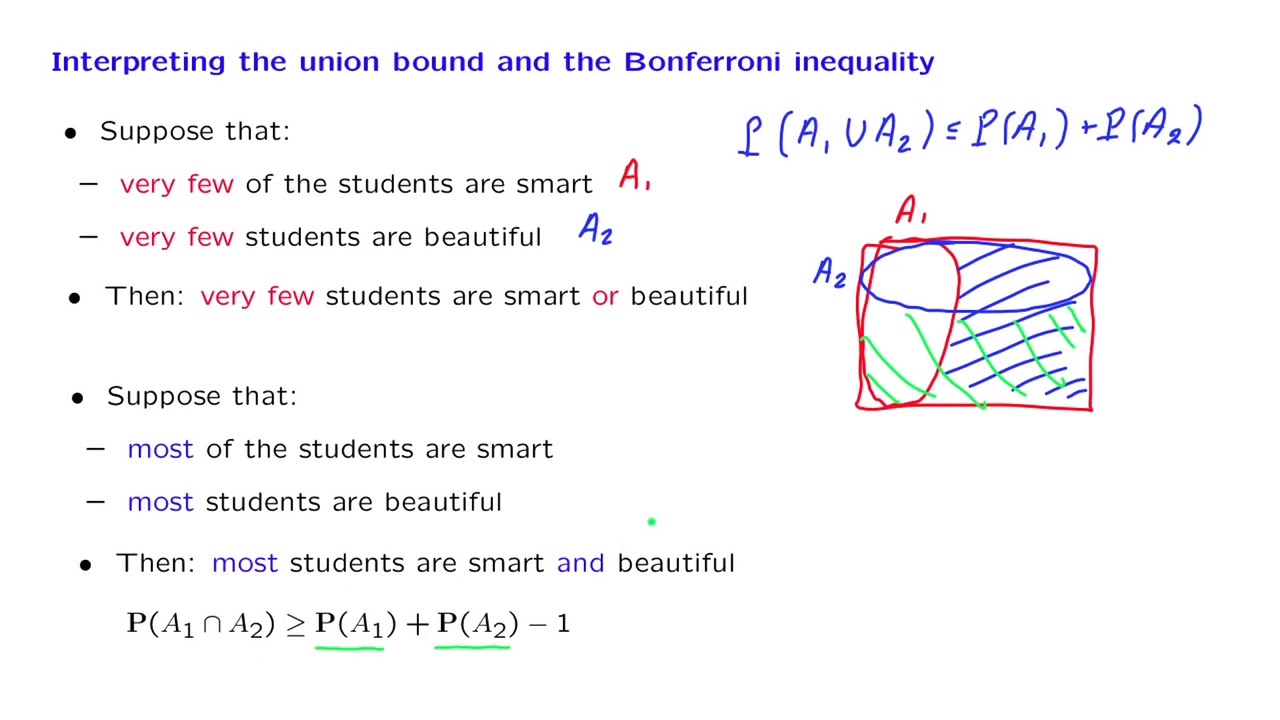
S01.10 Bonferroni's Inequality

MIT RES.6-012 Introduction to Probability, Spring 2018
Instructor: John Tsitsiklis

License: Creative Commons BY-NC-SA

[basedworldsk]

Essentially: if you have big sets with big probability then the intersection of those sets will also be big

[srsa]

Text: "Very few of the students are smart."
Professor: *Proceeds to draw ~1/3 of the set as smart*

[muhammadfaizanalibutt]

AT 4:09 the probability of A1 and A2 can be 1 or less than 1. If both are less than 1 and their sum cannot be grater than 1 because probability cannot be greater than 1. If we subtract 1 from the summation of P(A1) and P(A2) then we will be get a zero or a negative number and probability cannnot be negative.

[thepromptman]

Thank you for this video! It really helped me wrap my mind around Bonferroni's Inequality :)

[crestz]

This slide is missing from the lecture notes

[DonKarelove]

3:07, the argument that "most students are smart, most students are beautiful, and therefore, most students are smart and beautiful" is not convincing.

Suppose we have a group of 20 students. 11 of them (which is majority, the most) are smart, and among 11 smart students only 2 are titled as beautiful. Similarly, 11 students are beautiful, and, as we already established, only 2 of them hold the title of being smart. Here we have a contradiction, the most (interpreted as majority) of the students are smart, the most are beautiful, but very few (2 out of 20) is smart and beautiful.

[aetheruszhou]

I can't understand why P(A) + P(B) is small when both P(A) and P(B) are small.

[SecretEscapist]

Thanks a lot for this amazing lecture ☺

[VaibhavExploresTheWorld]

Suppose there is a universe of 100 people. 90 of those people have a car. 80 of those in the universe also have a bike. Suppose those who have both car and bike are 60 people. It seems that this situation violates the Bonferroni inequality since P(A∩B) here is 0.6 and P(A)+P(B)−1 is 0.7. But, 0.6 is not greater than equal to 0.7. What am I missing? Can any one help?!

[赵于-tu]

Thank you, this video is very useful.

[manastripathi]

What happens if A1 and A2 are disjoint sets ?

[raqueljardim]

I don't understand. Isn't P(A1 U A2) = P(A1) + P(A2)? The statement the former is less than or equal to the latter doesn't make any sence to me.

[duckymomo]

We didn’t go over this in the other probability course?

[standardcoder]

Some people might wanna argue that set A1 are boys and set A2 are girls, but not us... not us

[unrealengine]

Its does not look like a valid proof;

[daSurrealist]

An example of categorizing smart and beautiful is not a good idea lol. But got the concept.