filmov
tv
Electrical Engineering: Ch 16: Laplace Transform (16 of 58) The Residue Method

Показать описание
In this video I will explain and give an example of “the residue method” for finding the inverse of the Laplace transform.
Next video in this series can be seen at:
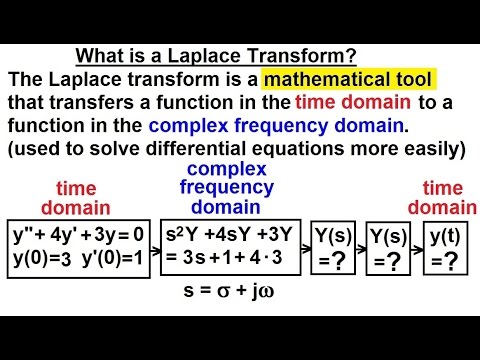
Electrical Engineering: Ch 16: Laplace Transform (1 of 58) What is a Laplace Transform?
Electrical Engineering: Ch 16: Laplace Transform (36 of 58) Find the Laplace Transform
Electrical Engineering: Ch 16: Laplace Transform (11 of 58) The Laplace Transform Table
Electrical Engineering: Ch 16: Laplace Transform (40 of 58) Laplace Transform of the Integral
Electrical Engineering: Ch 16: Laplace Transform (2 of 58) What is a Laplace Transform? Math Def
Electrical Engineering: Ch 16: Laplace Transform (3 of 58) The Laplace Transform of f(t)=t
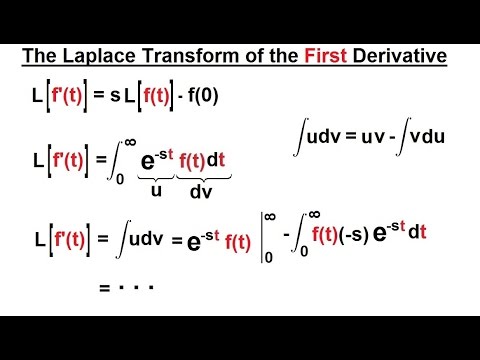
Electrical Engineering: Ch 16: Laplace Transform (20 of 58) Laplace Transform of the 1st Derivative
Electrical Engineering: Ch 16: Laplace Transform (38 of 58) Response to an Undamped System 1
Electrical Engineering: Ch 16: Laplace Transform (4 of 58) The Laplace Transform of f(t)=e^(at)
Electrical Engineering: Ch 16: Laplace Transform (22 of 58) Laplace Transf of the 2nd Derivative
Electrical Engineering: Ch 16: Laplace Transform (23 of 58) Laplace Transf of the 3rd Derivative
Electrical Engineering: Ch 16: Laplace Transform (17 of 58) Linear Properties of the Laplace Transf
Electrical Engineering: Ch 16: Laplace Transform (53 of 58) Laplace Transform of Periodic Fct.
Electrical Engineering: Ch 16: Laplace Transform (50 of 58) What is Convolution? Example 3
Electrical Engineering: Ch 16: Laplace Transform (55 of 58) Laplace Transform of Periodic Fct. Sum.
Electrical Engineering: Ch 16: Laplace Transform (12 of 58) The Inverse of the Laplace Transform
Electrical Engineering: Ch 16: Laplace Transform (57 of 58) Convolution Example
Electrical Engineering: Ch 16: Laplace Transform (54 of 58) Laplace Transform of Periodic Function
Electrical Engineering: Ch 16: Laplace Transform (48 of 58) What is Convolution? Def. 2: Graph 2
Electrical Engineering: Ch 16: Laplace Transform (14 of 58) The Inverse[Laplace Transf] Strategy 2
Electrical Engineering: Ch 16: Laplace Transform (9 of 53) s-Domain Equivalent of a Capacitor
Electrical Engineering: Ch 16: Laplace Transform (31 of 58) Solving Differential Equation Ex. 2
Electrical Engineering: Ch 16: Laplace Transform (46 of 58) What is Convolution? Def. 1: Ex.
Electrical Engineering: Ch 16: Laplace Transform (21 of 58) Laplace Transf of the 1st Derivative: 1
Комментарии
 0:06:02
0:06:02
 0:04:31
0:04:31
 0:04:31
0:04:31
 0:02:59
0:02:59
 0:05:42
0:05:42
 0:04:40
0:04:40
 0:04:34
0:04:34
 0:08:18
0:08:18
 0:02:55
0:02:55
 0:02:18
0:02:18
 0:03:03
0:03:03
 0:01:41
0:01:41
 0:04:04
0:04:04
 0:06:21
0:06:21
 0:03:39
0:03:39
 0:02:54
0:02:54
 0:03:17
0:03:17
 0:03:57
0:03:57
 0:06:19
0:06:19
 0:05:10
0:05:10
 0:06:00
0:06:00
 0:04:42
0:04:42
 0:06:09
0:06:09
 0:03:03
0:03:03