filmov
tv
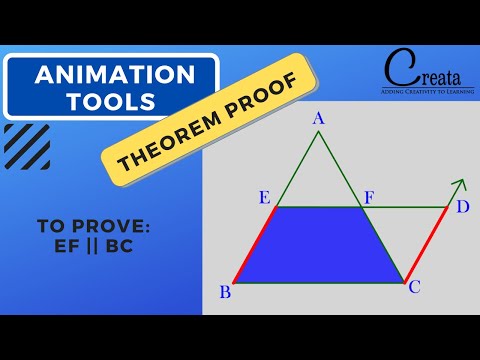
The line segment joining the midpoints of two sides of a Triangle is parallel to the third side.
Показать описание
Understand the proof of the theorem "The line segment joining the midpoints of two sides of a triangle is parallel to the third side."
We BELIEVE, that each and every student is unique in themself and has the inbuilt capability and capacity to learn anything in this world. Only the hindrance is the traditional teaching methods.
We at CREATA CLASSES enhance student comprehension through our line of instruction materials incorporating ANIMATION & visual tools, so they can achieve academic success and have a brighter and elevated future.
Regards
CREATA CLASSES
Adding Creativity to Learning.
We BELIEVE, that each and every student is unique in themself and has the inbuilt capability and capacity to learn anything in this world. Only the hindrance is the traditional teaching methods.
We at CREATA CLASSES enhance student comprehension through our line of instruction materials incorporating ANIMATION & visual tools, so they can achieve academic success and have a brighter and elevated future.
Regards
CREATA CLASSES
Adding Creativity to Learning.
0:07:10
The midpoint P of the line segment joining the points A(−10,4) and B(−2,0) lies on the line segment...
0:03:57
The line segment joining the midpoints of two sides of a Triangle is parallel to the third side.
0:04:46
Point P divides the line segment joining the points A (2,1) and B (5,-8) such that AP/AB=1/3.
0:05:50
Find the ratio in which the line segment joining the points (-3 10) and (6 -8) is divided by (-1 6)
0:05:55
the line segment joining the points (3 -4) and (1 2) is trisected at points p and q if coordinates
0:04:03
The line segment joining the points A(2, 1) and B(5, -8) is trisected at the points P and Q such
0:03:14
If the mid-point of the line segment joining the points A(3, 4) and B(k, 6) is P (x, y) and x+y-10=0
0:04:48
Find the ratio in which the y-axis divides the line segment joining the points (5 -6) and (-1 -4)
0:03:20
Points A, B, C and D divide the line segment joining the point (5,-10) and the origin in five e...
0:12:15
Calculate the coordinates of the midpoint of the line segment joining the pair of points.
0:04:02
The line segment joining the points A (2, 1) and B (5, -8) is trisected by the points P and Q
0:02:40
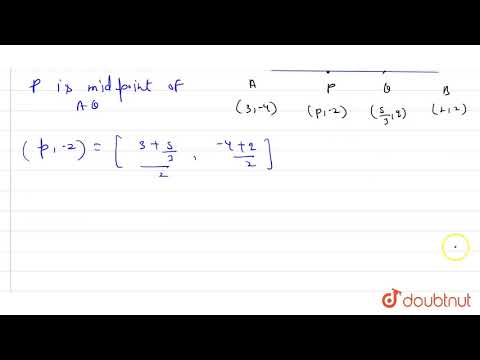
The line segment joining the points A(3, -4) and B(1, 2) is trisected at the points P(p, -2)
0:05:00
The line segment joining the points A(2, 1) and B(5, -8) is trisected at the points P and Q such tha
0:06:38
Find the coordinates of a point P on the line segment joining A(1 2) B(6 7) such that AP = 2/5AB
0:10:30
In what ratio is the line segment joining the points (-2,-3) and (3,7) divided by the y-axis? Also f
0:01:00
Find the coordinates of the mid-point of the line segment joining the points (6,3) and(4,7).
0:03:43
in what ratio is the line segment joining the points (–1, 1) and (5, 7) divided line x + y = 4
0:00:58
Find the midpoint of the line segment joining the points (3,-6) and (-1, -2)
0:01:32
The line segment joining the points `(3, -4)` and `(1, -2)` is divided by `Y-`axis in the ratio
0:03:26
Find the coordinates of the point which divides the line segment joining the points #sslcexam-23
0:02:01
The perpendicular bisector of the line segment joining the points A (1,5) and B (4,6) cuts y-axis
0:03:21
The line segment joining the points A (3, 2) and B (5,1) is divided at the point P in the ratio 1:2
0:05:13
Find the ratio in which the line segment joining A(1, -5) and B(-4, 5) is divided by the x-axis
0:04:03
4. Find the ratio in which the line segment joining the points(-3, 10) and (6, -8) is divided by (
Комментарии