filmov
tv
Graph Quadratic Function f(x)=-(x-2)^2: Find Vertex, Axis of Symmetry, Intercepts, Domain, Range

Показать описание
The given quadratic function is f(x)=-(x-2)^2 and it is compared to y=a(x-h)^2+k . (a) As the coefficient of the (x-h)^2 term is negative this parabola opens down. (b) The function y=a(x-h)^2+k has the vertex at (h,k) and this is used to find the vertex of the given function. (c) The axis of symmetry is the vertical line that passes through the vertex. (d) The y-intercept is found by setting x=0. (e) The x-intercepts are found by setting f(x)=0 and solving the resulting quadratic equation. (f) Additional points are calculated and then all this information is used to sketch the parabola. The domain and the range are then found from the graph.
Timestamps:
0:00 Introduction
1:03 Does Parabola Open Up or Down
1:27 Find Vertex
1:58 Axis of Symmetry
2:18 Find y-intercept
3:00 Find x-intercepts
4:15 Sketch Graph
6:36 Domain & Range
Timestamps:
0:00 Introduction
1:03 Does Parabola Open Up or Down
1:27 Find Vertex
1:58 Axis of Symmetry
2:18 Find y-intercept
3:00 Find x-intercepts
4:15 Sketch Graph
6:36 Domain & Range
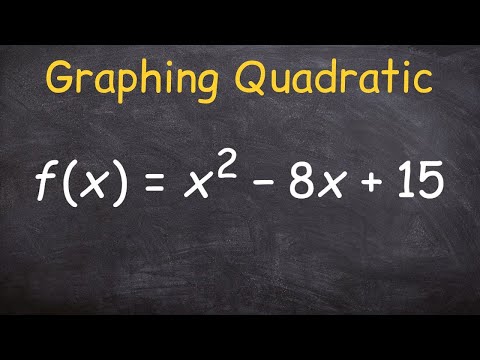 0:07:32
0:07:32
 0:07:36
0:07:36
 0:11:41
0:11:41
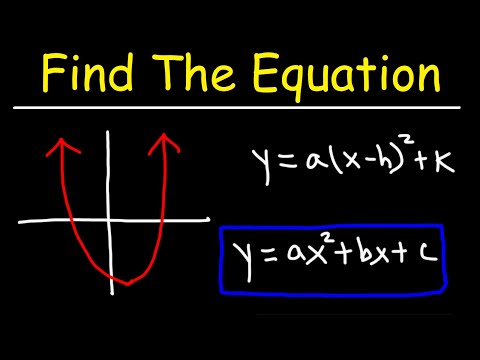 0:09:35
0:09:35
 0:18:49
0:18:49
 0:01:50
0:01:50
 0:01:33
0:01:33
 0:06:02
0:06:02
 1:48:58
1:48:58
 0:02:23
0:02:23
 0:09:29
0:09:29
 0:02:02
0:02:02
 0:15:59
0:15:59
 0:11:31
0:11:31
 0:01:56
0:01:56
 0:03:41
0:03:41
 0:04:53
0:04:53
 0:04:34
0:04:34
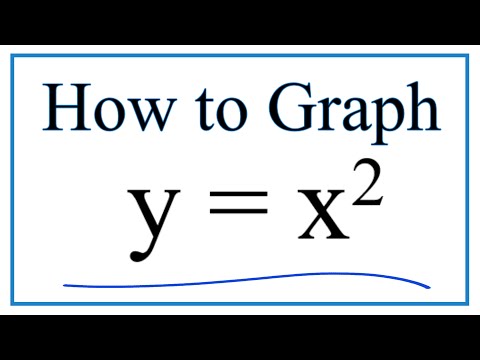 0:02:52
0:02:52
 0:07:46
0:07:46
 0:13:34
0:13:34
 0:02:26
0:02:26
 0:00:40
0:00:40
 0:02:48
0:02:48