filmov
tv
4]A Brief Explanation on Symmetric and Skew Symmetric Matrix with examples | Matrix Algebra
Показать описание
Symmetric matrix: Any square matrix is symmetric matrix if it is equal to its transpose.
Properties of Symmetric matrix are:
Sum is symmetric
Difference is symmetric
If A and B commute, product AB is symmetric
If A and B anti-commute, product AB is not symmetric
Skew-Symmetric matrix: Any square matrix is skew-symmetric matrix if it is equal to negative transpose.
Properties of Skew-Symmetric matrix are same as that of Symmetric matrix
Above matrices are explained with examples.
For latest updates subscribe our channel “YSR EduTech” or join me on Facebook page "YSR EduTech"
Properties of Symmetric matrix are:
Sum is symmetric
Difference is symmetric
If A and B commute, product AB is symmetric
If A and B anti-commute, product AB is not symmetric
Skew-Symmetric matrix: Any square matrix is skew-symmetric matrix if it is equal to negative transpose.
Properties of Skew-Symmetric matrix are same as that of Symmetric matrix
Above matrices are explained with examples.
For latest updates subscribe our channel “YSR EduTech” or join me on Facebook page "YSR EduTech"
![4]A Brief Explanation](https://i.ytimg.com/vi/0C_fWHKLhkM/hqdefault.jpg)
0:03:36
4]A Brief Explanation on Symmetric and Skew Symmetric Matrix with examples | Matrix Algebra
0:04:40
Asymmetric Encryption - Simply explained
0:05:30
Symmetric Groups (Abstract Algebra)
0:02:55
How to Identify and Calculate Rotational Symmetry
0:05:56
What is Symmetry? - Basics | Line of Symmetry | Don't Memorise
0:01:32
Math Shorts Episode 4 - Reflection
0:04:08
Simple Explanation of Conjugation in the Symmetric Group
0:01:45
What is Symmetric Encryption? Simply Explained | Venafi Chalk Talk
1:13:27
Lecture 17: Convergence of the Simple Symmetric-Cycle
0:04:18
Symmetric vs. Asymmetric Encryption - CompTIA Security+ SY0-401: 6.1
0:06:31
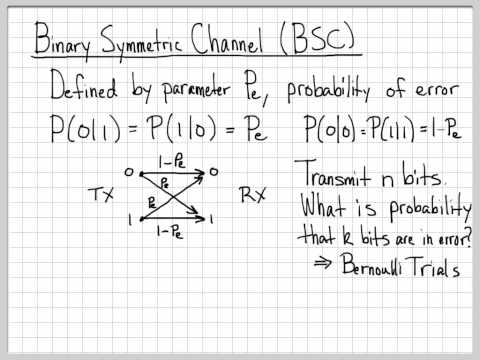
Fundamentals of Probability Theory (4/12): Binary Symmetric Channel
0:06:01
Reflectional Symmetry and Rotational Symmetry | Don't Memorise
0:01:22
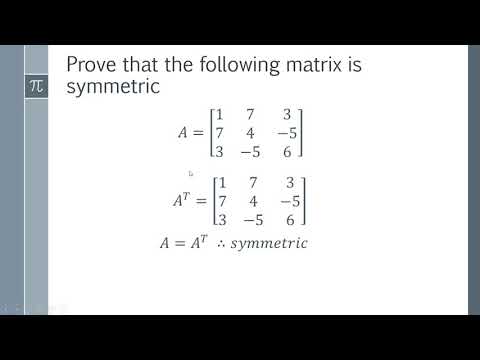
How to Prove a Matrix is Symmetric
0:03:09
Symmetry Song for Kids | A Day at Symmetry Land | Lines of Symmetry
0:31:09
Symmetric polynomials: definition and examples
0:04:25
What is a Skew-Symmetric Matrix?
0:10:41
Symmetric Key Cryptography | Stream Cipher & Block Cipher Explained | Network Security | Simplil...
0:00:49
Inverse of permutation #short video # group theory |symmetric group|B.sc math |M.sc maths
0:21:58
Group theory, abstraction, and the 196,883-dimensional monster
0:05:25
Class 12th – Symmetric & Skew Symmetric Matrix Problem-4 | Matrices | Tutorials Point
0:12:03
How to Find The Axis of Symmetry
0:05:45
What does a ≡ b (mod n) mean? Basic Modular Arithmetic, Congruence
0:07:25
How to find Centroid of an I - Section | Problem 1 |
0:12:13
Theorems on Symmetric and Hermitian Matrices
Комментарии