filmov
tv
Complex Multiplication in terms of Moduli and Arguments. Use Mathematica to illustrate.
Показать описание
Complex Analysis, Video #8 (Complex Arithmetic, Methods and Geometric Interpretations, Part 8). Complex Multiplication and its Geometric Interpretation. Intro to Modulus and Argument.
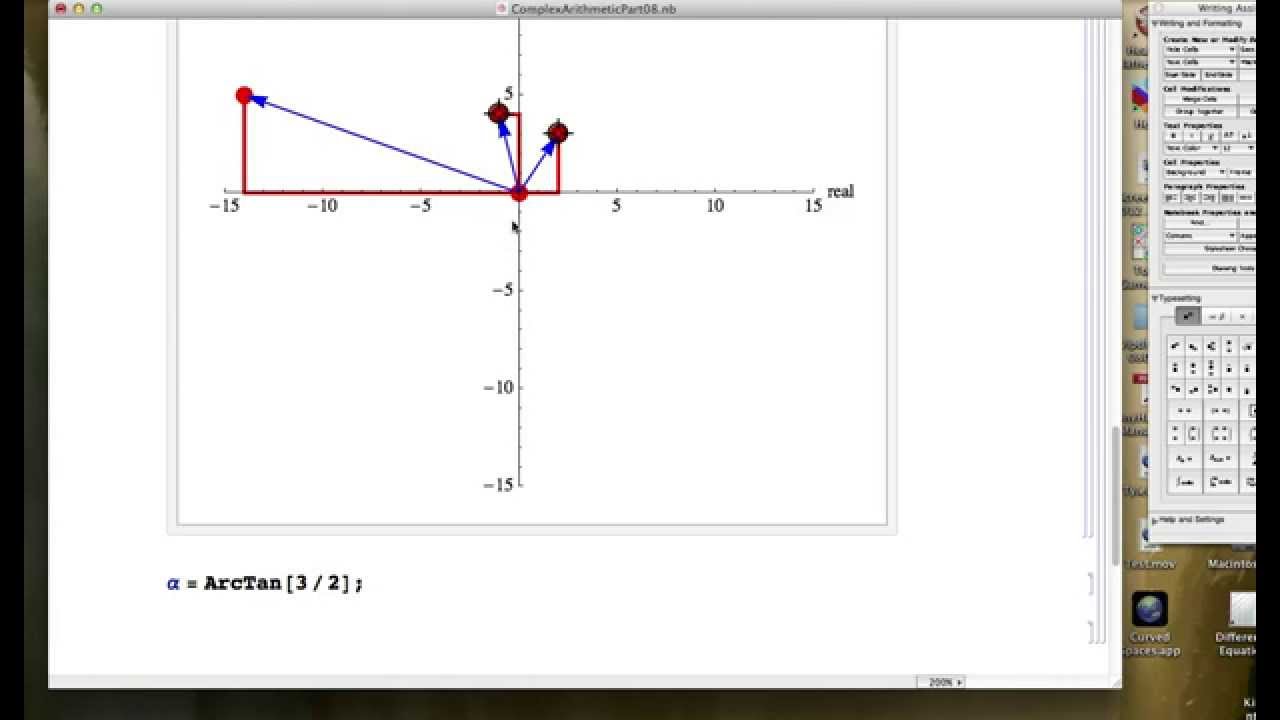
Details: Review complex multiplication. Review visualization using Locator on Mathematica. Verify that |z1*z2| = |z1|*|z2| using Abs on Mathematica (Abs[-14+5i] = Abs[2+3i]*Abs[-1+4i] since 13*17=221=196 + 25). The modulus of the product is the product of the modulii of the factors. The argument of a complex number. Verify that the argument of the product is the sum of the arguments of the factors by creating triangles and using trigonometry. Use the arctangent function on the triangles (via ArcTan on Mma) to confirm this.
Details: Review complex multiplication. Review visualization using Locator on Mathematica. Verify that |z1*z2| = |z1|*|z2| using Abs on Mathematica (Abs[-14+5i] = Abs[2+3i]*Abs[-1+4i] since 13*17=221=196 + 25). The modulus of the product is the product of the modulii of the factors. The argument of a complex number. Verify that the argument of the product is the sum of the arguments of the factors by creating triangles and using trigonometry. Use the arctangent function on the triangles (via ArcTan on Mma) to confirm this.
Complex Multiplication and Rotations
Complex Multiplication in Terms of Dot Product and Cross Product (with Geometrical Interpretation)
Complex Multiplication in terms of Moduli and Arguments. Use Mathematica to illustrate.
Benedict Gross “Complex Multiplication: Past, Present, Future” Lecture 2
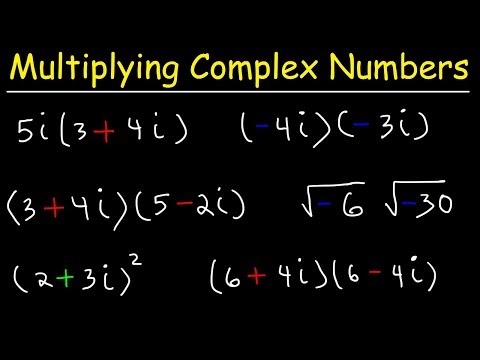
Multiplying Complex Numbers
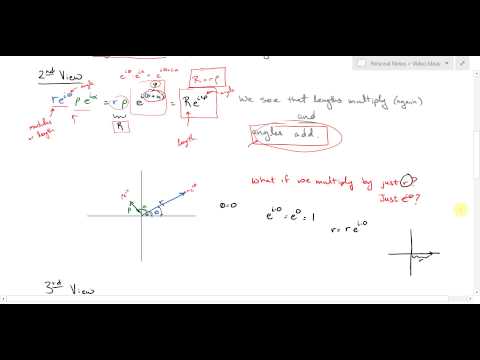
6 Three Views of Complex Multiplication
Advanced Complex Number Multiplication for Teens!
Jan Vonk : Complex multiplication - Lecture 1 -
Complex Multiplication General Case
Multiplication of Complex Numbers
Complex multiplication
Complex Multiplication Sqrt(3) + i
Ben Howard: Periods of Abelian Varieties with Complex Multiplication I
Complex Numbers IV: Complex Multiplication and Division
COMPLEX NUMBERS | How to Add, Subtract, Multiply and Divide Complex Numbers
Understanding Complex Multiplication...without Euler's Formula!
Videoconference: Complex multiplication for real quadratic fields and more
Complex Multiplication and Division 1080p
complex numbers multiplication – step by step
Benedict Gross “Complex Multiplication: Past, Present, Future” Lecture 3
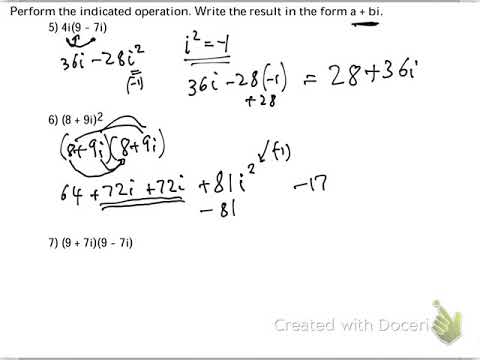
Multiplication of Complex Numbers
Why Complex Multiplication Produces Rotation
Algebra 80 - Multiplication with Complex Numbers
Complex Multiply Terms YR1
Комментарии
 0:20:29
0:20:29
 0:09:41
0:09:41
 0:10:04
0:10:04
 0:50:58
0:50:58
 0:06:29
0:06:29
 0:33:13
0:33:13
 0:05:45
0:05:45
 0:54:34
0:54:34
 0:04:38
0:04:38
 0:02:48
0:02:48
 0:02:16
0:02:16
 0:00:04
0:00:04
 0:47:58
0:47:58
 0:11:45
0:11:45
 0:14:58
0:14:58
 0:20:25
0:20:25
 1:12:38
1:12:38
 0:50:17
0:50:17
 0:13:57
0:13:57
 0:43:39
0:43:39
 0:01:48
0:01:48
 0:05:44
0:05:44
 0:18:55
0:18:55
 0:05:38
0:05:38