(NUMBER THEORY) Lecture 23: gcd(a,b)=ax+by

preview_player
Показать описание
I will teach you number theory and it's related theorem .... But before start the video you should know about the definition of number theory

Definition: Number theory is a branch of pure mathematics devoted to the study of the natural numbers and the integers. It is the study of the set of positive whole numbers which are usually called the set of natural numbers. ... Number Theory is partly experimental and partly theoretical.

Mathematics , number theory ,number theory theorems , divisibility , definition of divisibility , example of divisibility , learn mathematics , easy way to study mathematics
, Given integers a and b not both of which are zero there exist integers x and y such that 1=ax+by
  1. Khadija Shakeel
  2. Number theory lecture
  3. number theory bsc part 2
  4. number theory bsc
  5. number theory in discrete mathematics
  6. number theory bsc 3rd year
Рекомендации по теме
(NUMBER THEORY) Lecture 0:14:50

(NUMBER THEORY) Lecture 23: gcd(a,b)=ax+by

(NUMBER THEORY) Lecture 0:02:45

(NUMBER THEORY) Lecture 24: gcd (a,b) (multiple) = ax+by

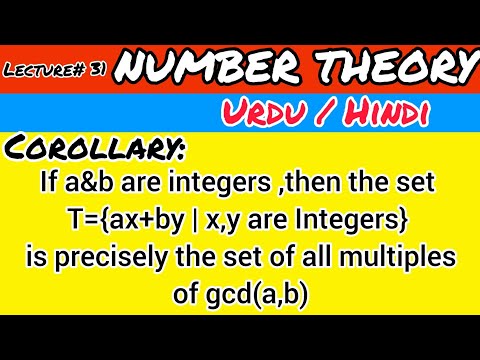
(NUMBER THEORY) Lecture 0:04:41

(NUMBER THEORY) Lecture 31: gcd(a,b) (multiple) =ax+by

NUMBER THEORY|| GCD(a,b)=ax+by 0:13:02

NUMBER THEORY|| GCD(a,b)=ax+by | Lecture- 12

Proof of GCD(a,b) 0:19:25

Proof of GCD(a,b) = ax+by The Diophantine Equation

Two Basic Theorems 0:08:12

Two Basic Theorems on gcd (Greatest Common Divisors) of Two Integers (Bezout's Identity)

Find integers x 0:11:22

Find integers x and y such that gcd (a, b) = a x + b y /gcd (a, b) as linear combinations of a and b

GCD and Bezout 0:06:08

GCD and Bezout Theorem | Math Olympiad, ISI, CMI Entrance | Number Theory

4. gcd(a,b) = 0:14:14

4. gcd(a,b) = ax+ by | GCD | Number Theory | Ravina Tutorial | in Hindi

Fundamentals of Number 0:06:43

Fundamentals of Number Theory - Greatest Common Divisor

S1 B.Sc; Number 0:17:55

S1 B.Sc; Number Theory; Lecture 23-Fibonacci Numbers & LDEs, Solution of LDE in more than2 varia...

Number Theory: vector 0:59:51

Number Theory: vector Euclidean Algorithm, survey of prime integers, 8-23-21 part 1

Number Theory Chapter 0:30:28

Number Theory Chapter 1Video 4

Bezout's Lemma | 0:25:00

Bezout's Lemma | Road to RSA Cryptography #2

A proof from 0:00:55

A proof from the Book on the infinite number of primes

Proof that GCD(a 0:20:54

Proof that GCD(a ,b) is minimum element is set S={ ax + by } ( Bezout's Identity Proof ).

linear diophantine equation 0:23:17

linear diophantine equation | L 4 | ax-by = c solvable iff GCd(a,b)|c | number theory

The GCD Divides 0:06:01

The GCD Divides All Common Divisors

Divisibility |Part 3| 0:13:05

Divisibility |Part 3| Greatest Common Divisor

Solving ax+by=gcd(a,b) 0:09:39

Solving ax+by=gcd(a,b)

S1 B.Sc; Number 0:21:04

S1 B.Sc; Number Theory; Lecture 11-Properties of gcd-Part 1

Algebra: Integers (part 0:20:26

Algebra: Integers (part 3) Euclidean algorithm

(NUMBER THEORY) Lecture 0:04:47

(NUMBER THEORY) Lecture 25: let a and b are relatively prime iff ax+by=1

[Deprecated] Number Theory 0:12:43

[Deprecated] Number Theory Primer Lecture 2 An Alternate Proof of Division with Remainder Theorem

Комментарии
Автор

Thanks a lot di for such wonderful lecture....all doubts of mine now cleared, 🙂🙂

harman