filmov
tv
AlgTop2: Homeomorphism and the group structure on a circle
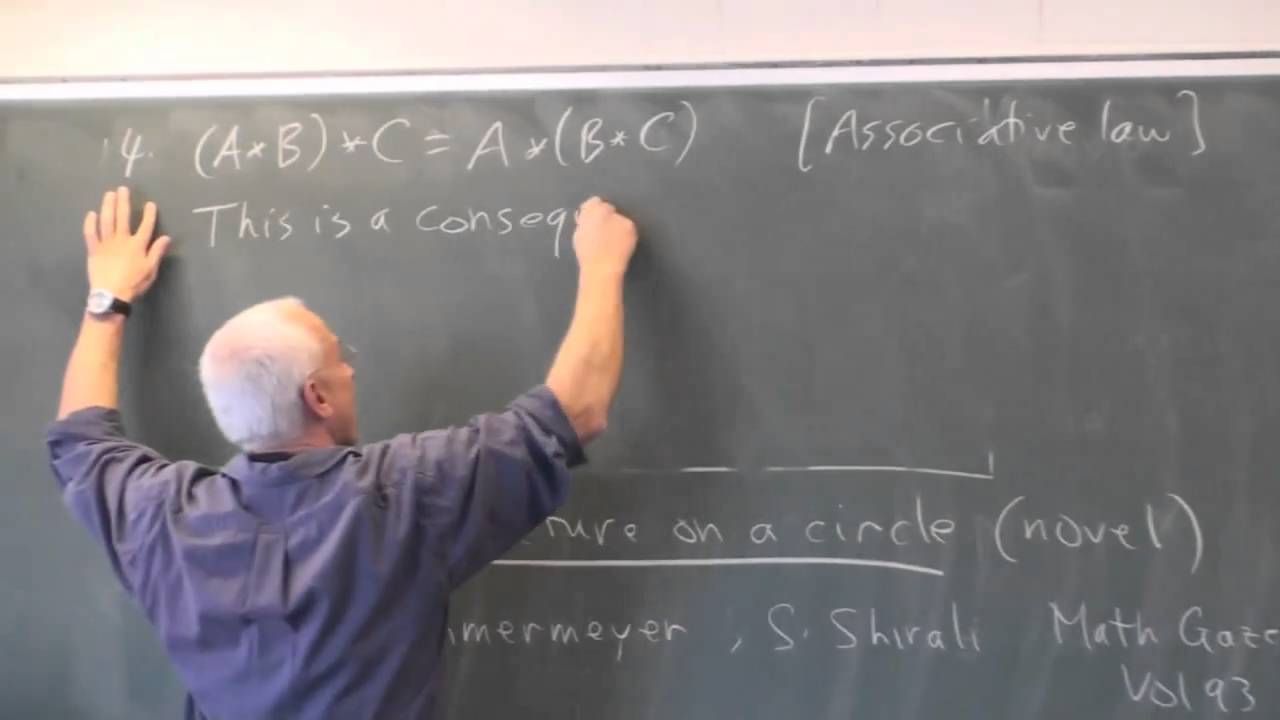
Показать описание
This is the first video of the second lecture in this beginner's course on Algebraic Topology. We give the basic definition of homeomorphism between two topological spaces, and explain why the line and circle are not homeomorphic.
Then we introduce the group structure on a circle, or in fact a general conic, in a novel way, following Lemmermeyer and as explained by S. Shirali.
This gives a gentle intro to the definition of a group. It also uses Pascal's theorem in an interesting way, so we give some background on projective geometry.
This lecture is part of a beginner's course in Algebraic Topology given by N J Wildberger at UNSW.
Then we introduce the group structure on a circle, or in fact a general conic, in a novel way, following Lemmermeyer and as explained by S. Shirali.
This gives a gentle intro to the definition of a group. It also uses Pascal's theorem in an interesting way, so we give some background on projective geometry.
This lecture is part of a beginner's course in Algebraic Topology given by N J Wildberger at UNSW.
AlgTop2: Homeomorphism and the group structure on a circle
Homeomorphism and the group structure on a circle | Algebraic Topology 2 | NJ Wildberger
Homeomorphism and Homotopy Equivalance
Homeomorphism Meaning
What does topological group mean?
Introduction to Topology. Fundamental Groups. Homeomorphisms
Topological group
Homeomorphism
Topological Homeomorphisms Part 1
Benjamin Vejnar - Complexity of the homeomorphism relation between compact spaces
Undergraduate Topology: March 2, quotienting by group of homeomorphisms (part 2)
Introduction to Algebraic Topology
Every continous bijection f from a compact metric space X to a metric space Y is a homeomorphism.
Undergraduate Topology: March 2, quotienting by group of homeomorphisms (part 1)
Undergraduate Topology: Feb 19, topological groups (part 1)
Homeomorphism
Homeomorphic Metric spaces | Analysis | BSc Analysis
What does homeomorphic mean?
Homeomorphism in topological spaces
C. Leininger - Teichmüller spaces and pseudo-Anosov homeomorphism (Part 2)
M-05. Homeomorphism
Examples of Homeomorphisms
Undergraduate Topology: Feb 19, topological groups (part 2)
Homeomorphic Meaning
Комментарии
 0:52:09
0:52:09
 0:52:09
0:52:09
 0:21:08
0:21:08
 0:00:35
0:00:35
 0:00:27
0:00:27
 0:10:06
0:10:06
 0:09:59
0:09:59
 0:05:52
0:05:52
 0:20:38
0:20:38
 1:29:59
1:29:59
 0:37:18
0:37:18
 0:20:01
0:20:01
 0:01:30
0:01:30
 0:59:51
0:59:51
 0:59:51
0:59:51
 0:02:52
0:02:52
 0:52:27
0:52:27
 0:00:33
0:00:33
 0:14:02
0:14:02
 1:08:39
1:08:39
 0:26:41
0:26:41
 0:23:36
0:23:36
 0:29:16
0:29:16
 0:00:25
0:00:25