filmov
tv
L6.2 Degenerate Perturbation Theory: Problem 6.6 Detailed Solution Part (a) 2/2
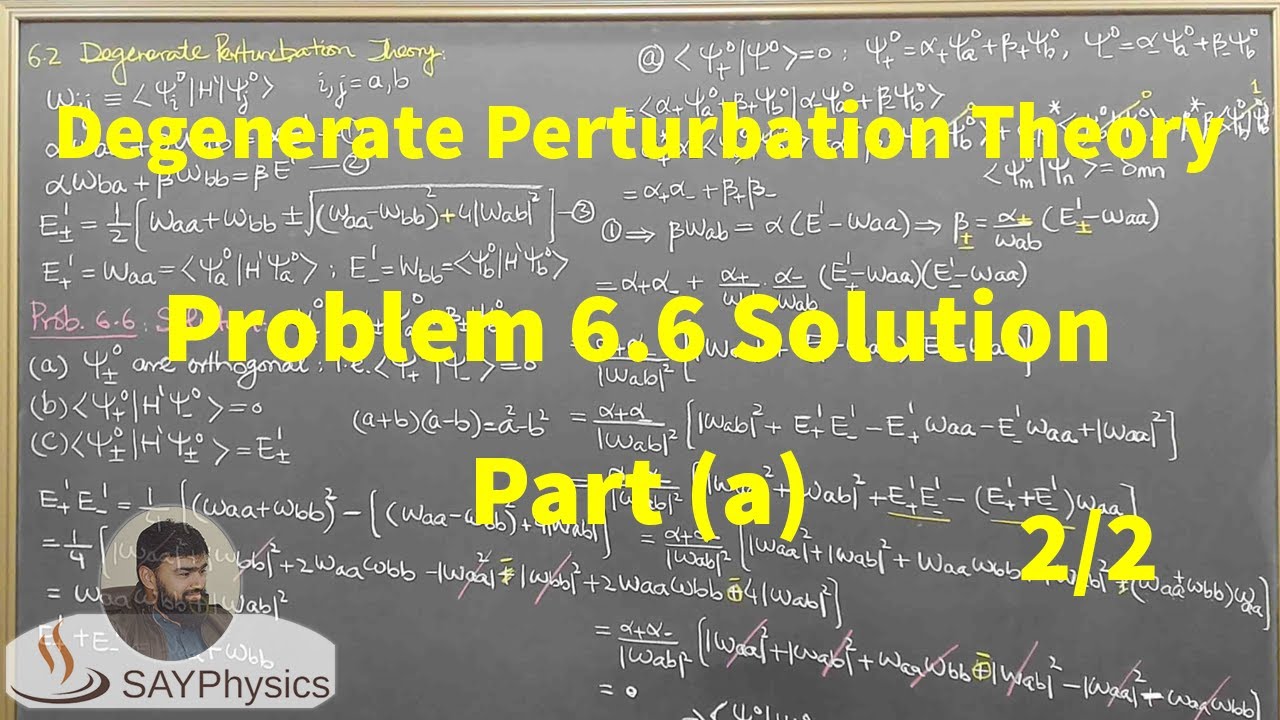
Показать описание
#QuantumMechanics #Physics #Griffiths #QuantumPhysics #ProblemSolving #PerturbationTheory #Orthogonality #PhysicsStudent #QuantumStates #EnergyShifts
Welcome to this detailed solution of Problem 6.6 from David J. Griffiths' Introduction to Quantum Mechanics (2nd Edition). In this video, we'll walk through the problem step by step, exploring the orthogonality of unperturbed states, matrix elements of the perturbation Hamiltonian, and energy shifts.
Problem Statement:
Let the two "good" unperturbed states be:
ψ₀₊ = α₊ψₐ₀ + β₊ψ_b₀,
where α₊ and β₊ are determined (up to normalization) by Equation 6.22 (or Equation 6.24). Show explicitly that:
(a) ψ₀₊ and ψ₀₋ are orthogonal (⟨ψ₀₊|ψ₀₋⟩ = 0);
(b) ⟨ψ₀₊|H'|ψ₀₋⟩ = 0;
(c) ⟨ψ₀₊|H'|ψ₀₊⟩ = E₊¹ with E₊¹ given by Equation 6.27.
Key Concepts Covered:
Quantum Superposition
Orthogonality of Quantum States
Perturbation Theory
Energy Shifts
If you found this video helpful, please give it a thumbs up, subscribe to the channel for more quantum mechanics tutorials, and leave a comment below with any questions or suggestions for future videos!
Welcome to this detailed solution of Problem 6.6 from David J. Griffiths' Introduction to Quantum Mechanics (2nd Edition). In this video, we'll walk through the problem step by step, exploring the orthogonality of unperturbed states, matrix elements of the perturbation Hamiltonian, and energy shifts.
Problem Statement:
Let the two "good" unperturbed states be:
ψ₀₊ = α₊ψₐ₀ + β₊ψ_b₀,
where α₊ and β₊ are determined (up to normalization) by Equation 6.22 (or Equation 6.24). Show explicitly that:
(a) ψ₀₊ and ψ₀₋ are orthogonal (⟨ψ₀₊|ψ₀₋⟩ = 0);
(b) ⟨ψ₀₊|H'|ψ₀₋⟩ = 0;
(c) ⟨ψ₀₊|H'|ψ₀₊⟩ = E₊¹ with E₊¹ given by Equation 6.27.
Key Concepts Covered:
Quantum Superposition
Orthogonality of Quantum States
Perturbation Theory
Energy Shifts
If you found this video helpful, please give it a thumbs up, subscribe to the channel for more quantum mechanics tutorials, and leave a comment below with any questions or suggestions for future videos!
0:15:36
L6.2 Degenerate Perturbation Theory: Problem 6.6 Detailed Solution Part (a) 2/2
0:15:24
L6.1 Degenerate Perturbation Theory: Problem 6.6 Detailed Solution Part (a) 1/2
0:22:56
L1.1 General problem. Non-degenerate perturbation theory
0:10:09
L6.2 Weak-field Zeeman effect; general structure
0:01:15
Phys3001 Example 20.1.2 Perturbation Theory
0:25:12
L6: Anharmonic Oscillator Part 3
0:49:54
Lecture - 3 | Perturbation Theory: Degenerate Case | Dalgarno-Lewis Method
0:00:16
Quantum mechanics perturbation theory wave function perturbed state II
0:46:43
Stark effect || Time Independent Perturbation Theory || Degenerate system
0:31:12
Perturbation Theory
0:22:28
L2.1 Remarks and validity of the perturbation series
0:17:39
L3.1 Remarks on a 'good basis'
0:13:07
L6.1 Zeeman effect and fine structure
0:26:12
Strong Field Zeeman effect derivation + Griffiths Intro to QM 6.20 (3rd edition) 6.22 (2nd edition)
0:04:24
The Linear Stark Effect | Quantum Mechanics
0:09:50
L6.4 Strong-field Zeeman
0:11:43
Delta Function Perturbation [Example]
0:06:27
L1.3 Calculating the energy corrections
0:44:36
JK-SET 2023 Non Degenerate Perturbation Quantum Mechanics
0:38:21
Time Independent Perturbation CSIR NET Physics Quantum
0:06:26
Non Degenerate Perturbation Theory For CSIR NET Physics | Quantum Mechanics | L1
0:18:45
L5: Anharmonic Oscillator Part 2
1:29:35
PH3203 - Lecture 6
0:05:14
Singular perturbation problem Ex.2//MM-II//M.Adnan Anwar
Комментарии