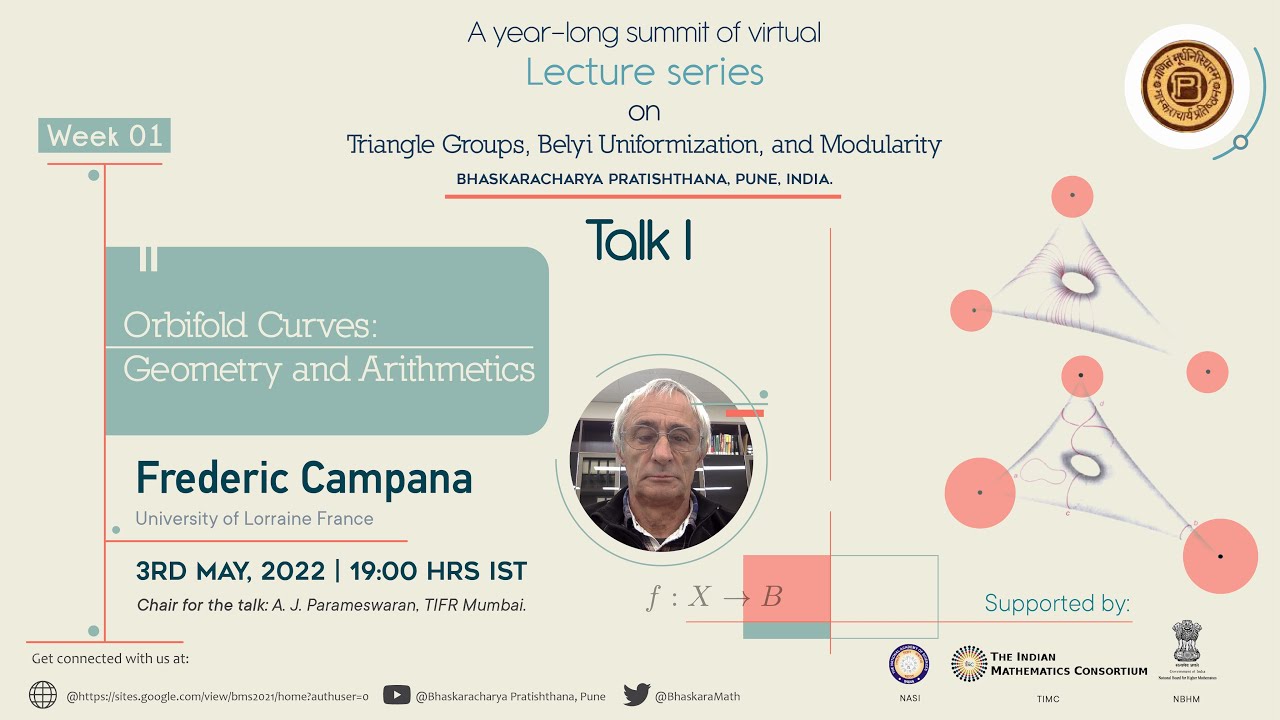
Orbifold Curves: Geometry and Arithmetics-Frederic Campana, University of Lorraine France.

Chair for the Talk: A. J. Parameswaran, TIFR Mumbai.

Abstract: Let $f:X\to B$ a 'fibration' between two complex projective connected manifolds. A fundamental problem of algebraic geometry consists in deriving the `qualitative geometry' of $X$ (as expressed by suitable invariants such as the fundamental group, the cohomology, or the Kodaira dimension) from the ones of the base $B$ and of $X_b$, the generic smooth fibre. Our objective here is to stress the r\^ole played by the multiple fibres of $f$, and especially the fact that the `classical' notion of multiplicity is not the appropriate one, in general. This geometric observation leads, among many other things, to a conjectural `orbifold' version of the Mordell/Lang `conjecture'. We restrict here to the technically much simpler case when $B$ is a curve, the ideas in the general case being similar.