8.06 Galois correspondence for covering spaces 2. Summary and examples

Показать описание

We bring together various things we have proved to state the Galois correspondence for covering spaces and illustrate this with some examples.

Jonathan Evans

Рекомендации по теме

Комментарии

Thank you very much for this video and for the whole playlist, which I have enjoyed a lot!

I liked that you ended the series by providing examples. In particular, you addressed the point I raised in a comment to some earlier video about the existence of a universal cover.

The universal cover of the figure 8 is exactly the example I tried to describe in that comment. I had wrongly named it “free space” (basically, I was looking at it as a free tree construction, if that makes sense). It’s kind of funny because the figure 8 is what corresponds to the free group on two generators. While its universal cover corresponds to the trivial group (which could also be seen as a free group, I guess, but without generators).

Back to the main point. At the end of the video, you hinted at the fact that there are spaces that don’t admit a universal cover. I thought about several options (using variants of the topologist’s sine curve, one of the weirdest spaces I know). But so far I haven’t been able to find a good counter-example. I will do some research then.

Thanks again!

lfossati

This is a great course and is being very useful to me in the study of algebraic topology, I would love to see a video on the construction of the universal cover

laflaca

8.06 Galois correspondence for covering spaces 2. Summary and examples

Algebraic Topology - Lecture 8 - Galois Correspondence

8.05 Galois correspondence for covering spaces 1. Covers from subgroups

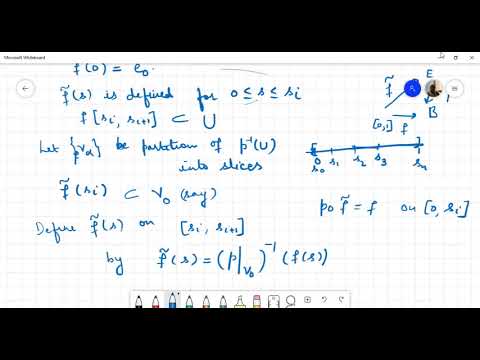
MATH 368 Galois Correspondence Examples

Galois Correspondence for Finite Fields

Abstract Algebra II: the Galois correspondence part 1, 2-12-18

FIT4.3.1. Galois Group of Order 8

GaloisTheoryI

Galois Group of Cyclotomic Polynomial

8. Universal Covers; Application to Free Groups - Pierre Albin

9. Classification of Covering Spaces; Deck Transformations - Pierre Albin

From representations of the symmetric group to branched covers of the disk - Amitai Netser Zernik

algebraic geometry and Galois theory 1

Algebraic Topology: Covering spaces, Lecture 4

Galois twisted forms and nonabelian H1

Covering spaces and 2-oriented graphs | Algebraic Topology | NJ Wildberger

A sketch of topological semi-Galois theory

Nathan Dunfield, Lecture 1: Fun with Finite Covers of 3-Manifolds

Fun with finite covers of 3-manifolds - Nathan Dunfield

Galois Extensions: Finding the Subgroups of the Galois Group

Egor Yasinsky (École Polytechnique)

Galois Groups of Cyclotomic Fields (Algebra 3: Lecture 25 Video 1)

Summary: an example covering ALL group theory concepts!! | Essence of Group Theory

Cyclotomic Extensions

Abstract Algebra II: Galois extensions, 2-14-18