filmov
tv
Integral of the Day 6.18.24 | Medium Spicy Level! What do you think? | Math with Professor V
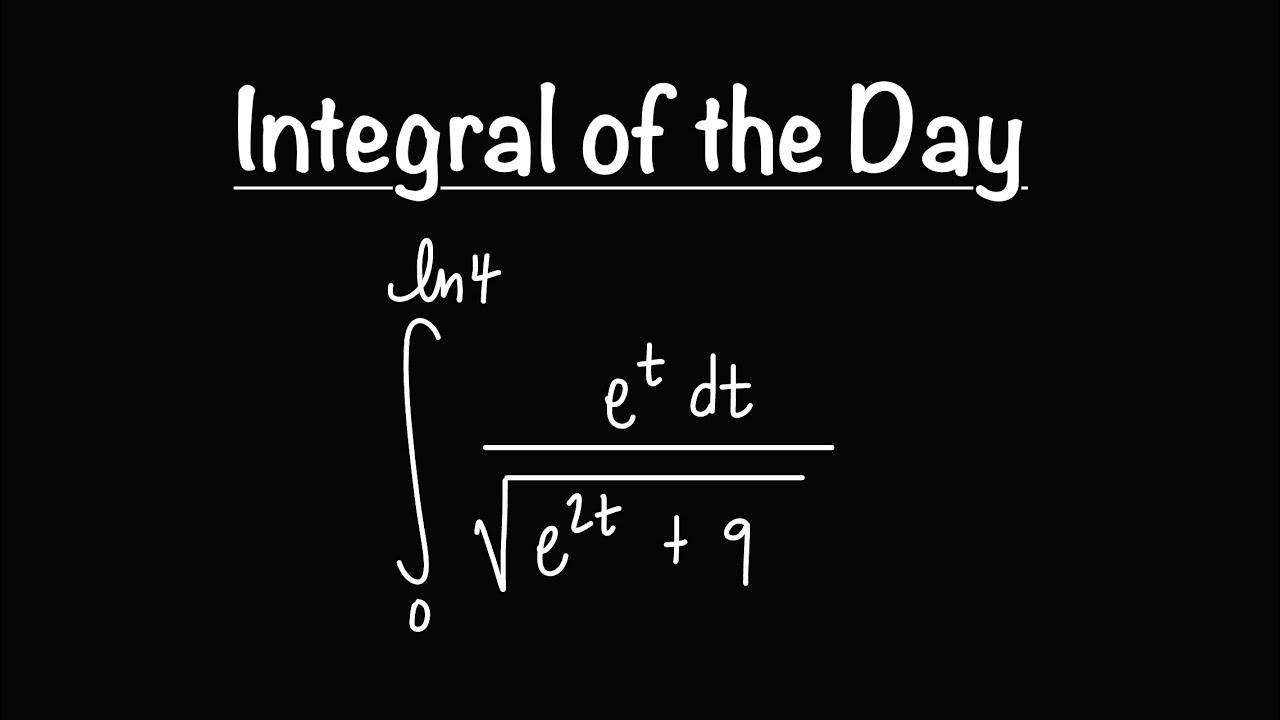
Показать описание
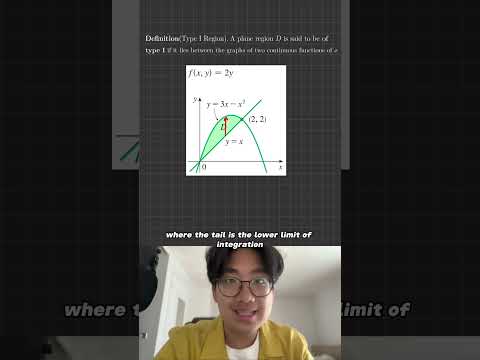
Here's your latest Integral of the Day! I promised a more straightforward integral after my most recent upload, so let me know if you were able to successfully solve it on your own. It did require some attention to detail with changing the limits of integration, especially with the trig sub portion, so if you need help with that check out the video below:
Don't forget to LIKE, Comment, & Subscribe!
xoxo,
Professor V
Calculus 2 Lecture Videos on Integration:
Trig Review:
Calculus 3 Video Lectures:
#mathwithprofessorv #integration #partialfractions #partialfractionmethod #partialfractiondecomposition #trigonometricsubstitution #trigintegrals #trigsubstitution #integrals #integralcalculus #calculus2 #calculusvideos #calculus2videos #integralvideos #trigonometricintegrals #math #youtubemath #mathvideos #mathtutor #mathprofessor #calculusvideos #integrationbyparts #integralcalculus
Join this channel to get access to perks:
Socials:
IG: @mathwithprofessorv
TikTok: @mathwithprofessorv
I'm also an Amazon influencer, so feel free to support and shop:
EXCITING NEWS: You can now sign up for my Patreon at the link below!
My Patreon is a place for students to have access to exclusive ad-free content not available on my YouTube channel! Access to the library of additional videos, worksheets, and more is available with the "Star Pupil Package" tier for just $9.99/month (USD).
*This video is copy protected and cannot be downloaded or used in any capacity without my permission.*
Don't forget to LIKE, Comment, & Subscribe!
xoxo,
Professor V
Calculus 2 Lecture Videos on Integration:
Trig Review:
Calculus 3 Video Lectures:
#mathwithprofessorv #integration #partialfractions #partialfractionmethod #partialfractiondecomposition #trigonometricsubstitution #trigintegrals #trigsubstitution #integrals #integralcalculus #calculus2 #calculusvideos #calculus2videos #integralvideos #trigonometricintegrals #math #youtubemath #mathvideos #mathtutor #mathprofessor #calculusvideos #integrationbyparts #integralcalculus
Join this channel to get access to perks:
Socials:
IG: @mathwithprofessorv
TikTok: @mathwithprofessorv
I'm also an Amazon influencer, so feel free to support and shop:
EXCITING NEWS: You can now sign up for my Patreon at the link below!
My Patreon is a place for students to have access to exclusive ad-free content not available on my YouTube channel! Access to the library of additional videos, worksheets, and more is available with the "Star Pupil Package" tier for just $9.99/month (USD).
*This video is copy protected and cannot be downloaded or used in any capacity without my permission.*
Комментарии
 0:06:07
0:06:07
 0:12:35
0:12:35
 0:00:28
0:00:28
 0:00:12
0:00:12
 0:00:30
0:00:30
 0:18:12
0:18:12
 0:09:15
0:09:15
 0:09:12
0:09:12
 9:29:27
9:29:27
 0:00:31
0:00:31
 0:05:36
0:05:36
 0:00:46
0:00:46
 0:00:13
0:00:13
 0:05:51
0:05:51
 0:01:00
0:01:00
 0:00:32
0:00:32
 0:18:30
0:18:30
 0:17:00
0:17:00
 0:00:36
0:00:36
 0:01:42
0:01:42
 0:00:26
0:00:26
 0:00:57
0:00:57
 0:09:18
0:09:18
 3:00:13
3:00:13